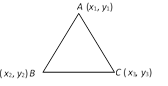
 \[\Delta =\frac{1}{2}\left| \begin{matrix} {{x}_{1}} & {{y}_{1}} & 1 \\ {{x}_{2}} & {{y}_{2}} & 1 \\ {{x}_{3}} & {{y}_{3}} & 1 \\ \end{matrix} \right|\]\[=\frac{1}{2}\left| \text{ }({{x}_{1}}({{y}_{2}}-{{y}_{3}})+{{x}_{2}}({{y}_{3}}-{{y}_{1}})+{{x}_{3}}({{y}_{1}}-{{y}_{2}})\text{ } \right|\]
In equilateral triangle
(i) Having sides a, area is \[\frac{\sqrt{3}}{4}{{a}^{2}}\].
(ii) Having length of perpendicular as 'p' area is \[\frac{({{p}^{2}})}{\sqrt{3}}\] .
(2) Collinear points : Three points \[A({{x}_{1}},{{y}_{1}}),\,\,B({{x}_{2}},{{y}_{2}}),\,C({{x}_{3}},{{y}_{3}})\] are collinear. If area of triangle is zero, then
(i) \[\Delta =0\] \[\Rightarrow \] \[\frac{1}{2}\left| \begin{matrix} {{x}_{1}} & {{y}_{1}} & 1 \\ {{x}_{2}} & {{y}_{2}} & 1 \\ {{x}_{3}} & {{y}_{3}} & 1 \\ \end{matrix} \right|=0\] \[\Rightarrow more...
\[\Delta =\frac{1}{2}\left| \begin{matrix} {{x}_{1}} & {{y}_{1}} & 1 \\ {{x}_{2}} & {{y}_{2}} & 1 \\ {{x}_{3}} & {{y}_{3}} & 1 \\ \end{matrix} \right|\]\[=\frac{1}{2}\left| \text{ }({{x}_{1}}({{y}_{2}}-{{y}_{3}})+{{x}_{2}}({{y}_{3}}-{{y}_{1}})+{{x}_{3}}({{y}_{1}}-{{y}_{2}})\text{ } \right|\]
In equilateral triangle
(i) Having sides a, area is \[\frac{\sqrt{3}}{4}{{a}^{2}}\].
(ii) Having length of perpendicular as 'p' area is \[\frac{({{p}^{2}})}{\sqrt{3}}\] .
(2) Collinear points : Three points \[A({{x}_{1}},{{y}_{1}}),\,\,B({{x}_{2}},{{y}_{2}}),\,C({{x}_{3}},{{y}_{3}})\] are collinear. If area of triangle is zero, then
(i) \[\Delta =0\] \[\Rightarrow \] \[\frac{1}{2}\left| \begin{matrix} {{x}_{1}} & {{y}_{1}} & 1 \\ {{x}_{2}} & {{y}_{2}} & 1 \\ {{x}_{3}} & {{y}_{3}} & 1 \\ \end{matrix} \right|=0\] \[\Rightarrow more...
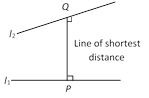 (2) Line of shortest distance : If \[{{l}_{1}}\] and \[{{l}_{2}}\] are two skew lines, then the straight line which is perpendicular to each of these two non-intersecting lines is called the “Line of shortest distance.”
There is one and only one line perpendicular to each of lines \[{{l}_{1}}\] and \[{{l}_{2}}\].
(3) Shortest distance between two skew lines
Let two skew lines be, \[\frac{x-{{x}_{1}}}{{{l}_{1}}}=\frac{y-{{y}_{1}}}{{{m}_{1}}}=\frac{z-{{z}_{1}}}{{{n}_{1}}}\] and \[\frac{x-{{x}_{2}}}{{{l}_{2}}}=\frac{y-{{y}_{2}}}{{{m}_{2}}}=\frac{z-{{z}_{2}}}{{{n}_{2}}}\]
Therefore, the shortest distance between the lines is given by \[d=\frac{\left| \,\begin{matrix} {{x}_{2}}-{{x}_{1}} & {{y}_{2}}-{{y}_{1}} & {{z}_{2}}-{{z}_{1}} \\ {{l}_{1}} & {{m}_{1}} & {{n}_{1}} \\ {{l}_{2}} & {{m}_{2}} & more...
(2) Line of shortest distance : If \[{{l}_{1}}\] and \[{{l}_{2}}\] are two skew lines, then the straight line which is perpendicular to each of these two non-intersecting lines is called the “Line of shortest distance.”
There is one and only one line perpendicular to each of lines \[{{l}_{1}}\] and \[{{l}_{2}}\].
(3) Shortest distance between two skew lines
Let two skew lines be, \[\frac{x-{{x}_{1}}}{{{l}_{1}}}=\frac{y-{{y}_{1}}}{{{m}_{1}}}=\frac{z-{{z}_{1}}}{{{n}_{1}}}\] and \[\frac{x-{{x}_{2}}}{{{l}_{2}}}=\frac{y-{{y}_{2}}}{{{m}_{2}}}=\frac{z-{{z}_{2}}}{{{n}_{2}}}\]
Therefore, the shortest distance between the lines is given by \[d=\frac{\left| \,\begin{matrix} {{x}_{2}}-{{x}_{1}} & {{y}_{2}}-{{y}_{1}} & {{z}_{2}}-{{z}_{1}} \\ {{l}_{1}} & {{m}_{1}} & {{n}_{1}} \\ {{l}_{2}} & {{m}_{2}} & more...
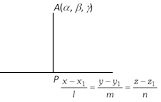 Length and equation of perpendicular : The length of the perpendicular is the distance AP and its equation is the line joining two known points A and P.
Length and equation of perpendicular : The length of the perpendicular is the distance AP and its equation is the line joining two known points A and P.
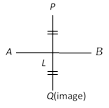 The length of the perpendicular is the perpendicular distance of given point from that line.
Reflection or image of a point in a straight line : If the perpendicular PL from point P on the given line be produced to more...
The length of the perpendicular is the perpendicular distance of given point from that line.
Reflection or image of a point in a straight line : If the perpendicular PL from point P on the given line be produced to more...
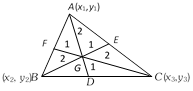 If \[A({{x}_{1}},{{y}_{1}})\], \[B({{x}_{2}},{{y}_{2}})\] and \[C({{x}_{3}},{{y}_{3}})\] are the vertices of a triangle. If G be the centroid upon one of the median (say) AD, then AG : GD = 2 : 1
\[\Rightarrow \] Co-ordinate of G are \[\left( \frac{{{x}_{1}}+{{x}_{2}}+{{x}_{3}}}{3},\frac{{{y}_{1}}+{{y}_{2}}+{{y}_{3}}}{3} \right)\]
(2) Circumcentre : The circumcentre of a triangle is the point of intersection of the perpendicular bisectors of the sides of a triangle. It is the centre of the circle which passes through the vertices of the triangle and so its distance from the vertices of the triangle is the same and this distance is more...
If \[A({{x}_{1}},{{y}_{1}})\], \[B({{x}_{2}},{{y}_{2}})\] and \[C({{x}_{3}},{{y}_{3}})\] are the vertices of a triangle. If G be the centroid upon one of the median (say) AD, then AG : GD = 2 : 1
\[\Rightarrow \] Co-ordinate of G are \[\left( \frac{{{x}_{1}}+{{x}_{2}}+{{x}_{3}}}{3},\frac{{{y}_{1}}+{{y}_{2}}+{{y}_{3}}}{3} \right)\]
(2) Circumcentre : The circumcentre of a triangle is the point of intersection of the perpendicular bisectors of the sides of a triangle. It is the centre of the circle which passes through the vertices of the triangle and so its distance from the vertices of the triangle is the same and this distance is more...
You need to login to perform this action.
You will be redirected in
3 sec
