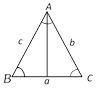
 where semiperimeter of triangle \[s=\frac{a+b+c}{2}\]
(3) When three sides and the circum-radius be given :
Area of triangle\[\Delta =\frac{abc}{4R}\], where R be the circum-radius of the triangle.
(4) When two angles and included side be given :
\[\Delta =\frac{1}{2}{{a}^{2}}\frac{\sin B\sin C}{\sin more...
where semiperimeter of triangle \[s=\frac{a+b+c}{2}\]
(3) When three sides and the circum-radius be given :
Area of triangle\[\Delta =\frac{abc}{4R}\], where R be the circum-radius of the triangle.
(4) When two angles and included side be given :
\[\Delta =\frac{1}{2}{{a}^{2}}\frac{\sin B\sin C}{\sin more...
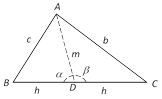 If \[\Delta \] be right angled, the mid point of hypotenuse is equidistant from the three vertices so that \[DA=DB=DC\].
\[\therefore {{b}^{2}}+{{c}^{2}}={{a}^{2}}\] which is pythagoras theorem. This theorem is very useful for solving problems of height and distance.
If \[\Delta \] be right angled, the mid point of hypotenuse is equidistant from the three vertices so that \[DA=DB=DC\].
\[\therefore {{b}^{2}}+{{c}^{2}}={{a}^{2}}\] which is pythagoras theorem. This theorem is very useful for solving problems of height and distance.
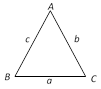 Similarly, we can deduct other projection formulae from sine rule.
(i)
Similarly, we can deduct other projection formulae from sine rule.
(i) 
| Functions | Periods |
| more...
(1) Check the validity of the given equation, e.g. \[2\sin \theta -\cos \theta =4\] can never be true for any \[\theta \] as the value \[(2\sin \theta -\cos \theta )\] can never exceeds \[\sqrt{{{2}^{2}}+{{(-1)}^{2}}}=\sqrt{5}\]. So there is no solution of this equation.
(2) Equation involving \[\sec \theta \] or \[\tan \theta \] can never have a solution of the form,\[(2n+1)\frac{\pi }{2}\].
Similarly, equations involving \[\text{cosec }\theta \] or \[\cot \theta \] can never have a solution of the form \[\theta =n\pi \]. The corresponding functions are undefined at these values of \[\theta \].
(3) If while solving an equation we have to square it, then the roots found after squaring must be checked whether they satisfy the original equation or not, e.g. let \[x=3\]. Squaring, we get \[{{x}^{2}}=9\] \[\therefore \] \[x=3\] and \[-\,3\] but \[x=-3\] does not satisfy the original equation \[x=3\].
(4) Do not cancel more...
Suppose we have to find the principal value of \[\theta \] satisfying the equation \[\sin \theta =-\frac{1}{2}\].
Since \[\sin \theta \] is negative, \[\theta \] will be in 3rd or 4th quadrant. We can approach 3rd or 4th quadrant from two directions. If we take anticlockwise direction the numerical value of the angle will be greater than \[\pi \]. If we approach it in clockwise direction the angle will be numerically less than \[\pi \]. For principal value, we have to take numerically smallest angle. So for principal value.
(1) If the angle is in 1st or 2nd quadrant we must select anticlockwise direction and if the angle is in 3rd or 4th quadrant, we must select clockwise direction.
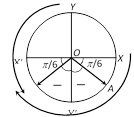 (2) Principal value is never numerically greater than \[\pi \].
(3) more...
(2) Principal value is never numerically greater than \[\pi \].
(3) more...
In \[a\cos \theta \,+b\sin \theta =c,\] put \[a=r\,\cos \alpha \] and \[b=r\,\sin \alpha \]where \[r=\sqrt{{{a}^{2}}+{{b}^{2}}}\] and \[|c|\le \sqrt{{{a}^{2}}+{{b}^{2}}}\]
Then,\[r\,(\cos \alpha \,\cos \theta +\sin \alpha \,\sin \theta )=c\]
\[\Rightarrow \,\,\cos (\theta -\alpha )=\frac{c}{\sqrt{{{a}^{2}}+{{b}^{2}}}}=\cos \beta \], (say) .....(i)
\[\Rightarrow \,\,\theta -\alpha =2n\pi \pm \beta \Rightarrow \,\theta =2n\pi \pm \beta +\alpha ,\] where \[\tan \alpha =\frac{b}{a}\] is the general solution.
Alternatively, putting \[a=r\,\sin \alpha \] and \[b=r\,\cos \alpha \],
where \[r=\sqrt{{{a}^{2}}+{{b}^{2}}}\] \[\Rightarrow \,\,\sin (\theta +\alpha )=\frac{c}{\sqrt{{{a}^{2}}+{{b}^{2}}}}=\sin \gamma \], (say)
\[\Rightarrow \,\,\theta +\alpha =n\pi +{{(-1)}^{n}}\gamma \]\[\Rightarrow \,\,\theta =n\pi +{{(-1)}^{n}}\gamma -\alpha ,\]
where \[\tan \alpha =\frac{a}{b}\] is the general solution.
\[(-\sqrt{{{a}^{2}}+{{b}^{2}}})\le \,a\cos \theta +b\sin \theta \le \,(\sqrt{{{a}^{2}}+{{b}^{2}}})\]
The general solution of \[a\cos x+b\sin x=c\] is
\[x=2n\pi +{{\tan }^{-1}}\left( \frac{b}{a} \right)\pm {{\cos }^{-1}}\left( \frac{c}{\sqrt{{{a}^{2}}+{{b}^{2}}}} \right)\].
Articles CategoriesArchive
Trending Articles
You need to login to perform this action. |