| Thousands | Hundreds | Tens | Ones |
| 1000 | 100 | 10 | 1 |
 Ascending Order of numbers:
Arranging numbers from the least number to the largest number is called Ascending Order.
e.g., 13, 67,132,168 are numbers in ascending order.
Descending Order of numbers:
Arranging numbers from the largest number to the least number is called Descending Order.
e.g., 168,132, 67, 13 are numbers in descending order.
Ascending Order of numbers:
Arranging numbers from the least number to the largest number is called Ascending Order.
e.g., 13, 67,132,168 are numbers in ascending order.
Descending Order of numbers:
Arranging numbers from the largest number to the least number is called Descending Order.
e.g., 168,132, 67, 13 are numbers in descending order.
| Symbol | Meaning | Example |
| = (is equal to) | The numbers on both the sides of = symbol have the same value. | 25 = 25 |
| < (is lesser than) | The number on the closed side of < symbol is lesser than the number on its open side. | 23 < 25 |
| > (is greater than) | The number on the open side of > symbol is greater than the number on its closed side. | 28 > 25 |
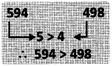 Rule 3: If the left more...
Rule 3: If the left more... | e.g., 5 + 3 = 8 \[\downarrow \] \[\downarrow \] \[\downarrow \] Addend Addend Sum |
| Note:- Adding two numbers in any order gives the same sum. e.g., 3 + 5 = 8 and 5 + 3 = 8 |
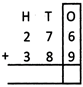 6 + 9 = 15
15 = 1 ten 5 ones
Write the ones under the ones place and carry the number in tens to the tens place.
6 + 9 = 15
15 = 1 ten 5 ones
Write the ones under the ones place and carry the number in tens to the tens place.
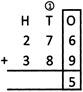 Then add the numbers in tens place along with the carry. Write the sum under tens place if it is less than or equal to 9.
Then add the numbers in tens place along with the carry. Write the sum under tens place if it is less than or equal to 9.
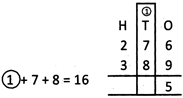 If the sum of tens is greater than 9, again regroup the tens as hundreds and tens. 16 tens = 1 hundred 6 tens.
If the sum of tens is greater than 9, again regroup the tens as hundreds and tens. 16 tens = 1 hundred 6 tens.
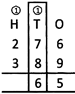 Write the tens in tens place and carry the hundreds to hundreds place. Add the numbers in hundreds place along with the carry and write the sum.
Write the tens in tens place and carry the hundreds to hundreds place. Add the numbers in hundreds place along with the carry and write the sum.
 So, 276 + 389 = 665.
Subtraction:
So, 276 + 389 = 665.
Subtraction:
| e.g., 24 \[\times \] 3 = 72 \[\downarrow \] \[\downarrow \] \[\downarrow \] Multiplicand Multiplier Product |
| Note: The product of a number and zero is zero. The product of a number and 1 is the number itself. Even if the order of the number to be multiplied is changed, the product remains the same. |
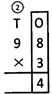 Step-2: Multiply 9 tens by 3.
9 tens \[\times \] 3 = 27 tens
= 20 tens + 7 tens
= 2 hundreds + 7 tens
Step-2: Multiply 9 tens by 3.
9 tens \[\times \] 3 = 27 tens
= 20 tens + 7 tens
= 2 hundreds + 7 tens
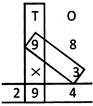 Add the carried 2 tens to 7 tens. So, 7 tens + 2 tens = 9 tens.
Write 9 in the tens place. Write 2 in hundreds place.
Add the carried 2 tens to 7 tens. So, 7 tens + 2 tens = 9 tens.
Write 9 in the tens place. Write 2 in hundreds place.
 Write 0 in the ones place and carry 3 tens to the tens place.
Step - 2: Multiply the digit in tens place of the multiplicand by the ones digit of the multiplier. 3 \[\times \] 5 = 15.
Now add the 3 carried from the product of ones to 15, which gives 15 + 3 = 18. Write 18 beside 0 in the product.
Step - 3: Now place a 0 below 0 in the product 180.
Write 0 in the ones place and carry 3 tens to the tens place.
Step - 2: Multiply the digit in tens place of the multiplicand by the ones digit of the multiplier. 3 \[\times \] 5 = 15.
Now add the 3 carried from the product of ones to 15, which gives 15 + 3 = 18. Write 18 beside 0 in the product.
Step - 3: Now place a 0 below 0 in the product 180.
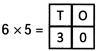
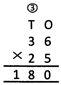 Multiply the digit 6 in ones place of the multiplicand by the digit 2 in tens place of multiplier. 6 \[\times \] 2 = 12
Write 2 below 8 in the more...
Multiply the digit 6 in ones place of the multiplicand by the digit 2 in tens place of multiplier. 6 \[\times \] 2 = 12
Write 2 below 8 in the more... | Note : Unlike addition, subtraction and multiplication which are done from the right most digit (i.e., ones) division is done from the left most digit of the dividend. |
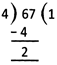 Step - 4: Bring down the next digit (ie., 7) beside 2 as shown.
Step - 4: Bring down the next digit (ie., 7) beside 2 as shown.
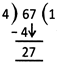 Find the number of 4's in the new dividend 27.
4 \[\times \] 6 = 24 and 4 \[\times \]7 = 28
So, there are 6 fours in the dividend 27. Write 6 beside 1 in the quotient and 24 below 27 as shown. Subtract 24 from 27 and write the difference 3 below the
separator line shown.
Find the number of 4's in the new dividend 27.
4 \[\times \] 6 = 24 and 4 \[\times \]7 = 28
So, there are 6 fours in the dividend 27. Write 6 beside 1 in the quotient and 24 below 27 as shown. Subtract 24 from 27 and write the difference 3 below the
separator line shown.  This is one whole.
One whole circle is shaded.
This is one whole.
One whole circle is shaded.
 The circle is divided into 2 equal parts.
1 out of the 2 equal parts is shaded.
That means \[\frac{1}{2}\] of the circle is shaded. We say one half of the circle is shaded. Two - halves is a whole.
The circle is divided into 2 equal parts.
1 out of the 2 equal parts is shaded.
That means \[\frac{1}{2}\] of the circle is shaded. We say one half of the circle is shaded. Two - halves is a whole.
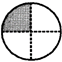 The circle is divided into 4 equal parts.
Each equal part is called a quarter.
1 out of the 4 equal parts is shaded.
That means - of the circle is shaded.
We say one quarter of the circle is shaded.
Four - quarters is a whole.
The circle is divided into 4 equal parts.
Each equal part is called a quarter.
1 out of the 4 equal parts is shaded.
That means - of the circle is shaded.
We say one quarter of the circle is shaded.
Four - quarters is a whole.
| Note: (1) One whole \[=1=\frac{2}{2}=\frac{3}{5}=\frac{4}{4}.........\] (2) Two quarters is a half. |
|
|
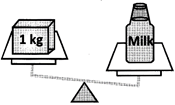 The milk bottle is heavier
than the 1 kg mass.
The milk bottle is heavier
than the 1 kg mass.
 The flour packet is as heavy as the 1 kg mass.
The flour packet is as heavy as the 1 kg mass.
 The bread is lighter than the 1 kg mass.
The bread is lighter than the 1 kg mass.
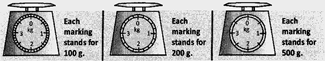
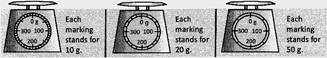
| · Mass can be measured in kilograms (kg) and grams (g). · 1 kg is equal to 1000 g. 1 kg is more than 1 g. · Half a kg is 500 g. |
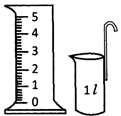
 CAPACITY
Volume is the amount of liquid (e.g., water Juice) in a container.
Capacity of a container is the volume of the liquid that it can hold.
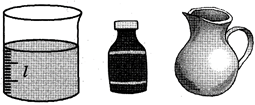
Volume is measured in litres (Z) and millilitres (m\[l\]).
1 I = 1000 m\[l\].
CAPACITY
Volume is the amount of liquid (e.g., water Juice) in a container.
Capacity of a container is the volume of the liquid that it can hold.
Volume is measured in litres (Z) and millilitres (m\[l\]).
1 I = 1000 m\[l\].
Do you remember these shapes?
|
 This figure is made up of only straight lines.
It has 5 straight lines.
This figure is made up of only straight lines.
It has 5 straight lines. |
 This figure is made up of only curved lines.
It has 8 curved lines.
This figure is made up of only curved lines.
It has 8 curved lines.
|
 This figure is made up of straight lines and curved lines. It has 3 straight lines and 3 curved liens.
This figure is made up of straight lines and curved lines. It has 3 straight lines and 3 curved liens.
|
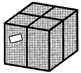 This parcel is made up of only flat surfaces. It has 6 flat surfaces. Some of them are hidden behind from our view.
This parcel is made up of only flat surfaces. It has 6 flat surfaces. Some of them are hidden behind from our view.
|
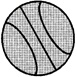 The ball is made up of only curved surfaces.
It has 1 curved surface.
The ball is made up of only curved surfaces.
It has 1 curved surface.
|
|
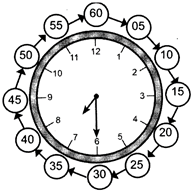
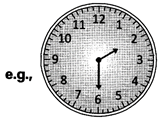 2: 30 is read as 30 minutes past 2 or half-past 2.
2: 30 is read as 30 minutes past 2 or half-past 2.
You need to login to perform this action.
You will be redirected in
3 sec
