Quadratic Equations
The most general quadratic equation is \[a{{x}^{2}}+bx+c=0\]. This equation can be solved by using the Discriminant method. In this method we find the discriminant of the given quadratic equation as follows:
\[D={{b}^{2}}-4ac,\]
(a) If D > 0, then the given equation will have real and distinct roots and we can find the roots of the given equation.
(b) If D = 0, then the given equation will have real and equal roots.
(c) If D < 0, then the given equation will have no real roots. In this case roots will be imaginary. Here we find the imaginary roots only.
In case of real roots we can find the roots by using the formula,
\[x=\frac{-b\pm \sqrt{{{b}^{2}}-4ac}}{2a}or\frac{-b\pm \sqrt{D}}{2a}\]
The quadratic equation can have maximum of two roots.
In case of imaginary roots we can find the roots by using the relation,
\[x=\frac{-b\pm more...
Introduction
In previous chapter we have studied about the polynomials. Quadratic equation is also a type of polynomial of degree two. The most general form of the quadratic equation is 
, where a, b, c are the coefficients. Solving the quadratic equation in general form has been worked out by some of the ancient mathematicians. In fact, Brahmagupta gave an explicit method to solve the quadratic equations. Later Sridharacharya derived special formulae for solving the quadratic equations known as the quadratic formula. One of the most prominent methods used in olden days mathematics for solving the quadratic equation was given by Bhaskar-11 for solving the quadratic equation by the completing square method.
Graphical Representation
Graphical Representation of Different Forms of Quadratic Equation
| Characteristic of the function | \[{{b}^{2}}-4\,ac\,<\,0\] | \[{{b}^{2}}-4\,ac\,\,0\] | \[{{b}^{2}}-4\,ac>0\] |
| When 'a' is positive | more...
Division of Polynomial
Previously we have studied about the division of the real numbers, in which we obtained quotient and remainders which satisfies the relation,
Dividend = Quotient \[\times \] Divisor + Remainder
This is also known as Euclid's division lemma. In this section we will discuss about the division of the polynomials which is known as the division algorithm for polynomials. The concept of division of the polynomials can be used for finding the zeroes of the cubic or biquadratic polynomials.
 Divide the polynomial \[g(x)={{x}^{3}}-3{{x}^{2}}+3{{x}^{2}}+3x-5\] by the polynomials \[h(x)={{x}^{2}}+x+1\] and find the quotient and remainder.
(a) \[(x-4,6x-1)\]
(b) \[(x+4,6x+1)\]
(c) \[({{x}^{2}}+1,3x+2)\]
(d) \[({{x}^{2}}+1,x-2)\]
(e) None of these
Answer: (a)
Explanation
When we divide \[g(x)\,by\,h(x)\] we have,
 more...
Introduction
Polynomials are an algebraic expression having many terms. We have studied about the polynomials in one variable in previous classes. There are different types of polynomials. The highest power of the polynomials is called degree of the polynomials. The polynomials of degree one is called linear polynomial. The polynomials of degree two are called quadratic polynomials. The polynomials of degree three are called cubic polynomials and the polynomials of degree four are called biquadratic polynomials. A real number which satisfies the given polynomials is called zeroes of the polynomials.
 Geometrical Meaning of Zeroes of Polynomial
If we represent linear polynomials on the graph we get a straight line. The straight line intersects the x axis at only one point. Thus number of zeroes of the linear polynomials is one. Hence we more...
 Introduction
Every number we have studied so far are real numbers. The real numbers are divided into two categories as rational and irrational numbers. All the positive counting numbers are called the natural numbers. It starts from 1 till infinity. The positive numbers which starts from zero are called whole numbers. The collections of natural numbers, their negatives along with the number zero are called integers the rational numbers are the numbers in the form \[\frac{p}{q},q\ne 0\] where p and q are integers.
Decimal Expansion of Rational Numbers
There are rational numbers which can be expressed as terminating decimals or non-terminating decimals. The non-terminating decimals may be repeating or non-repeating.. The rational number whose denominator has factor 2 or 5 are terminating and rest are non-terminating.
more...
Introduction
In mathematics, a linear equation is a equation in which the degree of the equation is one. Whether it is a linear equation in one variables or two variable or three variables they can be solved and solution can be found. For solving the system of linear equation in three or more variables, the concept of matrix is used which has been developed and introduced for the higher classes. In this chapter we will limit ourself up to the system of linear equation in three variables. The concept of system of linear equation has got wide application in solving the word problems based on day to day life situations.
A linear equation in two variables is a equation which contains a pair of variables which can be graphically represented in xy-plane in the form of coordinate system. By solution of the linear more...
 Graphical Representation
(A) Consistent Equation
It has two types of solutions: unique or infinitely many solutions.
(1) Unique Solution
If the graph of the pair of linear equation is intersecting each other at one point, then the system is said to have unique solution. The point at which the two lines intersect each other is called solution of the system of equation.
\[4x-6y=-4,8x+2y=48\]
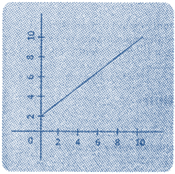
(2) Infinitely Many Solutions
If the graph of the pair of linear equation is coincident, then the system of linear equation is said to have infinitely many solutions.
\[y=3x+2\,and\,6x-2y+4=0\]
(B) Inconsistent Equation
It has only one type of solution, which is no solution.
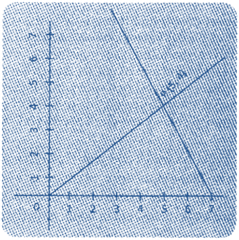
(1) No Solution
If the graph of the system of equation is more...
 Algebraic Method of Solving the System of Equation
There are different methods of solving the system of linear equations. The three different methods are:
(a) Elimination Method
(b) Substitution Method
(c) Cross Multiplication Method
 Elimination Method
In this method first we eliminate one of the variables by equating the coefficient of the one of the variable and finding the other variable. Then again re-substituting the value and getting the value of other variable.
Solve the system of the equation given by \[\frac{4}{16x+24z}+\frac{12}{21x-14z}=\frac{1}{2}\] and \[\frac{14}{4x+6z}+\frac{4}{(3x-2z)}=2\]
(a) \[(x=2,z=1)\]
(b) \[(x=3,z=5)\]
(c) \[(x=-4,z=1)\]
(d) \[(x=1,z=-1)\]
(e) None of these
Answer: (a)
 The value of x and y which satisfies the system of equation \[ax+ry=p+q\] and more...
Condition for Consistency
For the system of linear equation\[{{a}_{1}}x+{{b}_{1}}y={{c}_{1}}\,and\,{{a}_{2}}x+{{b}_{2}}\,y={{c}_{2}}\],
The types of solution the pair of linear equation \[3x+4y=7\, and \,4x-3y=7\] have?
(a) Unique solution
(b) No solution
(c) Infinitely many solution
(d) All of these
(e) None of these
Answer: (a)
Which one of the following is the condition for infinitely many solution?
(a) \[{{a}_{1}}{{a}_{2}}={{b}_{1}}{{b}_{2}}\]
(b) \[\frac{{{a}_{1}}}{{{a}_{2}}}\ne \frac{{{b}_{1}}}{{{b}_{2}}}\]
(c) \[\frac{{{a}_{1}}}{{{a}_{2}}}=\frac{{{b}_{1}}}{{{b}_{2}}}\ne \frac{{{c}_{1}}}{{{c}_{2}}}\]
(d) \[\frac{{{a}_{1}}}{{{a}_{2}}}=\frac{{{b}_{1}}}{{{b}_{2}}}=\frac{{{c}_{1}}}{{{c}_{2}}}\]
(e) None of these
Answer: (d)
The more...
Articles CategoriesArchive
Trending Articles
You need to login to perform this action. |
