(1) Number of permutations of \[n\] dissimilar things taken \[r\] at a time when p particular things always occur \[=\,{{\,}^{n-p}}{{C}_{r-p}}r!\].
(2) Number of permutations of \[n\] dissimilar things taken \[r\] at a time when \[p\] particular things never occur \[{{=}^{n-p}}{{C}_{r}}\,r!\].
(3) The total number of permutations of \[n\] different things taken not more than \[r\] at a time, when each thing may be repeated any number of times, is \[\frac{n({{n}^{r}}-1)}{n-1}\].
(4) Number of permutations of \[n\] different things, taken all at a time, when \[m\] specified things always come together is \[m\,!\,\times \,\,(n-m+1)\,!\].
(5) Number of permutations of \[n\] different things, taken all at a time, when \[m\] specified things never come together is \[n\,!-m\,!\,\,\times \,\,(n-m+1)\,!\].
(6) Let there be \[n\] objects, of which \[m\] objects are alike of one kind, and the remaining \[(n-m)\] objects are alike of another kind. Then, the total number of mutually distinguishable permutations that can be formed from these objects is \[\frac{n!}{(m\,!)\,\,\times \,\,(n-m)\,!}\].
The above theorem can be extended further i.e., if there are \[n\] objects, of which \[{{p}_{1}}\] are alike of one kind; \[{{p}_{2}}\] are alike of another kind; \[{{p}_{3}}\] are alike of \[{{3}^{rd}}\] kind;......; \[{{p}_{r}}\] are alike of \[{{r}^{th}}\] kind such that \[{{p}_{1}}+{{p}_{2}}+......+{{p}_{r}}=n\]; then the number of permutations of these n objects is \[\frac{n\,!}{({{p}_{1}}\,!)\times ({{p}_{2}}\,!)\times ......\times ({{p}_{r}}!)}\].
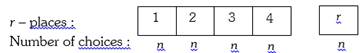 The number of permutations = The number of ways of filling \[r\] places \[={{(n)}^{r}}\].
(2) The number of arrangements that can be formed using \[n\] objects out of which \[p\] are identical (and of one kind) \[q\] are identical (and of another kind), \[r\] are identical (and of another kind) and the rest are distinct is \[\frac{n\,!}{p!\,q!r!}\].
The number of permutations = The number of ways of filling \[r\] places \[={{(n)}^{r}}\].
(2) The number of arrangements that can be formed using \[n\] objects out of which \[p\] are identical (and of one kind) \[q\] are identical (and of another kind), \[r\] are identical (and of another kind) and the rest are distinct is \[\frac{n\,!}{p!\,q!r!}\]. 