Division of Polynomials
Category : 10th Class
Previously we have studied about the division of the real numbers, in which we obtained quotient and remainders which satisfies the relation,
Dividend = Quotient \[\times \] Divisor + Remainder
This is also known as Euclid's division lemma. In this section we will discuss about the division of the polynomials which is known as the division algorithm for polynomials. The concept of division of the polynomials can be used for finding the zeroes of the cubic or biquadratic polynomials.

![]() Divide the polynomial \[g(x)={{x}^{3}}-3{{x}^{2}}+3{{x}^{2}}+3x-5\] by the polynomials \[h(x)={{x}^{2}}+x+1\] and find the quotient and remainder.
Divide the polynomial \[g(x)={{x}^{3}}-3{{x}^{2}}+3{{x}^{2}}+3x-5\] by the polynomials \[h(x)={{x}^{2}}+x+1\] and find the quotient and remainder.
(a) \[(x-4,6x-1)\]
(b) \[(x+4,6x+1)\]
(c) \[({{x}^{2}}+1,3x+2)\]
(d) \[({{x}^{2}}+1,x-2)\]
(e) None of these
Answer: (a)
Explanation
When we divide \[g(x)\,by\,h(x)\] we have,
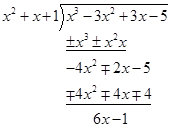
![]() Find the remaining two zeroes of the polynomial \[h(y)=3{{y}^{4}}+6{{y}^{3}}-2y-10y\,5\] if the two zeroes of the polynomial is \[\pm \sqrt{\frac{5}{3}}\].
Find the remaining two zeroes of the polynomial \[h(y)=3{{y}^{4}}+6{{y}^{3}}-2y-10y\,5\] if the two zeroes of the polynomial is \[\pm \sqrt{\frac{5}{3}}\].
(a) (- 1, 1)
(b) (-1, - 1)
(c) (+ 1, 2)
(d) (- 2 , 2)
(e) None of these
Answer: (b)
Explanation
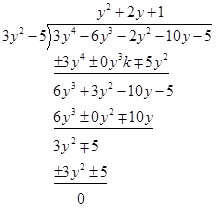
![]() Find the value of m and n such that \[{{z}^{2}}+1\] is the factor of \[g(z)={{z}^{4}}+{{z}^{3}}+8{{z}^{2}}+mz+n.\].
Find the value of m and n such that \[{{z}^{2}}+1\] is the factor of \[g(z)={{z}^{4}}+{{z}^{3}}+8{{z}^{2}}+mz+n.\].
(a) ( - 1, - 7)
(b) ( - 1, - 1)
(c) (1, 2)
(d) (1, 7)
(e) None of these
Answer: (d)
![]() Find the value of k and p in the polynomial \[m(z)={{z}^{4}}-6{{z}^{3}}+16{{z}^{2}}-25z+10\] is divisible by\[n(z)={{z}^{2}}-2z+k\], gives the remainder z + p.
Find the value of k and p in the polynomial \[m(z)={{z}^{4}}-6{{z}^{3}}+16{{z}^{2}}-25z+10\] is divisible by\[n(z)={{z}^{2}}-2z+k\], gives the remainder z + p.
(a) \[(k=-5,p=-7)\]
(b) \[(k=-5,p=-1)\]
(c) \[(k=5,p=-5)\]
(d) \[(k=1,p=7)\]
(e) None of these
Answer: (c)
![]() If the polynomial \[g(m)=6{{m}^{4}}+8{{m}^{3}}+17{{m}^{2}}+21m+7\] is divisible by another polynomial \[h(m)=3{{m}^{2}}+4m+1\] gives the remainder qm + a, then find the value of q and a.
If the polynomial \[g(m)=6{{m}^{4}}+8{{m}^{3}}+17{{m}^{2}}+21m+7\] is divisible by another polynomial \[h(m)=3{{m}^{2}}+4m+1\] gives the remainder qm + a, then find the value of q and a.
(a) (q = 5, a = -7)
(b) (q = 1, a = 2)
(c) (q = 2, a = -5)
(d) (q = 1, a = -2)
(e) None of these
Answer: (b)

![]()
You need to login to perform this action.
You will be redirected in
3 sec
