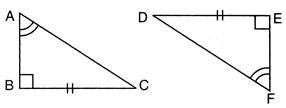
| Find the value of: |
| (a) \[15625\times \left( -2 \right)+\left( -15625 \right)\times 98\] |
| (b) \[18946\times 99-\left( -18946 \right)\] |
| Find: |
| (a) \[5\times 2\frac{3}{7}\] |
| (b) \[1\frac{4}{9}\times 6\] |
| Add and Subtract: |
| (a) m - n, m + n |
| (b) mn + 5 - 2, mn + 3. |
| Find: |
| (a) \[\frac{5}{6}-\frac{7}{3}\] |
| (b) \[3\frac{1}{5}-\left( -\frac{2}{3} \right)\] |
| When a = 0, b = -1, find the value of the given expressions: |
| (a) 2a+2b |
| (b) \[2{{a}^{2}}+\text{ }b2\text{ }+\text{ }1\] |
| (c) \[2{{a}^{2}}b+2a{{b}^{2}}+ab\] |
| Here are the shadows of some 3-D objects, when seen under the lamp of an overhead projector. Identify the solid(s) that match each shadow. (There may be multiple answers for these!) |
(a)  |
(b)  |
(c)  |
(d)  |
| (a) Construct 3 equations starting with x = 2 |
| (b) Construct 3 equations starting with x = -2 |
| Find |
| (a) \[\frac{-3}{7}+\frac{2}{3}\] |
| (b) \[\frac{-5}{6}+\frac{-3}{11}\] |
| Year | X | XII |
| 1994-95 | 90 | 95 |
| 1995-96 | 95 | 80 |
| 1996-97 | 90 | 85 |
| 1997-98 | 80 | 90 |
| 1998-99 | 98 | 95 |
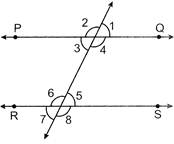
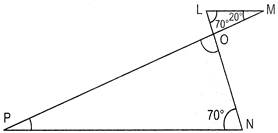
| Find the area of the following circles: |
| (a) radius = 14 mm (Take\[\pi =\frac{22}{7}\]) |
| (b) diameter = 49 m |
| (c) radius = 5 cm. |
| Simplify: |
| (a) \[\frac{{{12}^{4}}\times {{9}^{3}}\times 4}{{{6}^{3}}\times {{8}^{2}}\times 27}\] |
| (b) \[{{2}^{3}}\times {{a}^{3}}\times 5{{a}^{4}}\] |
You need to login to perform this action.
You will be redirected in
3 sec
