| Direction: A point object O is placed at the origin of coordinate system. An equi-convex thin lens\[({{\mu }_{g}}=1.5)\] of focal length \[f=20\,cm\]in air is placed so that its principal axis is along x-axis. Now the lens is cut at the middle (along the principal axis) and upper half is shifted along x-axis and y-axis by 20 cm and 2 mm respectively and right side of lower half is filled with water \[({{\mu }_{\omega }}=4/3)\] |
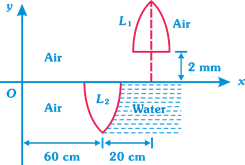 |
| Coordinates of the image produced by the lens \[{{L}_{2}}\] will be |
A) 140 cm, 0
B) 140 cm, 20
C) 70 cm, 0
D) 140 cm, 30
Correct Answer: A
Solution :
[a]| By refraction formula, |
| \[\frac{Mg}{{{v}_{1}}}-\frac{1}{(-60)}=\frac{Mg-1}{+20}\] ...(i) |
| \[\frac{{{\mu }_{w}}}{v}-\frac{Mg}{{{v}_{1}}}=\frac{{{\mu }_{w}}-{{\mu }_{g}}}{-20}\] ...(ii) |
| \[\therefore \] \[v=+\,80\text{ }cm\] |
| So, \[x=v+60=+\,80+60=140\text{ }cm\] |
You need to login to perform this action.
You will be redirected in
3 sec
