question_answer

A square loop of side length L carries a current which produces a magnetic field \[{{B}_{0}}\] at the centre \[(O)\] of the loop. Now the. Square loop is folded into two parts with one half being perpendicular to the other (see figure). Calculate the magnitude of magnetic field at the centre O.

A) \[\frac{{{B}_{0}}}{4}\]            

B) \[\frac{{{B}_{0}}}{\sqrt{2}}\]

C) \[2{{B}_{0}}\]             

D) Zero

Correct Answer: B

Solution :

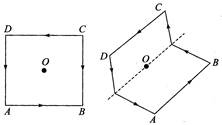
[b] \[{{B}_{0}}=\frac{4{{\mu }_{0}}I}{4\pi \frac{L}{2}}(\sin 45{}^\circ +\sin 45{}^\circ )=\frac{2\sqrt{2}{{\mu }_{0}}I}{\pi L}\] After folding \[{{\vec{B}}_{AB}}=\frac{{{\mu }_{0}}I}{4\pi \frac{L}{2}}(\sin 45{}^\circ +\sin 45{}^\circ )\hat{k}=\frac{2\sqrt{2}}{\pi }\frac{{{\mu }_{0}}I}{L}\hat{k}\]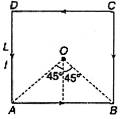 \[{{\vec{B}}_{BE}}=\frac{{{\mu }_{0}}I}{4\pi \frac{L}{2}}(\sin 0{}^\circ +\sin 45{}^\circ )\hat{k}=\frac{\sqrt{2}}{4\pi }\frac{{{\mu }_{0}}I}{L}\hat{k}\] Similarly, we have: \[{{\vec{B}}_{ac}}=\frac{\sqrt{2}{{\mu }_{0}}I}{4\pi L}\hat{i}\] 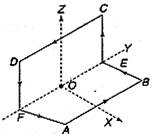\[{{\vec{B}}_{CD}}=\frac{2\sqrt{2}}{4\pi }\frac{{{\mu }_{0}}I}{L}\hat{i};\] \[{{\vec{B}}_{FA}}=\frac{\sqrt{2}}{4\pi }\frac{{{\mu }_{0}}I}{L}\hat{k}\] \[\vec{B}=\frac{\sqrt{2}{{\mu }_{0}}I}{\pi L}\hat{i}+\frac{\sqrt{2}{{\mu }_{0}}I}{\pi L}\hat{k}\] \[|\vec{B}|=\frac{2{{\mu }_{0}}I}{\pi L}=\frac{{{B}_{0}}}{\sqrt{2}}\]