JEE Main & Advanced Mathematics Rectangular Cartesian Coordinates Some Points of a Triangle

(1) Centroid of a triangle : The centroid of a triangle is the point of intersection of its medians. The centroid divides the medians in the ratio 2 : 1   (vertex : base)

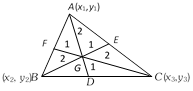
If \[A({{x}_{1}},{{y}_{1}})\], \[B({{x}_{2}},{{y}_{2}})\] and \[C({{x}_{3}},{{y}_{3}})\] are the vertices of a triangle. If G be the centroid upon one of the median (say) AD, then AG : GD = 2 : 1

\[\Rightarrow \] Co-ordinate of G are \[\left( \frac{{{x}_{1}}+{{x}_{2}}+{{x}_{3}}}{3},\frac{{{y}_{1}}+{{y}_{2}}+{{y}_{3}}}{3} \right)\]

(2) Circumcentre : The circumcentre of a triangle is the point of intersection of the perpendicular bisectors of the sides of a triangle. It is the centre of the circle which passes through the vertices of the triangle and so its distance from the vertices of the triangle is the same and this distance is known as the circum-radius of the triangle.

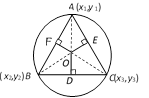
Let vertices A, B, C of the triangle ABC be \[({{x}_{1}},{{y}_{1}}),({{x}_{2}},{{y}_{2}})\] and \[({{x}_{3}},{{y}_{3}})\]and let circumcentre be \[O(x,\,\,y)\] and then \[(x,\,\,y)\] can be found by solving \[{{(OA)}^{2}}={{(OB)}^{2}}={{(OC)}^{2}}\]

i.e., \[{{(x-{{x}_{1}})}^{2}}+{{(y-{{y}_{1}})}^{2}}={{(x-{{x}_{2}})}^{2}}+{{(y-{{y}_{2}})}^{2}}\]\[={{(x-{{x}_{3}})}^{2}}+{{(y-{{y}_{3}})}^{2}}\]             

If a triangle is right angle, then its circumcentre is the mid point of hypotenuse. If angles of triangle i.e., A, B, C and vertices of triangle \[A({{x}_{1}},{{y}_{1}}),B({{x}_{2}},{{y}_{2}})\] and \[C\,({{x}_{3}},{{y}_{3}})\] are given, then circumcentre of the triangle ABC is

\[\left( \frac{{{x}_{1}}\sin 2A+{{x}_{2}}\sin 2B+{{x}_{3}}\sin 2C}{\sin 2A+\sin 2B+\sin 2C} \right.,\left. \frac{{{y}_{1}}\sin 2A+{{y}_{2}}\sin 2B+{{y}_{3}}\sin 2C}{\sin 2A+\sin 2B+\sin 2C} \right)\]

(3) Incentre : The incentre of a triangle is the point of intersection of internal bisector of the angles. Also it is a centre of a circle touching all the sides of a triangle.

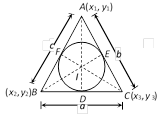
Co-ordinates of incentre

\[\left( \frac{a{{x}_{1}}+b{{x}_{2}}+c{{x}_{3}}}{a+b+c},\frac{a{{y}_{1}}+b{{y}_{2}}+c{{y}_{3}}}{a+b+c} \right)\]

where a, b, c are the sides of triangle ABC.

(4) Excircle : A circle touches one side outside the triangle and other two extended sides then circle is known as excircle. Let ABC be a triangle then there are three excircles with three excentres. Let \[{{I}_{1}},{{I}_{2}},{{I}_{3}}\] be the centres of ex-circles opposite to vertices A, B and C respectively. If vertices of triangle are \[A({{x}_{1}},{{y}_{1}}),\] \[B({{x}_{2}},{{y}_{2}})\] and \[C\,({{x}_{3}},{{y}_{3}})\], then 

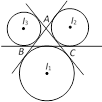
\[{{I}_{1}}\equiv \left( \frac{-a{{x}_{1}}+b{{x}_{2}}+c{{x}_{3}}}{-a+b+c},\frac{-a{{y}_{1}}+b{{y}_{2}}+c{{y}_{3}}}{-a+b+c} \right)\],

\[{{I}_{2}}\equiv \left( \frac{a{{x}_{1}}-b{{x}_{2}}+c{{x}_{3}}}{a-b+c},\frac{a{{y}_{1}}-b{{y}_{2}}+c{{y}_{3}}}{a-b+c} \right)\],

\[{{I}_{3}}\equiv \left( \frac{a{{x}_{1}}+b{{x}_{2}}-c{{x}_{3}}}{a+b-c},\frac{a{{y}_{1}}+b{{y}_{2}}-c{{y}_{3}}}{a+b-c} \right)\]

Angle bisector divides the opposite sides in the ratio of remaining sides e.g. \[\frac{BD}{DC}=\frac{AB}{AC}=\frac{c}{b}\].

Incentre divides the angle bisectors in the ratio \[(b+c):a,\text{  }(c+a):b\] and \[(a+b):c\].

Excentre : Point of intersection of one internal angle bisector and other two external angle bisector is called as excentre. There are three excentres in a triangle. Co-ordinate of each can be obtained by changing the sign of a, b, c respectively in the formula of in-centre.

(5) Orthocentre : It is the point of intersection of perpendiculars drawn from vertices on opposite sides (called altitudes) of a triangle and can be obtained by solving the equation of any two altitudes.      

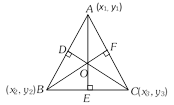
Here O is the orthocentre since \[AE\,\bot \,BC,\,BF\,\bot \,AC,\,\,CD\,\bot \,AB,\] then \[OE\,\,\bot \,BC,\,\,OF\,\bot \,AC\text{ and }\,OD\,\bot \,AB\]

Solving any two we can get coordinate of O.

The orthocentre of the triangle ABC is

\[\left( \frac{{{x}_{1}}\tan A+{{x}_{2}}\tan B+{{x}_{3}}\tan C}{\tan A+\tan B+\tan C},\ \frac{{{y}_{1}}\tan A+{{y}_{2}}\tan B+{{y}_{3}}\tan C}{\tan A+\tan B+\tan C} \right)\]

If a triangle is right angled triangle, then orthocentre is the point where right angle is formed.