SSC Sample Paper

done Mock Test-11 SSC CGL Tear-II Paper-1 Total Questions - 100    [Download]

• question_answer1)
  The number of digits in the square root of 625686734489 is
  A)
  4
  done clear
  B)
   5      
  done clear
  C)
   6
  done clear
  D)
   7
  done clear
  View Answer play_arrow

• question_answer2)
  If \[{{a}^{2x+2}}=1,\] where a is a positive real number other than 1, then x is equal to
  A)
   \[-\,\,2\]
  done clear
  B)
   \[-\,\,1\]
  done clear
  C)
   0
  done clear
  D)
   1
  done clear
  View Answer play_arrow

• question_answer3)
  \[\left[ 8-{{\left( \frac{{{4}^{\frac{9}{4}}}\sqrt{(2.2}{{)}^{2}}}{2\sqrt{{{2}^{-2}}}} \right)}^{\frac{1}{2}}} \right]\]is equal to
  A)
  32
  done clear
  B)
   8      
  done clear
  C)
   1
  done clear
  D)
   0
  done clear
  View Answer play_arrow

• question_answer4)
  The total number of integers between 200 and 400, each of which either begins with 3 or ends with 3 or both, is
  A)
  10
  done clear
  B)
   100    
  done clear
  C)
   110
  done clear
  D)
   120
  done clear
  View Answer play_arrow

• question_answer5)
  If \[a+\frac{1}{b}=1\] and \[b+\frac{1}{c}=1,\] then \[c+\frac{1}{a}\] is equal to
  A)
  0
  done clear
  B)
   \[\frac{1}{2}\]
  done clear
  C)
   1
  done clear
  D)
   2
  done clear
  View Answer play_arrow

• question_answer6)
  A number divided by 56 gives 29 as remainder. If the same number is divided by 8, the remainder will be
  A)
  7
  done clear
  B)
   6      
  done clear
  C)
   5
  done clear
  D)
   4
  done clear
  View Answer play_arrow

• question_answer7)
  If \[a:b:c=2:3:4\] and \[2a-3b+4c=33,\] then the value of c is
  A)
  6
  done clear
  B)
   9      
  done clear
  C)
   12
  done clear
  D)
   \[\frac{66}{7}\]
  done clear
  View Answer play_arrow

• question_answer8)
  If \[{{x}^{2}}-3x+1=0,\] then the value of \[x+\frac{1}{x}\] is
  A)
  0
  done clear
  B)
   1     
  done clear
  C)
   2
  done clear
  D)
   3
  done clear
  View Answer play_arrow

• question_answer9)
  The sum of two numbers is 45. Their difference is of their sum. Their LCM is
  A)
  200      
  done clear
  B)
   250    
  done clear
  C)
   100
  done clear
  D)
   150
  done clear
  View Answer play_arrow

• question_answer10)
  A man covers one-third of his journey at 30 km/h and the remaining two-third at 45 km/h. If the total journey is of 150 km, what is the average speed for whole journey?
  A)
  30 km/h
  done clear
  B)
   35 km/h
  done clear
  C)
   36 km/h
  done clear
  D)
   \[38\frac{4}{7}\text{km/h}\]
  done clear
  View Answer play_arrow

• question_answer11)
  There were 24 students in a class. One of them, who was 18 yr old, left the class and his place was filled up by a newcomer. If the average of the class there by was lowered by one month, the age of the newcomer is
  A)
  14 yr
  done clear
  B)
   15 yr  
  done clear
  C)
   16 yr   
  done clear
  D)
   17 yr
  done clear
  View Answer play_arrow

• question_answer12)
  64329 is divided by a certain number. While dividing, the numbers 175, 114 and 213 appear as three successive remainder. The divisor is
  A)
  184      
  done clear
  B)
   224    
  done clear
  C)
   234
  done clear
  D)
   296
  done clear
  View Answer play_arrow

• question_answer13)
  If \[x=3+\sqrt{8},\] then \[{{x}^{2}}+\frac{1}{{{x}^{2}}}\] equal  to
  A)
  38
  done clear
  B)
   36      
  done clear
  C)
   34
  done clear
  D)
   30
  done clear
  View Answer play_arrow

• question_answer14)
  A runs twice as fast as B and B runs thrice as fast as C. The distance covered by C in 72 min will be covered by A in
  A)
  6 min
  done clear
  B)
   8 min   
  done clear
  C)
   9 min
  done clear
  D)
   12 min
  done clear
  View Answer play_arrow

• question_answer15)
  In a school having roll strength 286, the ratio of boys and girls is 8 : 5. If 22 more girls get admitted into the school, the ratio of boys and girls becomes
  A)
  12 : 7
  done clear
  B)
  10 : 7  
  done clear
  C)
   8 : 7    
  done clear
  D)
   4 : 3
  done clear
  View Answer play_arrow

• question_answer16)
  The numbers 2272 and 875 are divided by a 3-digit number N, giving the same remainders. The sum of the digits of N is
  A)
  10
  done clear
  B)
   11      
  done clear
  C)
   12
  done clear
  D)
   13
  done clear
  View Answer play_arrow

• question_answer17)
  A 2-digit number is 3 times the sum of its digits. If 45 is added to the number, its digits are interchanged. The sum digits of the number is
  A)
  11
  done clear
  B)
   9  
  done clear
  C)
   7
  done clear
  D)
   5
  done clear
  View Answer play_arrow

• question_answer18)
  A rationalising factor of \[(\sqrt[3]{9}-\sqrt[3]{3}+1)\]is
  A)
   \[\sqrt[3]{3}-1\]
  done clear
  B)
   \[\sqrt[3]{3}+1\]
  done clear
  C)
   \[\sqrt[3]{9}+1\]
  done clear
  D)
   \[\sqrt[3]{9}-1\]
  done clear
  View Answer play_arrow

• question_answer19)
  A man covers half of his journey at 6 km/h and the remaining half at 3 km/h. His average speed is
  A)
  9 km/h
  done clear
  B)
   4.5 km/h
  done clear
  C)
   4 km/h
  done clear
  D)
   3 km/h
  done clear
  View Answer play_arrow

• question_answer20)
  At what rate "per cent per annum will a sum of Rs. 1000 amount of Rs. 1102.50 in 2 yr at compound interest?
  A)
  5%
  done clear
  B)
   5.5%    
  done clear
  C)
   6%
  done clear
  D)
   6.5%
  done clear
  View Answer play_arrow

• question_answer21)
  In an innings of a cricket match, three players A, B and C scored a total of 361 runs. If the ratio of the number of runs scored by A to that scored by B and also number of runs scored by 3 to that scored by 3 : 2, the number of run scored by A was
  A)
  171
  done clear
  B)
   181    
  done clear
  C)
   185     
  done clear
  D)
   16
  done clear
  View Answer play_arrow

• question_answer22)
  \[\left( \frac{1}{1\cdot 4}+\frac{1}{4\cdot 7}+\frac{1}{7\cdot 10}+\frac{1}{10\cdot 13}+\frac{1}{13\cdot 16} \right)\]is equal to
  A)
   \[\frac{1}{3}\]
  done clear
  B)
   \[\frac{5}{16}\]
  done clear
  C)
   \[\frac{3}{8}\]
  done clear
  D)
   \[\frac{41}{7280}\]
  done clear
  View Answer play_arrow

• question_answer23)
  What part of a ditch, 48 m long, 16.5 m broad and 4 m deep can be filled by the earth got by digging a cylindrical tunnel of diameter 4 m and length 56 m? \[\left( \text{Use}\,\,\pi =\frac{22}{7} \right)\]
  A)
   \[\frac{1}{9}\]
  done clear
  B)
   \[\frac{2}{9}\]
  done clear
  C)
   \[\frac{7}{9}\]
  done clear
  D)
   \[\frac{8}{9}\]
  done clear
  View Answer play_arrow

• question_answer24)
  Simple interest on Rs. 500 for 4 yr at 6.25% per annum is equal to the simple interest on Rs. 400 at 5% per annum for a certain period of time. The period of time is
  A)
  4 yr      
  done clear
  B)
   5 yr
  done clear
  C)
   \[6\frac{1}{4}\]yr
  done clear
  D)
   \[8\frac{2}{3}\]yr
  done clear
  View Answer play_arrow

• question_answer25)
  A right triangle with sides 3 cm, 4 cm and 5 cm is rotated about the side 3 cm to form a cone. The volume of the cone so formed is
  A)
   \[16\pi \,\,\text{c}{{\text{m}}^{3}}\]
  done clear
  B)
   \[12\pi \,\,\text{c}{{\text{m}}^{3}}\]
  done clear
  C)
   \[15\pi \,\,\text{c}{{\text{m}}^{3}}\]
  done clear
  D)
   \[20\pi \,\,\text{c}{{\text{m}}^{3}}\]
  done clear
  View Answer play_arrow

• question_answer26)
  A dealer buys an article marked Rs. 25000 with 20% and 5% off. He spends Rs. 1000 for its repairs and sells it for Rs. 25000. What is his gain or loss per cent?
  A)
  Loss of 25%      
  done clear
  B)
   Gain of 25%
  done clear
  C)
   Gain of 10%
  done clear
  D)
   Loss of 10%
  done clear
  View Answer play_arrow

• question_answer27)
  Given that \[\sqrt{24}\] is approximately equal to\[4.898.\]\[\sqrt{\frac{8}{3}}\]is nearly equal to
  A)
  0.544
  done clear
  B)
   1.333
  done clear
  C)
   1.633
  done clear
  D)
   2.666
  done clear
  View Answer play_arrow

• question_answer28)
  The area of a circle is increased by 22 cm, its radius is increased by 1 cm. The original radius of the circle is
  A)
  6 cm    
  done clear
  B)
   3.2 cm  
  done clear
  C)
   3 cm   
  done clear
  D)
   3.5 cm
  done clear
  View Answer play_arrow

• question_answer29)
  A student walks from his house at a Speed of \[2\frac{1}{2}\text{km/h}\] and reaches his school 6 min late. The next day he increase his speed by 1 km/h and reaches 6 min before school time. How far is the school from his house?
  A)
   \[\frac{5}{4}\,\,\text{km}\]
  done clear
  B)
   \[\frac{7}{4}\,\,\text{km}\]
  done clear
  C)
   \[\frac{9}{4}\,\,\text{km}\]
  done clear
  D)
   \[\frac{11}{4}\,\,\text{km}\]
  done clear
  View Answer play_arrow

• question_answer30)

  A company offers three types of successive discounts

  (i) 25% and 15%

  (ii) 30% and 10%

  (iii) 35% and 5%.

  Which offer is the best for a customer?

  A)
  First offer   
  done clear
  B)
                                            Second offer     
  done clear
  C)
                               Third offer   
  done clear
  D)
                                     Anyone, all are equally good    
  done clear
  View Answer play_arrow

• question_answer31)
  Two guns are fired from the same place at an interval of 6 min. A person approaching the place observes that 5 min 52 s have elapsed between the hearings of the sound of the two guns. If the velocity of the sound is 330 m/s, the man was approaching that place at what speed (in km/h)?          
  A)
  24
  done clear
  B)
   27    
  done clear
  C)
   30
  done clear
  D)
   36  
  done clear
  View Answer play_arrow

• question_answer32)
  The sum of the ages of a father and his son is 100 yr now. 5 yr ago, their ages were in the ratio of 2 : 1. The ratio of the ages of father and son after 10 yr will be
  A)
  5 : 3     
  done clear
  B)
   4 : 3   
  done clear
  C)
   10 : 7
  done clear
  D)
   3 : 5
  done clear
  View Answer play_arrow

• question_answer33)
  The average of 6 observations is 45.5. If one new observation is added to the previous observations, then the new average becomes 47. The new observation is    
  A)
                          58
  done clear
  B)
   56   
  done clear
  C)
   50
  done clear
  D)
   46 
  done clear
  View Answer play_arrow

• question_answer34)
  Three sets of English, Mathematics and Science books containing 336, 240, 96 books are respectively have to be stacked in such a way that all the books are stored subject wise and the height of each stack is the same. Total number of stacks will be
  A)
  14
  done clear
  B)
   21 
  done clear
  C)
   22
  done clear
  D)
   48
  done clear
  View Answer play_arrow

• question_answer35)
  With a given rate of simple interest, the ratio of principal and amount for a certain period of times is 4 : 5. After 3 yr, with the same rate of interest, the ratio of the principal and amount becomes 5 :7 rate of interest is  
  A)
                       4%
  done clear
  B)
   6%    
  done clear
  C)
   5%
  done clear
  D)
   7%
  done clear
  View Answer play_arrow

• question_answer36)
  In an examination, 60% of the candidates passed in English and 70% of the candidates passed in Mathematics, but 20% failed in both of these subjects. If 2500 candidates passed in both the subjects, the number of candidates that appeared at the examination was    
  A)
  3000
  done clear
  B)
   3500              
  done clear
  C)
   4000   
  done clear
  D)
   5000
  done clear
  View Answer play_arrow

• question_answer37)
  After measuring 120 m of a rope, it was discovered that the measuring metre rod was 3 cm longer. The true length of the rope measured is
  A)
  121 m 20 cm    
  done clear
  B)
   123 m 60 cm
  done clear
  C)
   123 m
  done clear
  D)
   116 m 40 cm
  done clear
  View Answer play_arrow

• question_answer38)
  The ratio 01 the number of boys and girls in a school is 3 : 2. If 20% of the boys and 30% of the girls are scholarship holders, then .the percentage of students, who do not get scholarship is
  A)
  50        
  done clear
  B)
   72     
  done clear
  C)
   75
  done clear
  D)
   76
  done clear
  View Answer play_arrow

• question_answer39)
  The ratio of incomes of A and B is 5 : 6. If A gets Rs. 1100 less than B, their total income (in Rs.) is
  A)
  9900
  done clear
  B)
   12100
  done clear
  C)
   14400
  done clear
  D)
   10000
  done clear
  View Answer play_arrow

• question_answer40)
  The marked price of a shirt and trousers are in the ratio 1 : 2. The shopkeeper gives 40% discount on the shirt. If the total discount on the set of the shirt and trousers is 30%. The discount offered on the rousers is
  A)
  15%     
  done clear
  B)
   20%   
  done clear
  C)
   25%    
  done clear
  D)
   30%
  done clear
  View Answer play_arrow

• question_answer41)
  The bus fare and train fare of a place from Kolkata were Rs. 20 and Rs. 30, respectively. Train fare has been increased by 20% and the bus fare has been increased by 10%. The ratio of new train fare to new bus fare is
  A)
  11 : 18
  done clear
  B)
   18 : 11
  done clear
  C)
   5 : 3    
  done clear
  D)
   3 : 5
  done clear
  View Answer play_arrow

• question_answer42)
  The perimeter of the floor of a room is 18 m. What is the area of the walls of the room, if the height of the room is 3 m?
  A)
   \[21\,\,{{\text{m}}^{2}}\]
  done clear
  B)
   \[42\,\,{{\text{m}}^{2}}\]
  done clear
  C)
   \[54\,\,{{\text{m}}^{2}}\]
  done clear
  D)
   \[108\,\,{{\text{m}}^{2}}\]
  done clear
  View Answer play_arrow

• question_answer43)
  \[0.\overline{142857}\div 0.\overline{285714}\]is equal to
  A)
  10
  done clear
  B)
   2   
  done clear
  C)
   \[\frac{1}{2}\]
  done clear
  D)
   \[\frac{1}{3}\]
  done clear
  View Answer play_arrow

• question_answer44)
  The length of a rectangle is increased by 10% and breadth decreased by 10%. Then, the area of the new rectangle is
  A)
  Neither decreased nor increased
  done clear
  B)
   Increased by 1%      
  done clear
  C)
                      Decreased by 1%
  done clear
  D)
   Decreased by 10%
  done clear
  View Answer play_arrow

• question_answer45)
  The average of 100 numbers is 44. The average of these 100 numbers and 4 other new numbers is 500. The average of the 4 new numbers will be
  A)
  800      
  done clear
  B)
   200   
  done clear
  C)
   176     
  done clear
  D)
   24
  done clear
  View Answer play_arrow

• question_answer46)
  Out of seven given numbers, the average of the first four numbers is 4 and that of the last four numbers is also 4. If the average of all the seven numbers is 3, fourth number is
  A)
  3
  done clear
  B)
   4      
  done clear
  C)
   7
  done clear
  D)
   11
  done clear
  View Answer play_arrow

• question_answer47)
  A liquid 'P' is \[1\frac{3}{7}\] times as heavy as water and water is \[1\frac{2}{3}\] times as heavy as another liquid 'Q'. The amount of liquid 'P' that must be added to 7 L of the liquid 'Q' so that the mixture may weight as much as an equal volume of water, will be
  A)
  7 L 
  done clear
  B)
   \[5\frac{1}{6}\text{L}\]
  done clear
  C)
   5 L
  done clear
  D)
   \[4\frac{2}{3}\text{L}\]
  done clear
  View Answer play_arrow

• question_answer48)
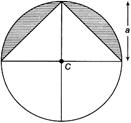
  The area of the shaded region in the figure given below is
  A)
   \[\frac{{{a}^{2}}}{2}\left( \frac{\pi }{2}-1 \right)\text{sq}\,\,\text{units}\]
  done clear
  B)
   \[{{a}^{2}}(\pi -1)\text{sq}\,\,\text{units}\]
  done clear
  C)
   \[{{a}^{2}}\left( \frac{\pi }{2}-1 \right)\text{sq}\,\,\text{units}\]
  done clear
  D)
   \[\frac{{{a}^{2}}}{2}(\pi -1)\text{sq}\,\,\text{units}\]
  done clear
  View Answer play_arrow

• question_answer49)
  A and B together can complete a work in 3 days. They start together but after 2 days, B left the work. If the work is completed after two more days, B alone could do the work in
  A)
  5 days
  done clear
  B)
   6 days  
  done clear
  C)
   9 days
  done clear
  D)
   10 days
  done clear
  View Answer play_arrow

• question_answer50)
  The speeds of A and B are in the ratio 3 : 4. A takes 20 min more than B to reach a destination. In what time does A reach the destination?
  A)
   \[1\frac{1}{3}\text{h}\]
  done clear
  B)
   \[2\,\,\text{h}\]
  done clear
  C)
   \[2\frac{2}{3}\,\,\text{h}\]
  done clear
  D)
   \[1\frac{2}{3}\,\,\text{h}\]
  done clear
  View Answer play_arrow

• question_answer51)
  The value of \[\sqrt{2+\sqrt{2+2\cos 4\theta }}\] is
  A)
   \[2{{\cos }^{2}}\theta \]
  done clear
  B)
   \[2\cos 2\theta \]
  done clear
  C)
   \[2\cos \theta \]
  done clear
  D)
   \[2\cos \frac{\theta }{2}\]
  done clear
  View Answer play_arrow

• question_answer52)
  If \[\sin \theta =\frac{{{m}^{2}}-{{n}^{2}}}{{{m}^{2}}+{{n}^{2}}},\]then what is the value of \[\tan \theta \]?
  A)
   \[\frac{{{m}^{2}}+{{n}^{2}}}{{{m}^{2}}-{{n}^{2}}}\]
  done clear
  B)
   \[\frac{2\,\,mn}{{{m}^{2}}+{{n}^{2}}}\]
  done clear
  C)
   \[\frac{{{m}^{2}}-{{n}^{2}}}{2mn}\]
  done clear
  D)
   \[\frac{{{m}^{2}}+{{n}^{2}}}{2\,\,mn}\]
  done clear
  View Answer play_arrow

• question_answer53)

  Consider the following equations

  I. \[\text{cose}{{\text{c}}^{2}}x+{{\sec }^{2}}x=\text{cose}{{\text{c}}^{2}}x{{\sec }^{2}}x\]

  II. \[{{\sec }^{2}}x+{{\tan }^{2}}x={{\sec }^{2}}x{{\tan }^{2}}x\]

  III. \[\text{cose}{{\text{c}}^{2}}x+{{\tan }^{2}}x={{\cot }^{2}}x+{{\sec }^{2}}x\]

  Which of the above statements are correct?

  A)
  Both I and II     
  done clear
  B)
   Both II and III
  done clear
  C)
   Both I and III   
  done clear
  D)
   I, and III
  done clear
  View Answer play_arrow

• question_answer54)
  If 9 lies in the third quadrant and \[3\tan \theta -4=0,\]then \[5\sin 2\theta +3\sin \theta +4\cos \theta \] is equal to
  A)
   \[0\]    
  done clear
  B)
   \[\frac{-\,\,24}{5}\]
  done clear
  C)
   \[\frac{24}{5}\]
  done clear
  D)
   \[\frac{48}{5}\]
  done clear
  View Answer play_arrow

• question_answer55)
  At a point P on the ground, the angle of elevation of the top of a 10m tall building and of a helicopter hovering some distance over the top of the building are \[30{}^\circ \]and \[60{}^\circ ,\]respectively. Then, the height of the helicopter above the ground is
  A)
   \[\frac{10}{\sqrt{3}}\,\,\text{m}\]
  done clear
  B)
   \[10\sqrt{3}\]
  done clear
  C)
   \[\frac{30}{\sqrt{3}}\,\,\text{m}\]
  done clear
  D)
   \[30\,\,\text{m}\]
  done clear
  View Answer play_arrow

• question_answer56)
  The radius and the slant height of a cone are in the ratio of 4 : 7. If its curved surface area is \[792\,\,c{{m}^{2}}.\]Then, the radius is
  A)
  10 cm
  done clear
  B)
   11 cm  
  done clear
  C)
   12 cm
  done clear
  D)
   13 cm
  done clear
  View Answer play_arrow

• question_answer57)
  The diameter of a right circular cone is 12 m and the slant height is 10 m. The total surface area of cone is
  A)
   \[\frac{2412}{7}\,\,{{\text{m}}^{2}}\]
  done clear
  B)
   \[\frac{2312}{7}\,\,{{\text{m}}^{2}}\]
  done clear
  C)
   \[\frac{2112}{7}\,\,{{\text{m}}^{2}}\]
  done clear
  D)
   \[\frac{2012}{7}\,\,{{\text{m}}^{2}}\]
  done clear
  View Answer play_arrow

• question_answer58)
  The circumference of the base of a 9 m high wooden solid cone is 44 m. The slant the height of the cone is
  A)
   \[\sqrt{120}\,\,\text{m}\]
  done clear
  B)
   \[\sqrt{130}\,\,\text{m}\]
  done clear
  C)
   \[\sqrt{150}\,\,\text{m}\]
  done clear
  D)
   \[7\sqrt{5}\,\,\text{m}\]
  done clear
  View Answer play_arrow

• question_answer59)
  In a trapezium ABCD, if AB||CD, then \[A{{C}^{2}}+B{{D}^{2}}\] equal to
  A)
   \[B{{C}^{2}}+A{{D}^{2}}+2AB\cdot CD\]
  done clear
  B)
   \[A{{B}^{2}}+C{{D}^{2}}2AD\cdot BC\]
  done clear
  C)
   \[A{{B}^{2}}+C{{D}^{2}}+2AB\cdot CD\]
  done clear
  D)
   \[B{{C}^{2}}+A{{D}^{2}}+2BC\cdot AD\]
  done clear
  View Answer play_arrow

• question_answer60)
  A point 0 in the interior of a rectangle ABCD is joined with each of the vertices A, B, C and D. Then,
  A)
   \[OB+OD=OC+OA\]
  done clear
  B)
   \[O{{B}^{2}}+O{{A}^{2}}=O{{C}^{2}}+O{{D}^{2}}\]
  done clear
  C)
   \[OB\cdot OD=OC\cdot OA\]
  done clear
  D)
   \[O{{B}^{2}}+O{{D}^{2}}=O{{C}^{2}}+O{{A}^{2}}\]
  done clear
  View Answer play_arrow

• question_answer61)
  The length of a rectangle is 2 cm more than its breadth. The perimeter is 48 cm. The area of the rectangle (in \[\text{c}{{\text{m}}^{2}}\]) is
  A)
  96
  done clear
  B)
   129    
  done clear
  C)
   143     
  done clear
  D)
   144
  done clear
  View Answer play_arrow

• question_answer62)
  If the perimeter of a rectangular field is 200 m and its breadth is 40 m, then its areas (In \[\text{c}{{\text{m}}^{2}}\]) is
  A)
  1200
  done clear
  B)
   2400   
  done clear
  C)
   3600   
  done clear
  D)
   4800
  done clear
  View Answer play_arrow

• question_answer63)
  The ratio between the number of sides of two polygons is 2 : 1 and the ratio between their interior angle is 4 : 3. The number of sides of these polygons are respectively
  A)
  8, 4      
  done clear
  B)
   10, 5   
  done clear
  C)
   12, 6
  done clear
  D)
   14, 7
  done clear
  View Answer play_arrow

• question_answer64)
  The ratio of the area of the equilateral triangle described on the side of a square to the area of the equilateral triangle described on its diagonal is
  A)
  1 : 3     
  done clear
  B)
   \[\sqrt{3}:4\]  
  done clear
  C)
   1 : 2    
  done clear
  D)
   1 : 4
  done clear
  View Answer play_arrow

• question_answer65)
  What are the factors of \[(6\sqrt{3}{{x}^{2}}-47x+5\sqrt{3})?\]
  A)
   \[(3\sqrt{3}x+5\sqrt{3})(2x-5\sqrt{3})\]
  done clear
  B)
  \[(3\sqrt{3}x-5\sqrt{3})(x\sqrt{3}x+1)\]
  done clear
  C)
   \[(2x+5\sqrt{3})(3\sqrt{3}x+1)\]
  done clear
  D)
   \[(2x-5\sqrt{3})(3\sqrt{3}x-1)\]
  done clear
  View Answer play_arrow

• question_answer66)
  The factors of \[({{m}^{2}}+17mn-84{{n}^{2}})\] are
  A)
   \[(m-21\,\,n)(m-4\,\,n)\]
  done clear
  B)
          \[(m+21\,\,n)(m-4\,\,n)\]
  done clear
  C)
   \[(m+n)(14\,\,m-2\,\,n)\]
  done clear
  D)
          None of the above
  done clear
  View Answer play_arrow

• question_answer67)
  What are the factor of \[\left( \frac{1}{3}{{x}^{2}}-2x-9 \right)?\]
  A)
   \[\frac{1}{3}(x-9)(x+3)\]
  done clear
  B)
   \[\frac{1}{3}(x-9)(x-3)\]
  done clear
  C)
  \[\frac{1}{3}(x+9)(x+3)\]
  done clear
  D)
   \[\frac{1}{3}(x+9)(x-3)\]
  done clear
  View Answer play_arrow

• question_answer68)
  The equation of the bisector of the acute angle between the lines \[2x-y+4=0\] and \[x-2y=1\] is
  A)
   \[x+y+5=0\]
  done clear
  B)
          \[x-y+1=0\]
  done clear
  C)
   \[x-y=5\]
  done clear
  D)
   None of these
  done clear
  View Answer play_arrow

• question_answer69)
  The equation of the line segment AB is \[y=x.\] If A and B lie on the same side of the line mirror \[2x-y=1,\]then the image of AB has the equation
  A)
   \[x+y=2\]
  done clear
  B)
   \[8x+y=9\]
  done clear
  C)
   \[7x-y=6\]
  done clear
  D)
   None of these
  done clear
  View Answer play_arrow

• question_answer70)
  The HCF of two polynomials is a + 5 and their LCM is \[(a+5)(a+4)(a-1).\] If one of the polynomial is \[{{a}^{2}}+4\,\,a-5,\] then the other polynomial is
  A)
   \[{{a}^{2}}+9a-20\]
  done clear
  B)
          \[{{a}^{2}}-9a+20\]
  done clear
  C)
   \[{{a}^{2}}+9a+20\]
  done clear
  D)
   \[{{a}^{2}}-9a-20\]
  done clear
  View Answer play_arrow

• question_answer71)
  The sum of the length of two diagonals of a square is144 cm, then the perimeter is square is
  A)
  144 cm
  done clear
  B)
   \[72\sqrt{2}\,\,\text{cm}\]
  done clear
  C)
   \[144\sqrt{2}\,\,\text{cm}\]
  done clear
  D)
   None of these
  done clear
  View Answer play_arrow

• question_answer72)
  In a circle of radius 42 cm, an arc subtends an angle of \[72{}^\circ \]at the centre. The length of the arc is
  A)
  52.8 cm
  done clear
  B)
   53.8 cm
  done clear
  C)
   72.8 cm
  done clear
  D)
   79.8 cm
  done clear
  View Answer play_arrow

• question_answer73)
  In figure, A ADE and \[\angle ABC\]are similar, if AC : BC =3 : 2, then the ratio \[\frac{DE}{AE}\] is
  A)
  3 : 2     
  done clear
  B)
   2 : 3     
  done clear
  C)
   1 : 3    
  done clear
  D)
   1 : 2
  done clear
  View Answer play_arrow

• question_answer74)
  AB and CD are two parallel lines. PQ cuts AB and CD at E and F, respectively. EL is the bisector of \[\angle FEB.\] If\[\angle LEB=35{}^\circ ,\] then \[\angle CFQ\] will be
  A)
   \[110{}^\circ \]
  done clear
  B)
   \[85{}^\circ \]
  done clear
  C)
   \[70{}^\circ \]
  done clear
  D)
   \[95{}^\circ \]
  done clear
  View Answer play_arrow

• question_answer75)
  To complete a work, A takes 50% more time than B. If together they take 18 days to complete the work, how much time shall B take to do it?
  A)
  30 days
  done clear
  B)
   35 days
  done clear
  C)
   40 days
  done clear
  D)
   45 days
  done clear
  View Answer play_arrow

• question_answer76)
  An article is listed at Rs. 900 and two successive discounts of 8% and 8% are given on it. How much would the seller gain or loss, if he gives a single discount of 16%, instead of two discounts?
  A)
  Gain of Rs. 4.76
  done clear
  B)
   Loss of Rs. 5.76
  done clear
  C)
   Gain of Rs. 5.76
  done clear
  D)
   Loss of Rs. 4.76
  done clear
  View Answer play_arrow

• question_answer77)
  A and B can complete a piece of work in 12 days and 18 days, respectively. A begins to do the work and they work alternatively one at a time for one day each. The whole work will be completed in
  A)
   \[14\frac{1}{3}\text{days}\]
  done clear
  B)
   \[15\frac{2}{3}\text{days}\]
  done clear
  C)
   \[16\frac{1}{3}\text{days}\]
  done clear
  D)
   \[18\frac{2}{3}\text{days}\]
  done clear
  View Answer play_arrow

• question_answer78)
  By selling 100 pencils, a shopkeeper gains the selling price of 20 pencils. His gain per cent is
  A)
  25
  done clear
  B)
   20     
  done clear
  C)
   15
  done clear
  D)
   12
  done clear
  View Answer play_arrow

• question_answer79)
  A pipe can fill a tank in 'x' hours and another pipe can empty it in 'y' \[(y>x)\]hours. If both the pipes are open, in how many hours will the tank be filled?
  A)
   \[r=\frac{6}{2}=3\,\,\text{cm}\]
  done clear
  B)
   \[(y-x)\,\,\text{hours}\]
  done clear
  C)
   \[\frac{xy}{x-y}\text{hours}\]
  done clear
  D)
   \[\frac{xy}{y-x}\text{hours}\]
  done clear
  View Answer play_arrow

• question_answer80)
  A train travelling at a speed of 30 m/s crosses a platform, 600 m long, in 30 s. The length (in metres) of train is
  A)
  120
  done clear
  B)
   150   
  done clear
  C)
   200
  done clear
  D)
   300
  done clear
  View Answer play_arrow

• question_answer81)
  A and B are two fixed points 5 cm apart and C is a point onA5 such that AC is 3 cm. If the length of AC is increased by 6%, the length of CB is decreased by
  A)
  6%
  done clear
  B)
   7%    
  done clear
  C)
   8%
  done clear
  D)
   9%
  done clear
  View Answer play_arrow

• question_answer82)
  A fruit seller buys lemons at 2 for Rs. 1 and sells them at 5 for Rs. 3. His profit per cent is
  A)
  10
  done clear
  B)
   15     
  done clear
  C)
   20
  done clear
  D)
   25
  done clear
  View Answer play_arrow

• question_answer83)
  A steamer goes downstream from one port to another in 4 h. It covers the same distance upstream in 5 h. If the speed of stream is 2 km/h, the distance between the two ports is
  A)
  50 km
  done clear
  B)
   60 km  
  done clear
  C)
   70 km
  done clear
  D)
   80 km
  done clear
  View Answer play_arrow

• question_answer84)
  Rs. 395 are divided among A, B and C in such a manner that B gets 25 % more than A and 20 % more than C. The share of A will be
  A)
  Rs. 195
  done clear
  B)
   Rs. 180   
  done clear
  C)
   Rs. 98
  done clear
  D)
   Rs. 120
  done clear
  View Answer play_arrow

• question_answer85)
  The speed of a motorboat is that of the stream of water as 36: 5. The boat goes along with the stream in 5 h 10 min. It will come back in
  A)
  5 h 50 min
  done clear
  B)
   6 h
  done clear
  C)
   6 h 50 min
  done clear
  D)
   12 h 10 min
  done clear
  View Answer play_arrow

• question_answer86)
  A pen is listed for Rs. 12. A discount of 15 % is given on it. A second discount is given bringing the price down to Rs. 8.16. The rate of second discount is
  A)
  20%     
  done clear
  B)
   15%   
  done clear
  C)
   18%
  done clear
  D)
   25%
  done clear
  View Answer play_arrow

• question_answer87)
  Rs. 1000 is invested at 5% per annum simple interest. If the interest is added to the principal after every 10 yr, the amount will become Rs. 2000 after
  A)
  15 yr
  done clear
  B)
   18 yr   
  done clear
  C)
   20 yr   
  done clear
  D)
   \[16\frac{2}{3}\]yr
  done clear
  View Answer play_arrow

• question_answer88)
  Two trains 105 m and 90 m long, run at the speeds of 45 km/h and 72 km/h respectively, in opposite directions on parallel tracks. The time which they take to cross each other is
  A)
  8 s       
  done clear
  B)
   6 s  
  done clear
  C)
   7 s
  done clear
  D)
   5 s
  done clear
  View Answer play_arrow

• question_answer89)
  The price of a certain item is increased by 15%. If a consumer wants to keep his expenditure on the item the same as before, how much per cent must be reduce his consumption on that item?
  A)
   \[15%\]
  done clear
  B)
   \[13\frac{1}{23}%\]
  done clear
  C)
   \[16\frac{2}{3}%\]
  done clear
  D)
   \[10\frac{20}{23}%\]
  done clear
  View Answer play_arrow

• question_answer90)
  The volumes of a sphere and a right circular cylinder having the same radius are equal. The ratio of the diameter of the sphere to the height of the cylinder is
  A)
  3 : 2     
  done clear
  B)
   2 : 3   
  done clear
  C)
   1 : 2    
  done clear
  D)
   2 : 1
  done clear
  View Answer play_arrow

• question_answer91)
  Directions (Q. Nos. 91 and 92): In each of the following number series, a wrong number is given. Find out that number. 2, 3, 5, 8, 14, 23, 41, 69
  A)
  41
  done clear
  B)
   69     
  done clear
  C)
   54
  done clear
  D)
   99
  done clear
  View Answer play_arrow

• question_answer92)
  108, 54, 36, 18, 9, 6, 4
  A)
  9
  done clear
  B)
   36       
  done clear
  C)
   6
  done clear
  D)
   18
  done clear
  View Answer play_arrow

• question_answer93)

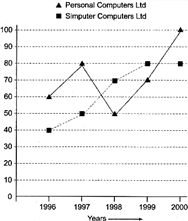
  Directions: Study the following graph carefully and answer the questions given below.

  Sales of two companies Personal Computers Ltd and Simputer Computers Ltd during last five years

  What is the difference between the average sales figure of the two companies during the last 5 yr, (in Rs. crore) ?
  A)
  8
  done clear
  B)
   7      
  done clear
  C)
   6
  done clear
  D)
   5
  done clear
  View Answer play_arrow

• question_answer94)

  Directions: Study the following graph carefully and answer the questions given below.

  Sales of two companies Personal Computers Ltd and Simputer Computers Ltd during last five years

  What is the average percentage growth for company Personal Computer Ltd during last 5 yr?
  A)
   \[70\frac{1}{3}%\]
  done clear
  B)
   \[68\frac{3}{4}%\]
  done clear
  C)
   \[66\frac{2}{3}%\]
  done clear
  D)
   \[65\frac{1}{3}%\]
  done clear
  View Answer play_arrow

• question_answer95)

  Directions: Study the following graph carefully and answer the questions given below.

  Sales of two companies Personal Computers Ltd and Simputer Computers Ltd during last five years

  The sales of company Personal Computer Ltd in 1999 is what per cent of the sales of company Simputer Computer Ltd in 1996?
  A)
  75
  done clear
  B)
   125     
  done clear
  C)
   150     
  done clear
  D)
   175
  done clear
  View Answer play_arrow

• question_answer96)

  Directions: Study the following graph carefully and answer the questions given below.

  Sales of two companies Personal Computers Ltd and Simputer Computers Ltd during last five years

  If the profit for company Simputer Computer Ltd 1998 was 40%, what was the input cost in that year
  A)
  Rs. 60 crore
  done clear
  B)
          Rs. 50 crore
  done clear
  C)
   Rs. 45 crore      
  done clear
  D)
   Rs. 40 crore
  done clear
  View Answer play_arrow

• question_answer97)

  Directions: Study the following graph carefully and answer the questions given below.

  Sales of two companies Personal Computers Ltd and Simputer Computers Ltd during last five years

  In which year company Simputer Company Ltd had maximum annual percentage growth?
  A)
  1996
  done clear
  B)
   1997  
  done clear
  C)
   1998   
  done clear
  D)
   1999
  done clear
  View Answer play_arrow

• question_answer98)

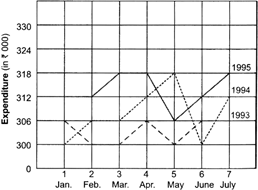
  Directions: Study the data presented in the following graph to answer the questions.

  Monthly expenditure of a firm from January to July during the years 1993, 1994 and 1995

  What is the total expenditure during the period under review (7 months) in 1993?
  A)
  Rs. 2107000
  done clear
  B)
          Rs. 2196000
  done clear
  C)
   Rs. 2124000     
  done clear
  D)
   Rs. 2240000
  done clear
  View Answer play_arrow

• question_answer99)

  Directions: Study the data presented in the following graph to answer the questions.

  Monthly expenditure of a firm from January to July during the years 1993, 1994 and 1995

  What total expenditure has been made during the year 1993 and 1994 in the period covered in the graph?  
  A)
                                  Rs. 4287000      
  done clear
  B)
   Rs. 270000      
  done clear
  C)
   Rs. 4827000     
  done clear
  D)
   Rs. 4278000  
  done clear
  View Answer play_arrow

• question_answer100)

  Directions: Study the data presented in the following graph to answer the questions.

  Monthly expenditure of a firm from January to July during the years 1993, 1994 and 1995

  What is the average monthly expenditure during the year 1995 cover the period shown in the graph
  A)
  275000
  done clear
  B)
   270000
  done clear
  C)
   314000
  done clear
  D)
   247000
  done clear
  View Answer play_arrow