Answer:
Let the young man drives x km and y km at 25 km/h and 40 km/h speed respectively, then LPP is maximise distance \[Z=x+y\] Subject to the constraints \[2x+5y\le 100\] \[\frac{x}{25}+\frac{y}{40}\le 1\]or \[8x+5y\le 200\] and \[x,\,\,y\ge 0\] 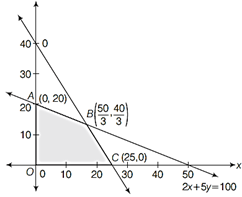 The feasible region of the LPP is shaded in figure. The coordinates of the corner-points of the feasible region OABC are O (0, 0), A (0, 20), \[B\,\left( \frac{50}{3},\,\,\frac{40}{3} \right)\] and C (25, 0).
The feasible region of the LPP is shaded in figure. The coordinates of the corner-points of the feasible region OABC are O (0, 0), A (0, 20), \[B\,\left( \frac{50}{3},\,\,\frac{40}{3} \right)\] and C (25, 0).
\[\therefore \]Maximum distance covered is 30 km with\[\frac{50}{3}\,km\] at 25 km/h speeds respectively. Value Vehicle should be driven at a moderate speed to decrease the pollution. Corner Point Value of Z \[A\,(0,\,\,20)\] 20 \[B\left( \frac{50}{3},\,\,\frac{40}{3} \right)\] 30 (maximum) \[C\,(25,\,\,0)\] 25
You need to login to perform this action.
You will be redirected in
3 sec
