question_answer

Following figure shows on adiabatic cylindrical container of volume \[{{V}_{0}}\] divided by an adiabatic smooth piston (area of cross-section = A) in two equal parts. An ideal gas \[({{C}_{P}}/{{C}_{V}}=\gamma )\] is at pressure P1 and temperature T1 in left part and gas at pressure P2 and temperature T2 in right part. The piston is slowly displaced and released at a position where it can stay in equilibrium. The final pressure of the two parts will be (Suppose x = displacement of the piston)

A)            \[{{P}_{2}}\]                         

B)            \[{{P}_{1}}\]

C)            \[\frac{{{P}_{1}}{{\left( \frac{{{V}_{0}}}{2} \right)}^{\gamma }}}{{{\left( \frac{{{V}_{0}}}{2}+Ax \right)}^{\gamma }}}\]                

D)            \[\frac{{{P}_{2}}{{\left( \frac{{{V}_{0}}}{2} \right)}^{\gamma }}}{{{\left( \frac{{{V}_{0}}}{2}+Ax \right)}^{\gamma }}}\]

Correct Answer: C

Solution :

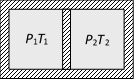
                   As finally the piston is in equilibrium, both the gases must be at same pressure \[{{P}_{f}}\]. It is given that displacement of piston be in final state x and if A is the area of cross-section of the piston. Hence the final volumes of the left and right part finally can be given by figure as \[{{V}_{L}}=\frac{{{V}_{0}}}{2}+Ax\] and \[{{V}_{R}}=\frac{{{V}_{0}}}{2}-Ax\] As it is given that the container walls and the piston are adiabatic in left side and the gas undergoes adiabatic expansion and on the right side the gas undergoes adiabatic compressive. Thus we have for initial and final state of gas on left side                    \[{{P}_{1}}{{\left( \frac{{{V}_{0}}}{2} \right)}^{\gamma }}={{P}_{f}}{{\left( \frac{{{V}_{0}}}{2}+Ax \right)}^{\gamma }}\]                          .....(i)                    Similarly for gas in right side, we have                    \[{{P}_{2}}{{\left( \frac{{{V}_{0}}}{2} \right)}^{\gamma }}={{P}_{f}}{{\left( \frac{{{V}_{0}}}{2}-Ax \right)}^{\gamma }}\]                          .....(ii)                    From eq. (i) and (ii)                    \[\frac{{{P}_{1}}}{{{P}_{2}}}=\frac{{{\left( \frac{{{V}_{0}}}{2}+Ax \right)}^{\gamma }}}{{{\left( \frac{{{V}_{0}}}{2}-Ax \right)}^{\gamma }}}\] Þ \[Ax=\frac{{{V}_{0}}}{2}\frac{\left[ P_{1}^{1/\gamma }-P_{2}^{1/\gamma } \right]}{\left[ P_{1}^{1/\gamma }+P_{2}^{1/\gamma } \right]}\]            Now from equation (i) \[{{P}_{f}}=\frac{{{P}_{1}}{{\left( \frac{{{V}_{0}}}{2} \right)}^{\gamma }}}{{{\left[ \frac{{{V}_{0}}}{2}+Ax \right]}^{\gamma }}}\]