Area of Triangle
Category : JEE Main & Advanced
Let three angles of \[\Delta ABC\] are denoted by \[A,\,\,B,\,\,C\] and the sides opposite to these angles by letters \[a,\,\,b,\,\,c\] respectively.
(1) When two sides and the included angle be given :
The area of triangle ABC is given by,
\[\Delta =\frac{1}{2}bc\sin A=\frac{1}{2}ca\sin B=\frac{1}{2}ab\sin C\]
i.e., \[\Delta =\frac{1}{2}\] (Product of two sides) \[\times \] sine of included angle
(2) When three sides are given :
Area of \[\Delta ABC=\,\Delta =\sqrt{s(s-a)(s-b)(s-c)}\]
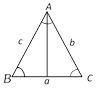
where semiperimeter of triangle \[s=\frac{a+b+c}{2}\]
(3) When three sides and the circum-radius be given :
Area of triangle\[\Delta =\frac{abc}{4R}\], where R be the circum-radius of the triangle.
(4) When two angles and included side be given :
\[\Delta =\frac{1}{2}{{a}^{2}}\frac{\sin B\sin C}{\sin (B+C)}=\frac{1}{2}{{b}^{2}}\frac{\sin A\sin C}{\sin (A+C)}=\frac{1}{2}{{c}^{2}}\frac{\sin A\sin B}{\sin (A+B)}\]
You need to login to perform this action.
You will be redirected in
3 sec
