question_answer

As shown in fig. when a spherical cavity (centred at O) of radius 1 is cut out of a uniform sphere of radius R (centred at C), the centre of mass of remaining (shaded) part of sphere is at G, i.e. on the surface of the cavity. R can be determined by the equation                                                [JEE MAIN Held on 08-01-2020 Evening]

A) \[\left( {{R}^{2}}\text{+}R+1 \right)\left( 2R \right)=1\]

B) \[\left( {{R}^{2}}R+1 \right)\left( 2-R \right)=1\]

C) \[\left( {{R}^{2}}R1 \right)\left( 2R \right)\,\,\text{=}\,1\]

D) \[\left( {{R}^{2}}+R1 \right)\left( 2R \right)=1\]

Correct Answer: A

Solution :

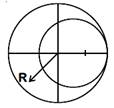\[{{M}_{0}}=\frac{4}{3}\pi {{R}^{3}}\rho \] \[{{M}_{cavity}}=\frac{4}{3}\pi {{\left( 1 \right)}^{3}}\rho \] \[{{M}_{\left( \operatorname{Re}maining \right)}}=\frac{4}{3}\pi {{R}^{3}}\rho -\frac{4}{3}\pi {{\left( 1 \right)}^{3}}\rho \] \[\therefore \left( \frac{4}{3}\pi {{R}^{3}}\rho -\frac{4}{3}\pi {{\left( 1 \right)}^{3}}\rho  \right)\times 2+\frac{4}{3}\pi {{\left( 1 \right)}^{3}}\rho =\frac{4}{3}\pi {{R}^{3}}\rho \cdot R\]        \[\Rightarrow \,\,\,\,\,{{R}^{4}}-2{{R}^{3}}+1=0\]      \[\because \,\,\,\,\,\,\,R\ne 1\] \[\therefore \,\,\,\,\,\,\,{{R}^{3}}-{{R}^{2}}-R-1=0\] \[\Rightarrow \,\,\left( {{R}^{2}}+R+1 \right)\left( 2-R \right)=1\]