SSC Sample Paper

done Mock Test-10 SSC CGL Tear-II Paper-1 Total Questions - 100    [Download]

• question_answer1)
  The value of \[\left( 1+\frac{1}{x} \right)\left( 1+\frac{1}{x+1} \right)\left( 1+\frac{1}{x+2} \right)\]\[\left( 1+\frac{1}{x+3} \right)\]is
  A)
   \[1+\frac{1}{x+4}\]
  done clear
  B)
   \[x+4\]
  done clear
  C)
   \[\frac{1}{x}\]
  done clear
  D)
   \[\frac{x+4}{x}\]
  done clear
  View Answer play_arrow

• question_answer2)
  The square of a natural number subtracted from its cube is 48. The number is
  A)
   8
  done clear
  B)
   6
  done clear
  C)
   5
  done clear
  D)
   4
  done clear
  View Answer play_arrow

• question_answer3)
  \[{{[1-2{{(1-2)}^{-1}}]}^{-1}}\] equal to
  A)
   \[\frac{1}{3}\]
  done clear
  B)
   \[-\,\,\frac{1}{3}\]
  done clear
  C)
   \[-\,\,1\]
  done clear
  D)
   \[\frac{1}{2}\]
  done clear
  View Answer play_arrow

• question_answer4)
  When a number is divided by 121, the remainder is 25. If the same number is divided by 11, the remainder will be
  A)
   3
  done clear
  B)
   4
  done clear
  C)
   6
  done clear
  D)
   25
  done clear
  View Answer play_arrow

• question_answer5)
  The    digit    in    the    unit's    place    of \[[{{(251)}^{98}}+{{(21)}^{29}}-{{(106)}^{100}}+{{(705)}^{35}}-{{16}^{4}}+259]\] is
  A)
   1
  done clear
  B)
   4
  done clear
  C)
   5
  done clear
  D)
   6
  done clear
  View Answer play_arrow

• question_answer6)
  The square root of \[({{272}^{2}}-{{128}^{2}})\]is
  A)
   256
  done clear
  B)
   200
  done clear
  C)
   240
  done clear
  D)
   144
  done clear
  View Answer play_arrow

• question_answer7)
  The LCM and the HCF of the numbers 28 and 42 are in the ratio
  A)
   6 : 1    
  done clear
  B)
   2 : 3
  done clear
  C)
   3 : 2
  done clear
  D)
   7 : 2
  done clear
  View Answer play_arrow

• question_answer8)
  The value of\[\sqrt{\frac{{{(0.1)}^{2}}+{{(0.01)}^{2}}+{{(0.009)}^{2}}}{{{(0.01)}^{2}}+{{(0.001)}^{2}}+{{(0.0009)}^{2}}}}\]is equal to
  A)
   1
  done clear
  B)
   10
  done clear
  C)
   100
  done clear
  D)
   0.1
  done clear
  View Answer play_arrow

• question_answer9)
  \[\left( \frac{\sqrt{625}}{11}\times \frac{14}{\sqrt{25}}\times \frac{11}{\sqrt{196}} \right)\] is equal to
  A)
   6
  done clear
  B)
   5
  done clear
  C)
   8
  done clear
  D)
   11
  done clear
  View Answer play_arrow

• question_answer10)
  The smallest integer, which when subtracted from both the terms of 6 : 7 gives a ratio less than 16 : 21 is
  A)
   5
  done clear
  B)
   4
  done clear
  C)
   3
  done clear
  D)
   2
  done clear
  View Answer play_arrow

• question_answer11)
  \[{{25}^{25}}\] is divided by 26, the remainder is
  A)
   1
  done clear
  B)
   2
  done clear
  C)
   24
  done clear
  D)
   25
  done clear
  View Answer play_arrow

• question_answer12)
  A man crosses a road 250 m wide in 75 s. His speed (in km/h) is
  A)
   10
  done clear
  B)
   12  
  done clear
  C)
   12.5    
  done clear
  D)
   15
  done clear
  View Answer play_arrow

• question_answer13)
  If m : n = 3 : 2, then (4 m + 5 n): \[(4\,\,m-5\,\,n)\] is equal to
  A)
   4 : 9    
  done clear
  B)
   9 : 4
  done clear
  C)
   11 :1
  done clear
  D)
   9 : 1
  done clear
  View Answer play_arrow

• question_answer14)
  The average of 9 numbers is 30. The average of first 5 numbers is 25 and that of the last 3 numbers is 35. What is the 6th number?
  A)
   20
  done clear
  B)
   30
  done clear
  C)
   40
  done clear
  D)
   50
  done clear
  View Answer play_arrow

• question_answer15)
  The average of 11 numbers is 10.8. If the average the first six be 10.4 and that of the last six is 11.5, then the middle (6th) number is
  A)
   10.3    
  done clear
  B)
   12.6
  done clear
  C)
   13.5    
  done clear
  D)
   15.5
  done clear
  View Answer play_arrow

• question_answer16)
  \[(7.5\times 7.5+37.5+2.5\times 2.5)\]is equal to
  A)
   100     
  done clear
  B)
   80
  done clear
  C)
   60
  done clear
  D)
   30
  done clear
  View Answer play_arrow

• question_answer17)
  \[{{\left( \frac{\sqrt{5}+\sqrt{3}}{\sqrt{5}-\sqrt{3}} \right)}^{2}}+{{\left( \frac{\sqrt{5}-\sqrt{3}}{\sqrt{5}+\sqrt{3}} \right)}^{2}}\] is equal to
  A)
   64
  done clear
  B)
   62
  done clear
  C)
   66
  done clear
  D)
   68
  done clear
  View Answer play_arrow

• question_answer18)
  When a ball bounces, it rises to \[\frac{3}{4}\] of the height from which it fell. If the ball is dropped from a height of 32 m, how high will it rise at the third bounce?
  A)
   \[14\frac{1}{2}\text{m}\]
  done clear
  B)
   \[13\frac{1}{2}\text{m}\]
  done clear
  C)
   13 m
  done clear
  D)
   None of these
  done clear
  View Answer play_arrow

• question_answer19)
  The sides of a triangle are in the ratio \[\frac{1}{2}:\frac{1}{3}:\frac{1}{4}\]If the perimeter of the triangle is 52 cm, the length of the smallest side is
  A)
   24 cm
  done clear
  B)
   10 cm
  done clear
  C)
   12 cm
  done clear
  D)
   9 cm
  done clear
  View Answer play_arrow

• question_answer20)
  If the difference between the compound interest, impounded every six months and the simple interest on a certain sum of money at the rate of 12% per annum for one year is Rs. 36, the sum is
  A)
   Rs. 10000
  done clear
  B)
   Rs. 12000
  done clear
  C)
   Rs. 15000
  done clear
  D)
   Rs. 9000
  done clear
  View Answer play_arrow

• question_answer21)
  In how many years will Rs. 2000 amount to Rs. 2420 at 10% per annum compound interest?
  A)
   3
  done clear
  B)
   \[2\frac{1}{2}\]
  done clear
  C)
   2
  done clear
  D)
   \[1\frac{1}{2}\]
  done clear
  View Answer play_arrow

• question_answer22)
  A rain passes a 50 m long platform in 14 s and a man standing on the platform in 10 s. The speed of the train is
  A)
   24 km/h
  done clear
  B)
   36 km/h    
  done clear
  C)
   40 km/h
  done clear
  D)
   45 km/h
  done clear
  View Answer play_arrow

• question_answer23)
  The area of a circle of a radius 5 cm is numerically what per cent of its circumference?
  A)
   200     
  done clear
  B)
   225
  done clear
  C)
   240
  done clear
  D)
   250
  done clear
  View Answer play_arrow

• question_answer24)
  If men or 20 women can do a piece of work in 25 days, in what time will 28 men and 15 women do
  A)
   \[14\frac{2}{7}\text{days}\]
  done clear
  B)
   \[33\frac{1}{3}\text{days}\]
  done clear
  C)
   \[18\frac{3}{4}\text{days}\]
  done clear
  D)
   10 days
  done clear
  View Answer play_arrow

• question_answer25)
  If \[\frac{a}{b}=\frac{2}{3}\]and \[\frac{b}{c}=\frac{4}{5},\]then the ratio \[\frac{a+b}{b+c}\]equal to
  A)
   \[\frac{20}{27}\]          
  done clear
  B)
   \[\frac{27}{20}\]
  done clear
  C)
   \[\frac{6}{8}\]
  done clear
  D)
   \[\frac{8}{6}\]
  done clear
  View Answer play_arrow

• question_answer26)
  A and B can do a job in 6 and 12 days respectively. They began the work together but A leaves after 3 days Then, the total number, of days needed for completion of the work is
  A)
   4
  done clear
  B)
   5
  done clear
  C)
   6
  done clear
  D)
   9
  done clear
  View Answer play_arrow

• question_answer27)
  If a number is divisible by both 11 and 13, then it must be necessarily
  A)
   divisible by \[(11+13)\]  
  done clear
  B)
   divisible by \[(13-11)\]
  done clear
  C)
   divisible by \[(11\times 13)\]
  done clear
  D)
   429
  done clear
  View Answer play_arrow

• question_answer28)
  A and B starts at the same time with speeds of 40 km/h and 50 km/h respectively. If in covering the journey A takes 15 min longer than B, the total distance of the journey is
  A)
   46 km
  done clear
  B)
   48 km
  done clear
  C)
   50 km
  done clear
  D)
   52 km
  done clear
  View Answer play_arrow

• question_answer29)
  What per cent decrease in salaries would exactly cancel out the 20% increase?
  A)
   20%
  done clear
  B)
   \[16\frac{2}{3}%\]
  done clear
  C)
  \[33\frac{1}{3}%\]
  done clear
  D)
   \[18%\]
  done clear
  View Answer play_arrow

• question_answer30)
  A certain number of men can complete a job in 30 days. If there were 5 men more, it could be completed in 10 days less. How many men were in the beginning?
  A)
   10
  done clear
  B)
   15     
  done clear
  C)
   20
  done clear
  D)
   25
  done clear
  View Answer play_arrow

• question_answer31)
  One-third of the square root of which number is 0.001?
  A)
   0.0009
  done clear
  B)
   0.000001
  done clear
  C)
   0.00009
  done clear
  D)
   None of these
  done clear
  View Answer play_arrow

• question_answer32)
  The sum of two numbers is 40 and their difference is 4. Then, the ratio of the numbers is
  A)
   21 : 19
  done clear
  B)
   22 : 9
  done clear
  C)
   11 : 9
  done clear
  D)
   11 : 18
  done clear
  View Answer play_arrow

• question_answer33)
  A can go round a circular path 8 times in 40 min, If the diameter of the circle is increased to 10 times the original diameter, the time required by A to go round the new path once travelling at the same speed as before is   
  A)
                          25 min
  done clear
  B)
   20 min  
  done clear
  C)
   50 min
  done clear
  D)
   100 min
  done clear
  View Answer play_arrow

• question_answer34)
  If food prices go up by 10%, by how much should a man reduce his consumption so as not to increase his expenditure?
  A)
   \[9\frac{1}{11}%\]
  done clear
  B)
   10%
  done clear
  C)
   \[11\frac{1}{9}%\]
  done clear
  D)
   None of these
  done clear
  View Answer play_arrow

• question_answer35)
  Tap 'A' can fill the cistern in 5 h and the another tap 'B' can empty that cistern in 4 h. How much time it will take to empty the cistern if both the taps are opened simultaneously when the cistern is already full?
  A)
   10 h    
  done clear
  B)
   16 h   
  done clear
  C)
   18 h    
  done clear
  D)
   20 h
  done clear
  View Answer play_arrow

• question_answer36)
  The length of a train and that of a platform are equal. If with a speed of 90 km/h the train crosses the platform in one minute, then the length of the train (in metres) is
  A)
   500     
  done clear
  B)
   600
  done clear
  C)
   750
  done clear
  D)
   900
  done clear
  View Answer play_arrow

• question_answer37)
  The HCF of two numbers is 8. Which one of the following can never by their LCM?
  A)
   24
  done clear
  B)
   48
  done clear
  C)
   56       
  done clear
  D)
   60
  done clear
  View Answer play_arrow

• question_answer38)
  The average wage of 500 workers was found to be Rs. 200. Later on it was discovered that the wages of two workers were misread as 180 and 20 instead of 80 and 220. The correct average wage is
  A)
   Rs. 200.10
  done clear
  B)
   Rs. 200.20
  done clear
  C)
   Rs. 200.50
  done clear
  D)
   Rs. 201.00
  done clear
  View Answer play_arrow

• question_answer39)
  The sum of the squares of three consecutive natural numbers is 2030. Then, what is the middle number?
  A)
   25
  done clear
  B)
   26
  done clear
  C)
   27
  done clear
  D)
   28
  done clear
  View Answer play_arrow

• question_answer40)
  If doubling a number and adding 20 to the result gives the same answer as multiplying the number by 8 and taking away 4 from the product, the number is
  A)
   2
  done clear
  B)
   3
  done clear
  C)
   4
  done clear
  D)
   6
  done clear
  View Answer play_arrow

• question_answer41)
  If the sum of two numbers is 22 and the sum of their squares is 404, then the product of the numbers is
  A)
   40
  done clear
  B)
   44
  done clear
  C)
   80
  done clear
  D)
   88
  done clear
  View Answer play_arrow

• question_answer42)
  The compound interest on Rs. 10000 in 2 yr at 4% per annum, the interest being compounded half-yearly is
  A)
   Rs. 636.80
  done clear
  B)
   Rs. 824.32
  done clear
  C)
   Rs. 912.86
  done clear
  D)
   Rs. 828.82
  done clear
  View Answer play_arrow

• question_answer43)
  If the 4th term of an arithmetic progression is 14 and the 12th term is 70, then the first term is
  A)
   \[-10\]  
  done clear
  B)
   \[-7\] 
  done clear
  C)
   \[+7\]
  done clear
  D)
   \[+10\]
  done clear
  View Answer play_arrow

• question_answer44)
  The difference of two numbers is 20% of the larger number. If the smaller number is 20, the larger number is
  A)
   25
  done clear
  B)
   45      
  done clear
  C)
   50
  done clear
  D)
   80
  done clear
  View Answer play_arrow

• question_answer45)
  When 75% of a number is added to 75, the result is the same number. Find the number
  A)
   225     
  done clear
  B)
   270    
  done clear
  C)
   300     
  done clear
  D)
   325
  done clear
  View Answer play_arrow

• question_answer46)
  A works twice as fast as B. If both of them can together finish a work in 12 days, B alone can do it in
  A)
   48 days
  done clear
  B)
   36 days
  done clear
  C)
   27 days
  done clear
  D)
   24 days
  done clear
  View Answer play_arrow

• question_answer47)
  If the circumference and area of a circle are numerically equal, then the diameter is equal to
  A)
   area of the circle
  done clear
  B)
  \[\frac{\pi }{2}\]
  done clear
  C)
   \[2\pi \]
  done clear
  D)
   4
  done clear
  View Answer play_arrow

• question_answer48)
  A, B and C are partners of a company. During a particular year A received one-third of the profit, B received one-fourth of the profit and C received remaining Rs. 5000. How much did A receive?
  A)
   Rs. 5000
  done clear
  B)
   Rs. 4000 
  done clear
  C)
   Rs. 3000
  done clear
  D)
   Rs. 1000     
  done clear
  View Answer play_arrow

• question_answer49)
    The product of two numbers is 120 and the sum their squares is 289. What would be the different between the two numbers?   
  A)
               3
  done clear
  B)
   5            
  done clear
  C)
   7
  done clear
  D)
   None of these  
  done clear
  View Answer play_arrow

• question_answer50)
  How many digits in all are required to write numbers from 1 to 50?  
  A)
                    100     
  done clear
  B)
   92     
  done clear
  C)
   91
  done clear
  D)
   50
  done clear
  View Answer play_arrow

• question_answer51)
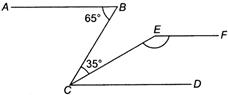
  AB and CD are two parallel lines. The points B and C are joined such that \[\angle ABC=65{}^\circ .\]A line CE is drawn making angle of \[35{}^\circ \]with the line CB, EF is drawn parallel to AB, as shown in figure, then \[\angle CEF\]is equal to                                
  A)
   \[160{}^\circ \]
  done clear
  B)
   \[155{}^\circ \]
  done clear
  C)
   \[150{}^\circ \]
  done clear
  D)
   \[145{}^\circ \]
  done clear
  View Answer play_arrow

• question_answer52)
  In \[\Delta ABC,\]\[AD\bot BC,\] then 
  A)
                 \[A{{B}^{2}}-B{{D}^{2}}=A{{C}^{2}}-C{{D}^{2}}\]
  done clear
  B)
              \[A{{B}^{2}}+B{{D}^{2}}=A{{C}^{2}}-C{{D}^{2}}\]  
  done clear
  C)
              \[A{{B}^{2}}+B{{D}^{2}}=A{{C}^{2}}+C{{D}^{2}}\] 
  done clear
  D)
           \[A{{B}^{2}}+A{{C}^{2}}=B{{D}^{2}}+D{{C}^{2}}\]  
  done clear
  View Answer play_arrow

• question_answer53)
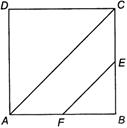
         In figure, ABCD is square. F is the mid-point of AB, BE is one-third of BC. If the area of the\[\Delta \,\,FBE\] is 108 sq cm. Then, the length of AC is      
  A)
   \[36\sqrt{3}\,\,\text{cm}\]
  done clear
  B)
   \[35\sqrt{2}\,\,\text{cm}\]  
  done clear
  C)
   \[12\sqrt{3}\,\,\text{cm}\]
  done clear
  D)
   \[36\sqrt{2}\,\,\text{cm}\]
  done clear
  View Answer play_arrow

• question_answer54)
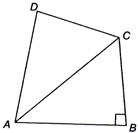
    In  a  quadrilateral  ABCD, \[\angle B=90{}^\circ \] and \[A{{D}^{2}}=A{{B}^{2}}+B{{C}^{2}}+C{{D}^{2}}.\] Then, \[\Delta ADC\] is
  A)
   equilateral triangle
  done clear
  B)
   isosceles
  done clear
  C)
   right angled triangle
  done clear
  D)
   None of these
  done clear
  View Answer play_arrow

• question_answer55)
  A rectangular grassy plot is 110 m by 65 m. It has a uniform path 2.5 m wide all around it on the inside. The area of the path is
  A)
   \[750\,\,\text{c}{{\text{m}}^{2}}\]
  done clear
  B)
   \[850\,\,\text{c}{{\text{m}}^{2}}\]
  done clear
  C)
   \[950\,\,\text{c}{{\text{m}}^{2}}\]
  done clear
  D)
   \[1050\,\,\text{c}{{\text{m}}^{2}}\]
  done clear
  View Answer play_arrow

• question_answer56)
  The circumference of a circle is 176 m. Then, its
  A)
   \[2464\,\,{{\text{m}}^{2}}\]
  done clear
  B)
   \[2164\,\,{{\text{m}}^{2}}\]
  done clear
  C)
   \[2346\,\,{{\text{m}}^{2}}\]
  done clear
  D)
   \[2246\,\,{{\text{m}}^{2}}\]
  done clear
  View Answer play_arrow

• question_answer57)
  A solid cylinder has a total surface area of \[231\,\,{{\text{m}}^{2}}.\] Its curved surface area is\[\frac{2}{3}\]of the total surface area. The volume of the cylinder is
  A)
   \[269\frac{1}{2}\,\,{{\text{m}}^{3}}\]
  done clear
  B)
   \[259\frac{1}{2}\,\,{{\text{m}}^{3}}\]
  done clear
  C)
   \[249\frac{1}{2}\,\,{{\text{m}}^{3}}\]
  done clear
  D)
   \[239\frac{1}{2}\,\,{{\text{m}}^{3}}\]
  done clear
  View Answer play_arrow

• question_answer58)
  A conical tent of a diameter 24 m at the base and its heights 16m. The canvas required to make it is
  A)
   \[\frac{5280}{7}\,\,{{\text{m}}^{2}}\]
  done clear
  B)
   \[\frac{5180}{7}\,\,{{\text{m}}^{2}}\]
  done clear
  C)
   \[\frac{4180}{7}\,\,{{\text{m}}^{2}}\]
  done clear
  D)
   \[\frac{3480}{7}\,\,{{\text{m}}^{2}}\]
  done clear
  View Answer play_arrow

• question_answer59)
  The area of a rhombus whose one side and one diagonal measure 20 cm and 24 cm respectively is
  A)
   \[364\,\,\text{c}{{\text{m}}^{2}}\]
  done clear
  B)
   \[374\,\,\text{c}{{\text{m}}^{2}}\]
  done clear
  C)
   \[384\,\,\text{c}{{\text{m}}^{2}}\]
  done clear
  D)
   \[394\,\,\text{c}{{\text{m}}^{2}}\]
  done clear
  View Answer play_arrow

• question_answer60)
  The diagonals of the three faces of a cuboid are x, y, and z respectively. What is the volume of the cuboid?
  A)
   \[\frac{xyz}{2\sqrt{2}}\]
  done clear
  B)
                      \[\frac{\sqrt{({{y}^{2}}+{{z}^{2}}-{{x}^{2}})({{z}^{2}}+{{x}^{2}}-{{y}^{2}})({{x}^{2}}+{{y}^{2}}-{{z}^{2}})}}{2\sqrt{2}}\]
  done clear
  C)
   \[\frac{\sqrt{({{y}^{2}}+{{z}^{2}})({{z}^{2}}+{{x}^{2}})({{x}^{2}}+{{y}^{2}})}}{2\sqrt{2}}\]
  done clear
  D)
   None of these
  done clear
  View Answer play_arrow

• question_answer61)
  The length, breadth and height of a rectangular parallelepiped are in ratio 6:3:1. If the surface area of a cube is equal to the surface area of this parallelepiped, then what is the ratio of the volume of the cube to the volume of the parallelepiped?
  A)
   1 : 1    
  done clear
  B)
   5 : 4   
  done clear
  C)
   7 : 5    
  done clear
  D)
   3 : 2
  done clear
  View Answer play_arrow

• question_answer62)
  A hollow cylindrical tube, open at both ends is made of iron 1 cm thick. The volume of iron used in making the tube, if the external diameter is 12 cm and the length of tube is 70 cm, is
  A)
   \[\text{Distance=}\frac{\text{Product}\,\,\text{of}\,\,\text{speeds}}{\text{Distance}\,\,\text{of}\,\,\text{speeds}}\times \text{Difference}\,\,\text{in}\,\,\text{time}\]
  done clear
  B)
   \[2520\,\,\text{c}{{\text{m}}^{3}}\]
  done clear
  C)
   \[2720\,\,\text{c}{{\text{m}}^{3}}\]
  done clear
  D)
   \[2900\,\,\text{c}{{\text{m}}^{3}}\]
  done clear
  View Answer play_arrow

• question_answer63)
  If \[(x+2)\] is the HCF of \[{{x}^{2}}+ax+b\] and \[{{x}^{2}}+cx+d\]\[(a\ne c\,\,\text{and}\,\,b\ne d),\] then which one of the following is correct?
  A)
   \[a+c=b+d\]
  done clear
  B)
          \[2a+b=2c+d\]
  done clear
  C)
   \[b+2c=2a+d\]
  done clear
  D)
   \[b-2c=2a-d\]
  done clear
  View Answer play_arrow

• question_answer64)
  If the HCF of \[{{x}^{3}}-27\] and \[{{x}^{3}}+4{{x}^{2}}+12x+k\] is a quadratic polynomial, then what is the value of K?
  A)
   27       
  done clear
  B)
   9
  done clear
  C)
   3
  done clear
  D)
   \[-\,\,3\]
  done clear
  View Answer play_arrow

• question_answer65)
  If \[\frac{5}{x}+6y=13\]and \[\frac{3}{x}+4y=7,\] find the value of y.
  A)
   \[-\,\,2\]
  done clear
  B)
   3       
  done clear
  C)
   4
  done clear
  D)
   5
  done clear
  View Answer play_arrow

• question_answer66)
  \[\frac{x+y-8}{2}=\frac{x+2y-14}{3}=\frac{3x+y-12}{11}\] find the value of x and y,
  A)
   \[-\,\,6,\,\,-2\]
  done clear
  B)
   \[-\,\,5,4\]
  done clear
  C)
   \[-\,\,2,6\]
  done clear
  D)
   \[2,6\]
  done clear
  View Answer play_arrow

• question_answer67)
  If \[a+b+c=6,\]\[{{a}^{2}}+{{b}^{2}}+{{c}^{2}}=26,\]then what is the value of \[ab+bc+ca?\]
  A)
   0
  done clear
  B)
   2
  done clear
  C)
   4
  done clear
  D)
   5
  done clear
  View Answer play_arrow

• question_answer68)
  Consider the following I. \[{{\tan }^{2}}\theta -{{\sin }^{2}}\theta ={{\tan }^{2}}\theta {{\sin }^{2}}\theta \] II. \[(\text{cosec}\theta -\sin \theta )(\sec \theta -\cos \theta )(\tan \theta +\cot \theta )=1\] Which of the above is/are correct?
  A)
   Only I is the identity
  done clear
  B)
   Only II is the identity
  done clear
  C)
   Both I and II are the identities
  done clear
  D)
   Neither I nor II is the identity
  done clear
  View Answer play_arrow

• question_answer69)
  The value of \[\sin 10{}^\circ \sin 50{}^\circ \sin 70{}^\circ \] is equal to
  A)
   \[\frac{1}{2}\]
  done clear
  B)
   \[\frac{1}{4}\]
  done clear
  C)
   \[\frac{1}{8}\] 
  done clear
  D)
   \[\frac{1}{16}\]
  done clear
  View Answer play_arrow

• question_answer70)
  The value of\[\sin 20{}^\circ sin40{}^\circ \sin 60{}^\circ \sin 80{}^\circ \]is equal to
  A)
   \[\frac{1}{2}\]
  done clear
  B)
   \[\frac{3}{4}\]
  done clear
  C)
   \[\frac{1}{8}\]
  done clear
  D)
   \[\frac{3}{16}\]
  done clear
  View Answer play_arrow

• question_answer71)
  The value of \[\frac{\sin 38{}^\circ -\cos 68{}^\circ }{\cos 68{}^\circ +\sin 38{}^\circ }\]equal to
  A)
   \[\sqrt{3}\,\,\tan 8{}^\circ \]
  done clear
  B)
   \[\sqrt{3}\cot 8{}^\circ \]
  done clear
  C)
   \[\sqrt{3}\,\,\sin 38{}^\circ \]
  done clear
  D)
   \[\sqrt{3}\,\,\sin 8{}^\circ \]
  done clear
  View Answer play_arrow

• question_answer72)
  If \[\cos x=k\cos \,\,(x-2y)\] then \[\tan \,\,(x-y)\,\,\tan y\] is equal to
  A)
   \[\frac{1+k}{1-k}\]
  done clear
  B)
   \[\frac{1-k}{1+k}\]
  done clear
  C)
   \[\frac{2k}{k+1}\]
  done clear
  D)
   \[\frac{k-1}{2k+1}\]
  done clear
  View Answer play_arrow

• question_answer73)
  From the top of a pillar of height 20 m the angles of elevation and depression of the top and bottom of another pillar are \[30{}^\circ \] and \[45{}^\circ \]respectively. The height of the second pillar (in metres) is
  A)
   \[\frac{20}{\sqrt{3}}(\sqrt{3}-1)\]          
  done clear
  B)
   \[\frac{20}{\sqrt{3}}(\sqrt{3}+1)\]
  done clear
  C)
   \[20\sqrt{3}\]
  done clear
  D)
   \[\frac{20}{\sqrt{3}}\]
  done clear
  View Answer play_arrow

• question_answer74)
  In a \[\Delta ABC,\]if A is \[(2,-1)\]and x andare equations of an altitude and an angle bisector respectively, drawn from B, then equation of BC is
  A)
       
  done clear
  B)
   
  done clear
  C)
   
  done clear
  D)
   
  done clear
  View Answer play_arrow

• question_answer75)
  A triangle is formed by the lines  and If  and m very subject to the condition then the locus of its circumcentre is
  A)
                     
  done clear
  B)
   
  done clear
  C)
   
  done clear
  D)
   
  done clear
  View Answer play_arrow

• question_answer76)
  The average age of 12 players of a team is 25 yr. If the captain's age is included, the average age increases by 1 yr. The age of the captain is
  A)
   25 yr
  done clear
  B)
   38 yr
  done clear
  C)
   36 yr
  done clear
  D)
   26 yr
  done clear
  View Answer play_arrow

• question_answer77)
  If a sum of money is to be divided among A, B and C such that A's share is equal to twice B?s share and B?s share is 4 times C's share, then their shares are in the ratio
  A)
   1 : 2 : 4
  done clear
  B)
   1 : 4 : 1
  done clear
  C)
   8 : 4 : 1
  done clear
  D)
   2 : 4 : 1
  done clear
  View Answer play_arrow

• question_answer78)
  A can do a work in 6 days and B in 9 days. How many days will both take together to complete the work?
  A)
   7.5      
  done clear
  B)
   5.4
  done clear
  C)
   3.6
  done clear
  D)
   3
  done clear
  View Answer play_arrow

• question_answer79)
  The volumes of two spheres are in the ratio 8 :1 The ratio of their surface areas is
  A)
   4 : 9    
  done clear
  B)
   2 : 3
  done clear
  C)
   4 : 5    
  done clear
  D)
   5 : 6  
  done clear
  View Answer play_arrow

• question_answer80)
  A shopkeeper marks his sarees at 20% above a cost price and allows the purchaser a discount 10% for cash buying. What profit per cent does he make?
  A)
   18%    
  done clear
  B)
   12%
  done clear
  C)
   10%    
  done clear
  D)
   8%
  done clear
  View Answer play_arrow

• question_answer81)
  A person travels 600 km by train at 80 km/h, 800 km by ship at 40 km/h, 500 km by aeroplane 400 km/h and 100 km by car at 50 km/h. What is the average speed for the entire distance?
  A)
   \[65\frac{6}{123}\text{km/h}\]
  done clear
  B)
          60 km/h
  done clear
  C)
   \[60\frac{5}{123}\text{km/h}\]  
  done clear
  D)
   62 km/h     
  done clear
  View Answer play_arrow

• question_answer82)
                              A trademan marks his goods at 20% above the cost price. He allows his customers a discount of 8% on marked price. Find out his profit per cent.   
  A)
   12%
  done clear
  B)
   10.4%   
  done clear
  C)
   8.6%
  done clear
  D)
   8.2%  
  done clear
  View Answer play_arrow

• question_answer83)
         A shopkeeper purchased a chair marked at Rs. 800, at two successive discounts of 10% and 15% respectively. He spent Rs. 28 on transportation sold the chair for Rs. 800. His gain per cent is  
  A)
   40%
  done clear
  B)
   30%          
  done clear
  C)
   25%    
  done clear
  D)
   14% 
  done clear
  View Answer play_arrow

• question_answer84)
           The selling price of 5 articles is the same as the cost price of 3 articles. The gain or loss is 
  A)
           20% gain
  done clear
  B)
   25% gain     
  done clear
  C)
   33.33% loss
  done clear
  D)
   40% loss  
  done clear
  View Answer play_arrow

• question_answer85)
     If the length of a rectangle is increased by 25% the width is decreased by 20%, then the area of the rectangle 
  A)
                                  increases by 5%
  done clear
  B)
   decreases by 5%   
  done clear
  C)
                         remains unchanged
  done clear
  D)
   increases by 10% 
  done clear
  View Answer play_arrow

• question_answer86)
                       A particular job can be completed by a team of 10 men in 12 days. The same job can be completed by a team of 10 women in 6 days. How many days are needed to complete the job if the two teams work together?  
  A)
                         4        
  done clear
  B)
   6           
  done clear
  C)
   9
  done clear
  D)
   18   
  done clear
  View Answer play_arrow

• question_answer87)
         A book seller bought 200 text books for Rs.12000. He wanted to sell them at a profit so that he got 20 books free. At what profit per cent should he sell them?
  A)
   10%    
  done clear
  B)
   11%
  done clear
  C)
   11.5%
  done clear
  D)
   12%
  done clear
  View Answer play_arrow

• question_answer88)
  By selling an article for Rs. 480 a person lost 20%. For what should he sell it to make a profit of 20%?
  A)
   Rs. 800
  done clear
  B)
   Rs. 760
  done clear
  C)
   Rs. 720
  done clear
  D)
   Rs. 680
  done clear
  View Answer play_arrow

• question_answer89)
  In an examination a candidate must secure 40% marks to pass. A candidate, who gets 220 marks, fails by 20 marks. What are the maximum marks for the examination?   
  A)
                1200
  done clear
  B)
   800
  done clear
  C)
   600
  done clear
  D)
   450
  done clear
  View Answer play_arrow

• question_answer90)
  \[\sqrt{6+\sqrt{6+\sqrt{6}}}+...\]is equal to
  A)
   \[{{6}^{2/3}}\]          
  done clear
  B)
   6
  done clear
  C)
   \[3\frac{1}{2}\]
  done clear
  D)
   3
  done clear
  View Answer play_arrow

• question_answer91)
  The diameter of a toy wheel is 14 cm. What is the distance travelled by it in 15 revolutions?
  A)
   880 cm
  done clear
  B)
   660 cm
  done clear
  C)
   600 cm
  done clear
  D)
   560 cm
  done clear
  View Answer play_arrow

• question_answer92)
  A policeman started chasing a thief from a distance of 200 m from which point the thief started running to flee. The running rate of policeman and thief was 11 km/h and 10 km/h respectively. How far they are from each other after 6 min of start the running?
  A)
   50 m
  done clear
  B)
   100 m
  done clear
  C)
   150 m
  done clear
  D)
   200 m
  done clear
  View Answer play_arrow

• question_answer93)
  What is the difference between compound interests on Rs. 5000 for \[1\frac{1}{2}\text{yr}\] at 4% per annum according as the interest is compounded yearly or half-yearly?
  A)
   Rs. 2.04
  done clear
  B)
   Rs. 3.06
  done clear
  C)
   Rs. 8.30
  done clear
  D)
   Rs. 4.80
  done clear
  View Answer play_arrow

• question_answer94)

  Directions: In each of following number series a wrong is given. Find out that number.

  5, 10, 17, 27, 37, 50, 65
  A)
   37
  done clear
  B)
   50
  done clear
  C)
   10       
  done clear
  D)
   27
  done clear
  View Answer play_arrow

• question_answer95)

  Directions: In each of following number series a wrong is given. Find out that number.

  19, 68, 102, 129, 145, 154
  A)
   154
  done clear
  B)
   129
  done clear
  C)
   145
  done clear
  D)
   102
  done clear
  View Answer play_arrow

• question_answer96)

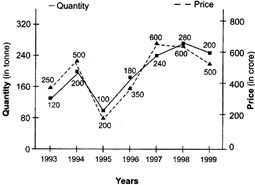
  Directions: Study the following graph carefully and answer the questions given below.

  The quantity and the price of pearls, produced by a company in given years

  How much percentage fall in the price in 1999 that of in 1998?
  A)
   \[12\frac{2}{3}\]
  done clear
  B)
   \[3\frac{1}{3}\]
  done clear
  C)
   20
  done clear
  D)
   162
  done clear
  View Answer play_arrow

• question_answer97)

  Directions: Study the following graph carefully and answer the questions given below.

  The quantity and the price of pearls, produced by a company in given years

  If per kg price of pearls in 1999 was equal to that of in 1997, what will be the total value in 1999?
  A)
   Rs. 650 crore    
  done clear
  B)
   Rs. 625 crore
  done clear
  C)
   Rs. 700 crore    
  done clear
  D)
   Rs. 725 crore
  done clear
  View Answer play_arrow

• question_answer98)

  Directions: Study the following graph carefully and answer the questions given below.

  The quantity and the price of pearls, produced by a company in given years

  In which of the following years the price per kg pearls was Rs. 2600?
  A)
   1996
  done clear
  B)
   1997
  done clear
  C)
   1994   
  done clear
  D)
   None of these
  done clear
  View Answer play_arrow

• question_answer99)

  Directions: Study the following graph carefully and answer the questions given below.

  The quantity and the price of pearls, produced by a company in given years

  In which of the following years price per tonne pearls was maximum?
  A)
   1993 and 1994
  done clear
  B)
   1995 and 1997
  done clear
  C)
   1994 and 1997
  done clear
  D)
   1997 and 1999
  done clear
  View Answer play_arrow

• question_answer100)

  Directions: Study the following graph carefully and answer the questions given below.

  The quantity and the price of pearls, produced by a company in given years

  What was the average approximate production of pearls?
  A)
   200 tonne
  done clear
  B)
   250 tonne
  done clear
  C)
   150 tonne
  done clear
  D)
   180 tonne
  done clear
  View Answer play_arrow