question_answer

Derive an expression for the potential energy of an electric dipole placed in a uniform electric field. Hence, discuss the conditions of stable and unstable equilibrium.

Or

Four charges are arranged at the corners of a square ABCD of side d as shown in the figure.

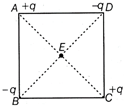A square ABCD of side d

(i) Find the work required to put together this arrangement.

(ii) A charge \[{{q}_{0}}\] is brought to the centre E of the square, the four charges being held fixed at its corners. How much extra work is needed to do this?

(iii) Compute the work done by the charges in rearranging this arrangement of to another similar square arrangement of the charges such that the side of the square now becomes 2d.

Answer:

            Let at any instant, dipole makes an angle 6 with the direction of electric field E. Two equal and opposite forces \[+\,qE\] and \[-\,qE\] act on the two point charges of dipole. 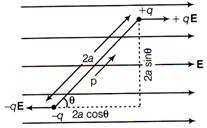            Torque on a dipole in These forces form couple whose torque \[(\tau )\] is given by \[\tau =\text{ }F\times \]perpendicular distances between forces \[\Rightarrow \tau =qE\times (2a\sin \theta )=[q\,(2a)]Esin\theta \] \[\Rightarrow \tau =pE\sin \theta \]                                                ?(i) where, p =q(2a) is electric dipole moment. If the dipole is rotated through a small angle \[d\theta \]against the torque, then small work done is given by \[dW=\tau d\theta \Rightarrow dW=(pE\sin \theta )d\theta \] The total work done in rotating the dipole from angle \[{{\theta }_{1}}\] to \[{{\theta }_{2}}\] with the direction of electric field E is given by             \[W=\int{dW=\int_{{{\theta }_{1}}}^{{{\theta }_{2}}}{pE\,\,\sin d\theta }}\] \[W=pE\,[-\cos \theta ]_{{{\theta }_{1}}}^{{{\theta }_{2}}}=pE[\cos {{\theta }_{1}}-\cos {{\theta }_{2}}]\] This work done is stored in the form of electrostatic potential energy. \[\therefore \] Electrostatic potential energy,             \[U=pE\,[\cos {{\theta }_{1}}-\cos {{\theta }_{2}}]\] If \[{{\theta }_{1}}=90{}^\circ \] and \[{{\theta }_{2}}=\theta \] \[\Rightarrow \]   \[U=pE\,(\cos \,90{}^\circ -\cos \theta )\]             \[=-pE\,\cos \theta =-p.E\]           \[[\because cos90{}^\circ =0]\] This is the required expression of potential energy of an electric dipole placed in uniform electric field. For stable equilibrium When    \[\theta =0{}^\circ \] Minimum potential energy \[({{U}_{\min }})=-pE\cos \theta \] \[=-pE\cos 0{}^\circ \] or,        \[{{U}_{\min }}=-pE\] The potential energy of an electric dipole is minimum and dipole attains equilibrium. For unstable equilibrium When    \[\theta =180{}^\circ \] Potential energy \[(U)=pE\,cos\theta =-pEcos180{}^\circ \]                        \[=-pE(-1)=pE\] Thus, the potential energy is maximum and in this situation dipole is said to be in unstable equilibrium. Thus, when dipole is parallel to electric field, then it is said to be in stable equilibrium and when dipole is anti-parallel, then it is referred as in unstable equilibrium. Or (i) Work done in making the system = - (Total electrostatic potential energy of the system) We have,           AB = BC = CD = DA = d and                   \[AC=BD=\sqrt{{{d}^{2}}+{{d}^{2}}}=\sqrt{2d}\] There will be six pairs (four sides and two diagonals) \[\therefore \]      \[U={{U}_{AB}}+{{U}_{BC}}+{{U}_{CD}}+{{U}_{DA}}+{{U}_{AC}}+{{U}_{BD}}\] \[\Rightarrow U=\frac{1}{4\pi {{\varepsilon }_{0}}}\left[ -\frac{{{q}^{2}}}{d}-\frac{{{q}^{2}}}{d}-\frac{{{q}^{2}}}{d}-\frac{{{q}^{2}}}{d}+\frac{{{q}^{2}}}{(\sqrt{2}d)}+\frac{{{q}^{2}}}{(\sqrt{2}d)} \right]\]\[\Rightarrow U=\frac{{{q}^{2}}}{4\pi {{\varepsilon }_{0}}d}[-\,4+\sqrt{2}]\] \[\Rightarrow U=-\frac{{{q}^{2}}}{4\pi {{\varepsilon }_{0}}d}[4-\sqrt{2}]\] Joule \[\therefore \] Work required to put together this arrangement \[W=-U=\frac{{{q}^{2}}}{4\pi {{\varepsilon }_{0}}d}[4-\sqrt{2}]\] Joule (ii) Extra work needed to bring charge \[{{q}_{0}}\] to centre \[EW={{q}_{0}}\times \]electrostatic potential at £ due to four charges \[={{q}_{0}}\left[ \frac{1}{4\pi {{\varepsilon }_{0}}}\frac{q}{\left( \frac{d}{\sqrt{2}} \right)}+\frac{1}{4\pi {{\varepsilon }_{0}}}\frac{-q}{\left( \frac{d}{\sqrt{2}} \right)}+\frac{1}{4\pi {{\varepsilon }_{0}}}\frac{q}{\left( \frac{d}{\sqrt{2}} \right)}+\frac{1}{4\pi {{\varepsilon }_{0}}}\frac{-q}{\left( \frac{d}{\sqrt{2}} \right)} \right]\]\[={{q}_{0}}\times 0=0\Rightarrow W=0\] Hence, no work Is required to bring any charge to centre E. (iii) Initial electrostatic potential energy of the square arrangement of charges \[{{U}_{i}}=U\] [from problem (a)] \[\therefore \] Electrostatic potantial energy of new arrangement of charges             \[{{U}_{f}}=\frac{-{{q}^{2}}}{4\pi {{\varepsilon }_{0}}(2d)}[4-\sqrt{2}]\,\,joule=\frac{U}{2}\] \[\therefore \] Required work done for rearrangement of charges             \[\Delta \,W={{U}_{f}}-{{U}_{i}}=\frac{U}{2}-U\] \[=-\frac{U}{2}=\frac{-{{q}^{2}}}{8\pi {{\varepsilon }_{0}}d}[4-\sqrt{2}]\] Joule