question_answer

A beam of protons with a velocity of \[4\times {{10}^{5}}\,m/s\]enters in a uniform magnetic field of\[3.0T\]. The velocity makes an angle of \[{{60}^{o}}\] with the magnetic field. The radius of the helical path taken by the proton beam, and the pitch of the helix, are

A) \[1.2cm\]and \[4.4cm~~\]

B)  \[1.4cm\]and \[4.2cm\]

C)  \[2.4cm\]and \[3.1cm\]

D)  None of these

Correct Answer: A

Solution :

The component of the proton's velocity along and perpendicular to the magnetic field are \[{{V}_{||}}=(4\times {{10}^{5}}m/s)\,\sin {{60}^{o}}\] \[=2\times {{10}^{5}}m/s\]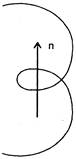 and           \[{{V}_{\bot }}=(4\times {{10}^{5}}m/s)\,\,sin{{60}^{o}}\]                 \[=2\sqrt{3}\times {{10}^{5}}m/s\] As the force \[qV\times B\] is perpendicular to the magnetic field, the component \[{{V}_{||}}\] will remain constant. In the plane perpendicular to the field, the proton will discribe a circle whose radius is obtained from the equation.                                 \[q{{V}_{\bot }}B=\frac{mV_{\bot }^{2}}{r}\] or         \[r=\frac{m{{V}_{||}}}{qB}\]                 \[=\frac{(1.67\times {{10}^{-27}}kg)\times (2\sqrt{3}\times {{10}^{5}}m/s)}{(1.6\times {{10}^{-19}}C)\times (0.3T)}\]                 \[=0.012=1.2cm\] The time taken in one complete revolution in the plane perpendicular to B is.                                 \[T=\frac{2\pi r}{{{V}_{\bot }}}=\frac{2\times 3.14\times 0.012m}{2\sqrt{3}\times {{10}^{5}}m/s}\] The distance moved along the field during this period i.e. the pitch.             \[=\frac{(2\times {{10}^{5}}m/s)\times 2\times 3.14\times 0.012m}{2\sqrt{3}\times {{10}^{5}}m/s}\]                 \[=0.044m=4.4cm\]