2. Sample Papers
  3. NEET

NEET Sample Paper NEET Sample Test Paper-21

  • question_answer
    A smooth uniform rod of length L and mass M has two identical beads of negligible size, each of mass m, which can slide freely along the rod. Initially, the two beads are at the center of the rod and the system is rotating with angular velocity\[{{\omega }_{0}}\]about an axis perpendicular to rod passing through the midpoint of the rod. There are no external forces. When the beads reach the ends of the rod the angular velocity of the system is:

    A) \[\frac{M{{\omega }_{0}}}{M+3m}\]                     

    B) \[\frac{M{{\omega }_{0}}}{M+6m}\]         

    C)   \[\frac{M+6m}{M}{{\omega }_{0}}\]         

    D)   \[{{\omega }_{0}}\]   

    Correct Answer: B

    Solution :

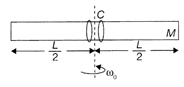
    Suppose, when the leads reach the ends, moment of inertia of the system changes from \[{{\text{I}}_{\text{1}}}\]to \[{{\text{I}}_{2}}\]an therefore, The angular velocity changes from \[{{\omega }_{1}}\]to\[{{\omega }_{2}}.\] as External torque acting on the system is zero, hence Angular momentum of the system is conserved. i.e., \[{{I}_{1}}{{\omega }_{1}}={{I}_{2}}{{\omega }_{2}}\]             \[[{{\omega }_{0}}={{\omega }_{1}}\,\text{here}]\] \[\left\{ \frac{M{{L}^{2}}}{12}+0 \right\}{{\omega }_{0}}=\left\{ \frac{M{{L}^{2}}}{12}+2m{{\left( \frac{L}{2} \right)}^{2}} \right\}{{\omega }_{2}}\] \[\therefore \]\[{{\omega }_{2}}=\left\{ \frac{M{{L}^{2}}/12}{\frac{M{{L}^{2}}}{12}+\frac{m{{L}^{2}}}{2}} \right\}{{\omega }_{0}}\]or \[{{\omega }_{2}}=\left\{ \frac{M}{M+6m} \right\}{{\omega }_{0}}\] Hence, the correction option is (b).


You need to login to perform this action.
You will be redirected in 3 sec spinner