question_answer

                         Find the area of the region bounded by a circle \[4{{x}^{2}}+4{{y}^{2}}=9\] and the parabola \[{{y}^{2}}=4x.\]

Answer:

Given equations of curves are             \[4{{x}^{2}}+4{{y}^{2}}=9\]                                   ?(i) and       \[{{y}^{2}}=4x\]                                   ?(ii) Here, Eq. (i) represents a circle with centre (0, 0) and radius \[\frac{3}{2}\] and Eq. (ii) represents a parabola, having vertex (0, 0) and axis is along X-axis. On putting \[{{y}^{2}}=4x\] from Eq, (ii) in Eq. (i), we get \[4{{x}^{2}}+16x-9=0\] \[\Rightarrow \]   \[4{{x}^{2}}+18x-2x-9=0\] \[\Rightarrow \]   \[2x(2x+9)-1(2x+9)=0\] \[\Rightarrow \]   \[2x(2x-1)(2x+9)=0\] \[\Rightarrow \] \[x=\frac{1}{2},-\frac{9}{2}\] When \[x=\frac{1}{2},\] then from Eq. (ii),             \[{{y}^{2}}=4\times \frac{1}{2}=2\] \[\Rightarrow \]   \[y=\pm \sqrt{2}\] and when \[x=-\frac{9}{2},\] Then, from Eq. (ii), \[{{y}^{2}}=4\times -\frac{9}{2},=-\,18\] which is not possible. 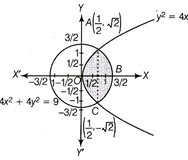              Hence, the points of intersection of given curves are \[\left( \frac{1}{2},\,\,\sqrt{2} \right)\] and \[\left( \frac{1}{2},\,\,-\sqrt{2} \right).\] Thus, the region bounded by given curves is OABCO, which is symmetrical about X-axis. \[\therefore \] Required area = Area of shaded region OABCO\[=2\times \] Area of region AOBA \[=2\left[ \int_{0}^{1/2}{{{y}_{(parabola)}}dx+\int_{1/2}^{3/2}{{{y}_{(circle)}}dx}} \right]\] \[=2\left[ \int_{0}^{1/2}{2\sqrt{x\,}dx+\int_{1/2}^{3/2}{\left( \sqrt{\frac{9}{4}-{{x}^{2}}} \right)dx}} \right]\] 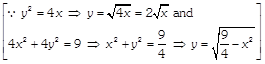\[=2\left[ 2\int_{0}^{1/2}{\sqrt{x\,\,}}dx+\int_{1/2}^{3/2}{\sqrt{\left( \frac{3}{2} \right)-{{x}^{2}}dx}} \right]\] \[=2\left[ \left[ 2\cdot {{x}^{3/2}}\cdot \frac{2}{3} \right]_{0}^{1/2}+\left[ \frac{x}{2}\sqrt{\frac{9}{4}-{{x}^{2}}}+\frac{9}{8}{{\sin }^{-1}}\frac{2x}{3} \right]_{1/2}^{3/2} \right]\]\[\left[ \because \,\,\,\int{\sqrt{{{a}^{2}}-{{x}^{2}}}dx=\frac{x}{2}\sqrt{{{a}^{2}}-{{x}^{2}}}+\frac{{{a}^{2}}}{2}{{\sin }^{-1}}\frac{x}{a}} \right]\] \[=2\left[ \frac{4}{3}\cdot \frac{1}{{{[{{(\sqrt{2})}^{2}}]}^{3/2}}}+\frac{9}{8}\cdot \frac{\pi }{2}-\frac{\sqrt{2}}{4}-\frac{9}{8}{{\sin }^{-1}}\frac{1}{3} \right]\]                                                 \[\left[ \because \,\,{{\sin }^{-1}}1=\frac{\pi }{2} \right]\] \[=2\left[ \frac{4}{3}\cdot \frac{1}{2\sqrt{2}}+\frac{9\pi }{16}-\frac{\sqrt{2}}{4}-\frac{9}{8}{{\sin }^{-1}}\frac{1}{3} \right]\] \[=2\left[ \frac{2}{3\sqrt{2}}+\frac{9\pi }{16}-\frac{\sqrt{2}}{4}-\frac{9}{8}{{\sin }^{-1}}\frac{1}{3} \right]\] \[=\frac{4}{3\sqrt{2}}+\frac{9\pi }{8}-\frac{\sqrt{2}}{2}-\frac{9}{4}{{\sin }^{-1}}\frac{1}{3}\] \[=\frac{4\sqrt{2}}{6}+\frac{9\pi }{8}-\frac{\sqrt{2}}{2}-\frac{9}{4}{{\sin }^{-1}}\frac{1}{3}\] \[=\frac{2\sqrt{2}}{3}+\frac{\sqrt{2}}{2}+\frac{9\pi }{8}-\frac{9}{4}{{\sin }^{-1}}\frac{1}{3}\] \[=\frac{\sqrt{2}}{6}+\frac{9\pi }{8}-\frac{9}{4}{{\sin }^{-1}}\frac{1}{3}\] Hence, required area is \[\left( \frac{\sqrt{2}}{6}+\frac{9\pi }{8}-\frac{9}{4}{{\sin }^{-1}}\frac{1}{3} \right)\] sq units.