| Find the volume of the largest cylinder that can be inscribed in sphere of radius r. |
| OR |
| A given quantity of metal is to be cast into a half cylinder with a rectangular base and semicircular ends. Show that in order that total surface area is minimum, the ratio of length of cylinder to the diameter of its semicircular ends is \[\pi :(\pi +2).\] |
Answer:
Given, r is the radius of sphere. Let R be the radius, h be the height of cylinder and V be the volume of the cylinder. Then, \[V=\pi {{R}^{2}}h\] ?(i) 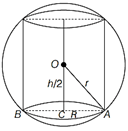
In right angled \[\Delta \,OAC,\]we have \[{{r}^{2}}={{R}^{2}}+{{\left( \frac{h}{2} \right)}^{2}}\] \[\Rightarrow \] \[{{R}^{2}}={{r}^{2}}-\frac{{{h}^{2}}}{4}\] \[\therefore \] \[V=\pi {{r}^{2}}h-\frac{\pi {{h}^{3}}}{4}\] \[\therefore \] \[\frac{dV}{dh}=\pi {{r}^{2}}-\frac{3\pi {{h}^{2}}}{4}\] Now, \[\frac{{{d}^{2}}V}{d{{h}^{2}}}=0-\frac{6\pi h}{4}=\frac{-\,3\pi h}{2}\] For maximum or minimum value of V, put \[\frac{dV}{dh}=0\] \[\Rightarrow \] \[\pi {{r}^{2}}-\frac{3\pi {{h}^{2}}}{4}=0\Rightarrow h=\frac{2}{\sqrt{3}}r\] Now, \[{{\left( \frac{{{d}^{2}}V}{d{{h}^{2}}} \right)}_{h=\frac{2}{\sqrt{3}}r}}=\frac{-\,3\pi }{2}\times \frac{2}{\sqrt{3}}r=-\,\sqrt{3}\pi r<0\] Thus, V is maximum when\[h=\frac{2}{\sqrt{3}}r\]. \[\therefore \]R is calculated as \[{{R}^{2}}={{r}^{2}}-\frac{{{h}^{2}}}{4}\] \[\Rightarrow \] \[{{R}^{2}}={{r}^{2}}-\frac{1}{4}\times {{\left( \frac{2}{\sqrt{3}}r \right)}^{2}}=\sqrt{\frac{2}{3}}r\] \[\therefore \]Maximum volume of the cylinder is given by \[{{V}_{\max }}=\pi {{R}^{2}}h=\pi {{\left( \sqrt{\frac{2}{3}}r \right)}^{2}}\left( \frac{2}{\sqrt{3}}r \right)=\frac{4\pi {{r}^{3}}}{3\sqrt{3}}cu\,\,units\] OR Let r be radius of semicircular end and h be the height of the half cylinder. Volume of half cylinder,\[V=\frac{1}{2}\pi {{r}^{2}}h;\] ?(i) Total surface area, \[S=\pi rh+\pi {{r}^{2}}+2rh\] \[S=(\pi +2)\,r\cdot \frac{2V}{\pi {{r}^{2}}}+\pi {{r}^{2}}=\frac{2V\,(\pi +2)}{\pi r}+\pi {{r}^{2}}\] [from Eq. (i)] \[\frac{dS}{dr}=\frac{-\,2V\,(\pi +2)}{\pi {{r}^{2}}}+2\pi r\] ?(ii) For minimum or minimum surface area, put \[\frac{dS}{dr}=0\] \[\Rightarrow \] \[\frac{2V\,(\pi +2)}{\pi {{r}^{2}}}=2\pi r\] ?(iii) 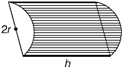
\[\frac{{{d}^{2}}S}{d{{r}^{2}}}=\frac{4V\,(\pi +2)}{\pi {{r}^{3}}}+2\pi >0\]for \[{{r}^{3}}=\frac{2V\,(\pi +2)}{2{{\pi }^{2}}}\] [from Eq. (iii)] \[\therefore \]S is minimum for \[\frac{V\,(\pi +2)}{\pi {{r}^{2}}}=\pi r\] [from Eq. (iii)] \[\Rightarrow \] \[\frac{\frac{1}{2}\pi {{r}^{2}}h\,(\pi +2)}{\pi {{r}^{2}}}=\pi r\] [from Eq. (i)] \[\Rightarrow \] \[\frac{h}{2r}=\frac{\pi }{\pi +2}\Rightarrow h:2r=\pi :\pi +2\] Hence proved.
You need to login to perform this action.
You will be redirected in
3 sec
