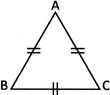
A) \[\frac{\text{AB}+\text{AC}}{2}=\text{BC}\]
B) \[\angle \text{BAC}+\angle \text{ACB}=120{}^\circ \]
C) \[\frac{\text{AB}}{\text{AC}}=\frac{\text{AC}}{\text{BC}}\]
D) \[\frac{\angle \text{BAC}+\angle \text{ACB}+\angle \text{ABC}}{2}=2\times 90{}^\circ \]
E) None of these
Correct Answer: D
Solution :
Explanation: Option (d) is correct. Since, \[\vartriangle \text{ABC}\] is an equilateral triangle, therefore, \[\angle \text{ABC}=\angle \text{ACB}=\angle \text{BAC}=60{}^\circ \] (each) and, AB = BC = AC = 1 unit (say) Now, in option (a); \[\frac{\text{AB+AC}}{2}=\frac{1\text{unit}+1\text{unit}}{2}\] \[=\frac{2\text{units}}{2}=1\text{unit=BC}\] In option (b); \[\angle \text{BAC}+\angle \text{ACB}=60{}^\circ +60{}^\circ =120{}^\circ \] In option (c); \[\frac{\text{AB}}{\text{BC}}=\frac{1\text{unit}}{1\text{unit}}=1;=\frac{\text{AC}}{\text{BC}}=\frac{1\text{unit}}{1\text{unit}}=1\] In option (d); \[\frac{\angle \text{BAC}+\angle \text{ACB}+\angle \text{ABC}}{2}\] \[=\frac{60{}^\circ +60{}^\circ +60{}^\circ }{2}=\frac{180{}^\circ }{2}=90{}^\circ \] \[=1\times 90{}^\circ =1\] right angle (not 2 right angles).You need to login to perform this action.
You will be redirected in
3 sec
