Specific Heat of Solids
Category : JEE Main & Advanced
When a solid is heated through a small range of temperature, its volume remains more or less constant. Therefore specific heat of a solid may be called its specific heat at constant volume \[{{C}_{V}}\].
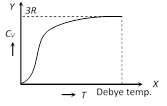
(1) From the graph it is clear that at \[T=0,\,\,{{C}_{V}}\] tends to zero
(2) With rise in temperature, \[{{C}_{V}}\] increases and at a particular temperature (called Debey's temperature) it becomes constant = 3R = 6 cal/mole \[\times \] kelvin = 25 J/mole \[\times \] kelvin
(3) For most of the solids, Debye temperature is close to room temperature.
(4) Dulong and Petit law : Average molar specific heat of all metals at room temperature is constant, being nearly equal to 3R = 6 cal. \[mol{{e}^{-1}}\,{{K}^{-1}}\] = 25 J \[mol{{e}^{-1}}\,{{K}^{-1}}\], where R is gas constant for one mole of the gas. This statement is known as Dulong and Petit law.
(5) Debey's law : It was observed that at very low temperature molar specific heat \[\propto {{T}^{3}}\] exception are Sn, Pb and Pt)
(6) Specific heat of ice : In C.G.S. \[{{c}_{\text{ice}}}=0.5\,\frac{cal}{gm\times {}^\circ C}\]
In S.I. \[{{c}_{ice}}==500\,\frac{cal}{kg\times {}^\circ C}=2100\,\frac{Joule}{kg\times {}^\circ C}\].
Specific heat of some solids at room temperature and atmospheric pressure
| Substance | Specific heat \[\mathbf{(J-k}{{\mathbf{g}}^{\mathbf{-1}}}\,{{\mathbf{K}}^{\mathbf{-1}}}\mathbf{)}\] | Molar specific heat \[\mathbf{(J-g}\,\,\mathbf{mol}{{\mathbf{e}}^{\mathbf{-1}}}\,{{\mathbf{K}}^{\mathbf{-1}}}\mathbf{)}\] |
| Aluminium | 900.0 | 24.4 |
| Copper | 386.4 | 24.5 |
| Silver | 236.1 | 25.5 |
| Lead | 127.7 | 26.5 |
| Tungsten | 134.4 | 24.9 |
You need to login to perform this action.
You will be redirected in
3 sec
