JEE Main & Advanced Physics Simple Harmonic Motion Various Formulae of S.H.M.

(1) S.H.M. of a liquid in U tube : If a liquid of density r contained in a vertical U tube performs S.H.M. in its two limbs. Then time period

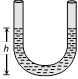
\[T=2\pi \sqrt{\frac{L}{2g}}\]\[=2\pi \sqrt{\frac{h}{g}}\]

where \[L=\] Total length of liquid column,

\[h=\] Height of undisturbed liquid in each limb \[(L-2h)\]

(2) S.H.M. of a floating cylinder : If \[l\] is the length of cylinder dipping in liquid then

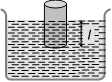
Time period  \[T=2\pi \sqrt{\frac{l}{g}}\]

(3) S.H.M. of a small ball rolling down in hemi-spherical bowl

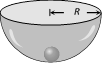
\[T=2\pi \sqrt{\frac{R-r}{g}}\]

\[R=\] Radius of the bowl

\[r=\]Radius of the ball

(4) S.H.M. of a piston in a cylinder

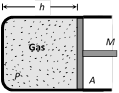
\[T=2\pi \sqrt{\frac{Mh}{PA}}\]

\[M=\] mass of the piston

\[A=\] area of cross section

\[h=\] height of cylinder

\[P=\] pressure in a cylinder

(5) S.H.M. of a body in a tunnel dug along any chord of earth

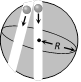
\[T=2\pi \sqrt{\frac{R}{g}}\]= 84.6 minutes

(6) Torsional pendulum : In a torsional pendulum an object is suspended from a wire. If such a wire is twisted, due to elasticity it exert a restoring toque \[\tau =C\theta \]. 

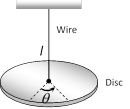
In this case time period is given by

\[T=2\pi \sqrt{\frac{I}{C}}\]

where \[l=\] Moment of inertia a disc

\[C=\] Torsional constant of wire \[=\frac{\pi \eta {{r}^{4}}}{2l}\]

\[\eta =\] Modulus of elasticity of wire and

\[r=\]Radius of wire

(7) Longitudinal oscillations of an elastic wire : Wire/string pulled a distance \[\Delta l\] and left. It executes longitudinal oscillations. Restoring force \[F=-\,AY\,\left( \frac{\Delta l}{l} \right)\]

\[Y=\]Young's modulus

\[A=\] Area of cross-section

Hence \[T=2\pi \sqrt{\frac{m}{k}}=2\pi \sqrt{\frac{ml}{AY}}\]