Electricity
Category : Banking
Introduction
Charge is something associated with matter due to which it produces and experiences electric and magnetic effects. The study of charges at rest is called static electricity or electrostatics while the study of charges in motion is called current electricity. There are two types of electric charge:
(i) Positive charge and (ii) Negative charge. The magnitude of elementary positive or negative charge is same and is equal to. \[1.6\times {{10}^{-19}}C\] Charge is a scalar quantity its SI unit is ampere second or coulomb.
(1) Similar charges repel and opposite charges attract.
(2) A charged body attracts light uncharged bodies.
(3) Accelerated charge radiates energy.
The materials which allow electric charge (or electricity) to flow freely through them are called conductors. Metals are very good conductors of electric charge. Silver, copper and aluminium are some of tile good conductors of electricity. The materials which do not allow electric charge to flow through them are called nonconductors or insulators. For example, most plastics, rubber, non-metals (except graphite), dry wood, wax, mica, porcelain, dry air etc., are insulators.
It states that, the electrostatic force of interaction (repulsion or attraction) between two electric charges \[{{q}_{1}}\] and \[{{q}_{2}}\]separated by a distance r, is directly proportional to the product of the charges and inversely proportional to the square of distance between them.
\[F\propto {{q}_{1}}{{q}_{2}}\] and \[F\propto 1/{{r}^{2}}\] or \[F=k\frac{{{q}_{1}}{{q}_{2}}}{{{r}^{2}}}\]
\[K=\frac{1}{4\pi {{\varepsilon }_{0}}}\]\[=9\times {{10}^{9}}\frac{N{{m}^{2}}}{cou{{l}^{2}}}\Rightarrow {{\varepsilon }_{0}}=8.85\times {{10}^{-12}}\frac{cou{{l}^{2}}}{N{{m}^{2}}}\]
²
Electric Field
An electric line of force is that imaginary smooth curve drawn in an electric field along which a free isolated unit positive charge moves. Two lines offeree never intersect. If they are assumed to intersect, there will be two directions of electric field at the point of intersection, which is impossible.
The total number of electric lines of force through a given area is called the electric flux.
(a) For open surface, \[{{\phi }_{0}}=\int{d\phi =\int{\overrightarrow{E}.d\overrightarrow{s}}}\]
(b) For closed surface, \[{{\phi }_{0}}=\oint{\overrightarrow{E}.d\overrightarrow{s}}\]
The total electric flux linked with a closed surface is \[\left( \frac{1}{{{\varepsilon }_{0}}} \right)\] times the charge enclosed by the closed surface (Gaussian surface), i.e. \[\oint{\overrightarrow{E}.d\overrightarrow{s}=\frac{q}{{{\varepsilon }_{0}}}}\]
Potential at a point can be physically interpreted as the work done by the field in displacing a unit + ve charge from some reference point to the given point.
i.e., \[V=\frac{w}{{{q}_{0}}}\]
\[V=-\int\limits_{\infty }^{r}{\overrightarrow{E}.d\overrightarrow{s}}\] i.e. \[E=-\frac{dv}{dr}\]
It is a scalar quantity.
Its dimensions: \[[M{{L}^{2}}{{T}^{-3}}{{A}^{-1}}]\].
Its SI unit is volt or joule coulomb\[^{-1}\].
Equipotential Surfaces
For a given charge distribution, locus of all points having same potential is called equipotential surfaces.
Capacitors and Capacitance
The symbol of a capacitor .
The net charge on a capacitor is zero.
Capacitance or capacity of a capacitor is a measure of ability of the capacitor to store charge on it. When a conductor is charged then its potential rises. The increase in potential is directly proportional to the charge given to the conductor. i.e., \[Q\propto V\]or \[Q=CV\]or\[C=\frac{Q}{V}\] The constant C is known as the capacitance of the conductor. Its SI unit is farad (F) or coulomb/volt.
(i) Size of conductor
(ii) Surrounding medium
(iii) Presence of other conductors nearby
In series: \[\frac{1}{{{C}_{eq}}}=\frac{1}{{{C}_{1}}}+\frac{1}{{{C}_{2}}}+...+\frac{1}{{{C}_{n}}}\]
In parallel: \[{{C}_{eq}}={{C}_{1}}+{{C}_{2}}+....{{C}_{n}}\]
R.J. Van de Graff in 1931 designed an electrostatic generator capable of generating very high potential of the order of \[5\times {{10}^{6}}\] V, which was then made use of an accelerating charged particles so as to carry out nuclear reactions.
Principle: It is based on the following two electrostatic phenomena
(i) The electric discharge takes place in air or gases readily at pointed conductors.
(ii) If a hollow conductor is in contact with an-other conductor, then as charge is supplied to the conductor, the hollow conductor continues accepting the charge irrespective of the fact, howsoever large its potential may grow.
Electric Current
The time rate of flow of charge through any cross-section is called electric current. If \[\Delta q\]charge passes through a cross-section in time\[\Delta t\] then, average current \[{{I}_{av}}=\frac{\Delta q}{\Delta t}\]
Instantaneous current \[I=\underset{\Delta t\to 0}{\mathop{\lim }}\,\frac{\Delta q}{\Delta t}=\frac{dq}{dt}\]
Electric current is measured in ampere (A).
(a) Direct current: The current whose magnitude and direction does not vary with time is called direct current (dc). The various sources are cells, dc dynamo, etc. It’s symbol is
(b) Alternating current: The current whose magnitude continuously changes with time and periodically changes its direction is called alternating current. It has constant amplitude and has alternate positive and negative halves. It is produced by ac dynamo. It's symbol is
\[R\propto L\]and \[R\propto \frac{1}{A}\]so, \[R\propto \frac{1}{A}\] or \[R=\rho \frac{L}{A}\]
where L = length, A= area of cross-section of wire and p is called resistivity or specific resistance. The reciprocal of specific resistance is conductance i.e. \[\sigma =\frac{1}{\rho }\]
At a very low temperature, the resistance of the conductor may vanish completely. When it happens, the conductor is called a superconductor. For example, helium is a super conductor at 4.2K (-268.8°C).
It states that if the physical state i.e. temperature, nature of material and dimensions of a conductor remain unchanged then the ratio of potential difference applied across its ends to current flowing through it remains constant.
i.e., \[V\propto I\]or V =I R, where R = \[\frac{V}{I}\]is the resistance of conductor.
Resistances are said to be connected in series between two points if they provide only a single path between two points. \[{{R}_{s}}={{R}_{1}}+{{R}_{2}}+{{R}_{3}}+....+{{R}_{n}}\]
Resistances are said to be connected in parallel between two points, if it is possible to proceed from one point to another along different paths.
\[\frac{1}{{{R}_{p}}}=\frac{1}{{{R}_{1}}}+\frac{1}{{{R}_{2}}}+\frac{1}{{{R}_{3}}}+....+\frac{1}{{{R}_{n}}}\]
When a current is passed through a resistor energy is wasted in overcoming the resistance of the wire. This energy is converted into heat. The heat generated (in joule) when a current of I ampere flows through a resistance of R ohm for T second is given by:
\[H={{I}^{2}}RT=VIt=\frac{{{V}^{2}}}{R}t\]\[joule=\frac{{{I}^{2}}RT}{4.2}calorie\]
1 unit of electrical energy = 1 Kilowatt hour (1 KWh) = \[3.6\times {{10}^{6}}\]joule.
This is known as Board of trade (B.O.T) unit of electrical energy. Energy liberated per second is called its power. The electrical power P delivered or consumed by an electrical device is given by P = VI, where V = Potential difference across the device and I = current.
An ammeter is a low resistance galvanometer used to measure strength of current in an electrical circuit. An ammeter is always connected in series in a circuit because, when an ammeter is connected in series it does not appreciably change the resistance of circuit and hence the main current flowing through the circuit.
Conversion of galvanometer into ammeter:
A galvanometer can be converted to an ammeter by connecting a low resistance or shunt in parallel to coil of galvanometer.
A voltmeter is a high resistance galvanometer used to measure potential difference. A voltmeter is connected in parallel to a circuit element because, when connected in parallel it draws least current from the main current. So it measures nearly accurate potential difference.
Conversion of galvanometer into voltmeter:
A galvanometer is converted to a voltmeter by connecting a high resistance in series with the coil of galvanometer.
When an alternating voltage is applied across a coil or a bulb, it sends a similar varying current (i. e., of the same nature as that of voltage) through the coil. The current is called alternating current (A.C.). The current flowing in only one direction in a circuit is called direct current (D.C.). Batteries, thermocouples and solar cells are some of the sources of direct current.
(i) A.C. can be obtained over a wide range of voltages. These voltages can be easily stepped up or stepped down with the help of transformers.
(ii) The generation of A.C. is found to be economical than that of D.C.
(iii) Alternating current can be controlled by using a choke coil without any significant wastage of electrical energy.
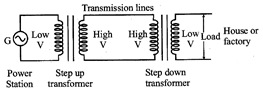
(iv) Alternating current may be transmitted at a high voltage from the power house to any place where it can again be brought down to low voltage. The cost in such a transmission is low and energy losses are minimized. Transformers cannot be used for D.C. Hence the cost of D.C. transmission from one place to other is quite high.
(v) A.C. equipments such as electric motors etc are more durable and convenient as compared to D.C. equipments.
Transformers
It is a device used for transforming a low alternating voltage of high current into a high alternating voltage of low current and vice versa, without increasing power or changing frequency.
If a high voltage is to be transformed into a low voltage, then the number of turns to secondary is less than those in primary. The transformer is called a step-down transformer. Transformation ratio of the transformer,
\[K=\frac{Number\,\,of\,\,turns\,\,in\,\,\sec \,\,ondary({{N}_{s}})}{Number\,\,of\,\,turns\,\,\,in\,\,primary({{N}_{p}})}\]
K > 1, for step-up transformer.
K< 1, for step-down transformer.
input power = output power
\[{{E}_{p}}\times {{I}_{p}}={{E}_{s}}\times {{I}_{s}}\]or, \[\frac{{{I}_{p}}}{{{I}_{s}}}=\frac{{{E}_{s}}}{{{E}_{p}}}=\frac{{{N}_{s}}}{{{N}_{p}}}\]
A transformer is used in almost all ac operation.
(i) In voltage regulators for TV, refrigerator, computer, air conditioner etc.
(ii) In the induction furnaces.
(iii) Step down transformer is used for welding purposes.
(iv) In the transmission of ac over long distance.
(v) Step down and step up transformers are used in electrical power distribution.
(vi) Audio frequency transformers are used in radiography, television, radio, telephone etc.
(vii) Radio frequency transformers are used in radio communication.
Faraday gave two laws of electromagnetic induction.
According to Lenz's law, the direction of the induced current is such that it opposes the change in the magnetic flux that causes the induced current ore. m. f. i.e., induced current tries to maintain flux. On combining Lenz's law with Faraday's laws \[e=-\frac{d\phi }{dt}\] The Lena's law is consistent with the law of conservation of energy.
The induced circulating current produced in a metal itself due to change in magnetic flux linked with the metal are called eddy current. The direction of eddy currents is given by Lenz's law.
(1) Dead beat galvanometer. (2) Energy meter.
(3) Speedometer. (4) Electric brakes.
(5) Single phase AC motor. (6) Induction furnace.
Production of induced e. m. f. in a coil due to the changes in current in the same coil, is called self-induction. The magnetic flux (\[\phi \]) linked with the coil is directly proportional to the current (I) flowing through it.
i.e. \[\phi \propto I\] \[\therefore \,\,\,\phi =LI\]
The constant L is called coefficient of self-induction or self-inductance of the coil. The S.I. unit of self-inductance or inductance is henry (H).
\[L=\frac{{{\mu }_{0}}{{N}^{2}}A}{l}\]
Factors on which self-inductance depends: If no iron or similar material is nearby, then the value of self-inductance depends only on the geometrical factors (length, cross- sectional area, number of turns and magnetic permeability of free space).
Production of induced e. m. f. in a coil due to the changes of current in a neighboring coil, is called mutual induction. Coefficient of mutual induction or mutual inductance: Let \[{{\phi }_{s}}\]= magnetic flux linked with the secondary coil when a current \[{{I}_{p}}\]flows through the primary coil.
Then, \[{{\phi }_{s}}\propto {{I}_{p}}\]or\[{{\phi }_{s}}=M{{I}_{p}}\] ......(1)
M = constant of proportionality called mutual inductance or coefficient of mutual induction.
An electrical machine used to convert mechanical energy into electrical energy is knownm as AC generator/alternator or dynamo.
A D. C. motor converts direct current energy from a battery into mechanical energy of rotation.
\[\eta =\frac{EI}{VI}=\frac{E}{V}=\frac{Back\,\,e.m.f.}{Applied\,\,e.m.f}\]
You need to login to perform this action.
You will be redirected in
3 sec
