6th Class Mathematics Related to Competitive Exam VENN DIAGRAM

Learning Objective

To understand how to solve the problems based on venn diagram.

INTRODUCTION:

The main aim of this section is to test the students' ability about the relation between some items of a group by diagrams. In these questions some figures of circles and some words are given. You have to choose a figure which represents the given words.

Example 1:

If all the words are of different groups then they will be shown by the diagram as given below.

1. Dog, Cow, Horse

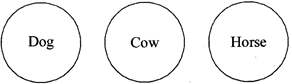
All these three are animals but of different groups; there is no relation between them. So they will be represented by three separate circles.

Example 2:

If the first word is related to the second and second word is related to the third, then they will be shown as

e.g. Units, Tens, Hundreds

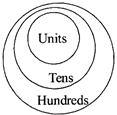
Ten units together make one Ten or in one Ten, ten whole units are available and ten tens together make one hundred.

Example 3:                                                                                      

If two different items are completely related to the third item but not to each other, they will be shown as below.

e.g. Pen, Pencil, Stationery.

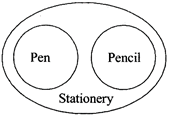
Example 4:

If there is some relation between two items and these items are completely related a third item, they will be shown as given below

e.g. Women, Sisters, Mothers.

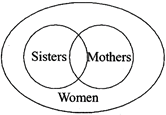
Some sisters may be mothers and vice-versa. Similarly some mothers may not be sisters and vice-versa.

But all sisters and all mothers belong to the women group.

Examples 5:

Two items are related to a third item to some extent but not completely and first two items are totally different.

e.g. Students, Boys, Girls.

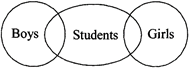
The boys and girls are different items while some boys may be students. Similarly among girls, some may be students.

Example 6:

All the three items are related to one another but to some extent, not completely.

e.g. Boys, Students, Athletes.

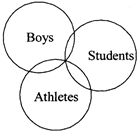
Some boys may be students and vice-versa. Similarly some boys may be athletes and vice-versa. Some students may be athletes and vice-versa.

Example 7:

In a town, 65% people read HT, 40% read ET and 25% read both HT and ET. What percentage of the people neither read HT nor ET?

(a) 50%                                 (b) 10%             

(c) 15%                                 (d) 20%

(d) Let the total number of people be 100. Let circle A but not to each other, represent people who read HT and circle B represent people who read ET.

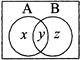
Then x + y = 65 y + z = 40 y = 25

We get x = 40 y = 25 z = 15.

Then the number of people who neither read HT nor ET.

= 100-(x + y + z)

= 100-(40 + 25 +15)

= 100 – 80 = 20

Therefore, the required percentage is 20%.