ARITHMETIC
Category : 6th Class
Learning Objective
FRACTION
Fraction is a method for representing the parts of a whole number. In the fraction, \[\frac{a}{b},\] a is the numerator and b is the denominator.
Example: \[\frac{2}{3},\,\frac{7}{8},\,\frac{3}{7},\,\frac{4}{9}]\ etc.
TYPES OF FRACTION
PROPER FRACTIONS
In a proper fraction, the numerator is always smaller than the denominator.
For example: \[\frac{1}{4},\,\frac{3}{5}\] etc.
IMPROPER FRACTIONS
In an improper fraction the numerator is greater than the denominator.
For example: \[\frac{5}{3},\,\frac{7}{4}\] is
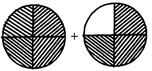
Fractions in the form of \[1\frac{1}{4}\] or \[2\frac{1}{2}\] are know as mixed fractions.
Let us represent mixed fraction by using figures.
EQUIVALENT FRACTIONS
Equivalent fractions represent same part of the whole.
For example \[\frac{1}{2}\,=\frac{2}{4}\,=\frac{3}{6}\,=\frac{4}{8}\,=\frac{5}{10}\]
We can find more equivalent fractions by multiplying or dividing the numerator and the denominator by the same number.
SIMPLEST FORM OF A FRACTION
A fraction is said to be in the simplest form (or lowest form) if its numerator and denominator have no common factor except 1.
The easiest way to find the simplest form of a fraction is to divide the numerator and denominator by their HCF.
For example: To reduce \[\frac{125}{225},\] find their HCF.
HCF of 125 and 225 =25
\[\therefore \,\,\,\,\,\,\frac{125}{225}\div \frac{25}{25}=\frac{5}{9}\] simplest form
To reduce \[\frac{36}{72}\]
H.C.F of 36 and 72 = 36
\[\therefore \,\,\,\,\,\frac{36}{72}\div \frac{36}{36}=\frac{1}{2}\] simplest form.
LIKE FRACTIONS
Fractions with the same denominator are called like fractions.
\[\frac{4}{13},\,\frac{3}{13},\,\frac{12}{13},\,\frac{9}{13}\] are examples of like fractions.
UNLIKE FRACTIONS
Fractions like \[\frac{1}{5},\,\frac{2}{3},\,\frac{3}{4}\] have different denominators are called unlike fractions.
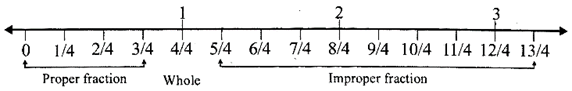
Fraction on the number line
Let us draw a number line and mark \[\frac{3}{4}\] on it. \[\frac{3}{4}\] is greater than 0 and less that 1. As \[\frac{3}{4}\] means 3 part out of 4, we will divide the gap between 0 and 1 into four equal parts, and mark \[\frac{1}{4},\,\frac{2}{4},\,\frac{3}{4},\,\frac{4}{4}(=1)\] as shown below.
![]()
COMPARING FRACTIONS
COMPARING LIKE FRACTIONS
To compare like fractions like \[\frac{2}{5},\,\frac{7}{5},\,\frac{1}{5},\,\frac{3}{5},\,\frac{9}{5}\] we compare the numerators only \[1<2<3<7<9\]
\[\frac{1}{5},\,\frac{2}{5},\,\frac{3}{5},\,\frac{7}{5},\,\frac{9}{5}\]
To compare unlike fractions like \[\frac{2}{3},\,\frac{3}{4},\,\frac{5}{7}\]. We must first convert then to like fraction as follows:
(i) Find the L.C.M of denominators 3, 4 and 7 i.e., L.C.M of 3, 4 and 7 is 84.
(ii) Make each denominator 84.
\[\frac{2\times 28}{3\times 28}=\frac{56}{84}\]
\[\frac{3\times 21}{4\times 21}\,=\frac{63}{84}\]
\[\frac{5\times 12}{7\times 12}\,=\frac{60}{84}\]
(iii) Now, we compare the numerators of these like fractions
\[\frac{56}{84},\,\frac{63}{84},\,\frac{60}{84}\]
\[\Rightarrow \,\,\frac{56}{84},\,\frac{60}{84},\,\frac{63}{84}\]
i.e., \[\frac{2}{3},\,\frac{5}{7},\,\frac{3}{4}\]
ADDITION AND SUBTRACTION OF FRACTIONS
ADDITION AND SUBTRACTION OF LIKE FRACTIONS
Addition and subtraction of like fraction is very simple as they have same denominator.
For example:
\[\frac{2}{10}+\frac{9}{10}=\frac{11}{10}\] (add the numerators)
\[\frac{9}{11}-\frac{5}{11}=\frac{4}{11}\] (subtract the numerators)
ADDITION AND SUBTRACTION OF UNLIKE FRACTIONS
To add or subtract unlike fractions, we should first find their equivalent fractions with the same denominator
For example:
To add \[\frac{3}{2}\] and \[\frac{4}{3},\] we find the LCM of the denominators 2 and 3 which is 6
Thus \[\frac{3}{2}\times \frac{3}{3}=\frac{9}{6}\] (add the numerators)
Similarly, to subtract \[\frac{1}{3}\] from \[\frac{8}{7}\] i.e., \[\frac{8}{7}-\frac{1}{3}\]
LCM of 7 and 3 =21
\[\frac{8}{7}\times \frac{3}{3}\,=\frac{24}{21}\] and \[\frac{1}{3}\,\times \frac{7}{7}=\frac{7}{21}\]
Thus \[\frac{24}{21}\,-\frac{7}{21}\,=\frac{17}{21}\] (subtract the numerators)
ADDITION AND SUBTRACTION OF MIXED FRACTIONS
Method I: Convert mixed fraction into improper fraction and add as in the case of unlike fraction.
For example: Add: \[3\frac{3}{5}\] and \[2\frac{5}{6}\]
\[3\frac{3}{5}+2\frac{5}{6}=\frac{18}{5}+\frac{17}{6}=\frac{18\times 6}{5\times 6}\,+\frac{17\times 5}{6\times 5}\]
\[=\frac{108}{30}+\frac{85}{30}\] (L.C.M of 5, 6 is 30)
\[\frac{193}{30}=6\frac{13}{30}\]
Subtract: \[3\frac{1}{4}-1\frac{1}{6}\]
\[3\frac{1}{4}\,-1\frac{1}{6}\,=\frac{13}{4}-\frac{7}{6}\]
\[=\frac{13\times 3}{4\times 3}-\frac{7\times 2}{6\times 2}\]
\[=\frac{39}{12}-\frac{14}{12}\]
\[=\frac{25}{12}=2\,\frac{1}{12}\]
Method II
The other method is to add the whole parts and proper fractions separately.
For example:
Add: \[3\frac{3}{5}+2\frac{5}{6}\]
\[3\frac{3}{5}+2\frac{5}{6}\,=3+2+\frac{3}{5}+\frac{5}{6}\,=5+\frac{3}{5}+\frac{5}{6}\]
Now,
\[\frac{3}{5}+\frac{5}{6}\,=\frac{3\times 6}{5\times 6}\,+\frac{5\times 5}{\,6\times 5}\,=\frac{18}{30}+\frac{25}{30}=\frac{43}{30}\,=1\frac{13}{30}\]
\[\therefore \,\,3+2+\frac{3}{5}+\frac{5}{6}\,=5+1\frac{13}{30}\,=5+1+\frac{13}{30}\,=6\frac{13}{30}\]
Subtract: \[3\frac{1}{4}\,-1\frac{1}{6}\]
\[3\frac{1}{4}\,-1\frac{1}{6}=3-1+\frac{1}{4}-\frac{1}{6}\]
Consider, \[\frac{1}{4}-\frac{1}{6}=\frac{1\times 3}{4\times 3}-\frac{1\times 2}{6\times 2}\]
\[=\frac{3}{12}-\frac{2}{12}=\frac{1}{12}\]
\[\therefore \,\,3-1\,+\frac{1}{4}\,-\frac{1}{6}\,=2+\frac{1}{12}=2\frac{1}{12}\]
DECIMALS
Decimal numbers or simply decimals are the fractions with denominators 10, 100, 1000 etc.
For example: \[\frac{7}{10},\,\frac{15}{100},\,\frac{21}{1000}\] etc.
The number before the decimal point is called the whole part or integral part, whereas the number after the decimal point is called the decimal part.
For example: In 12.73
Whole part is 12 and decimal part is 73
We read 12.73 as twelve point seven three.
LIKE DECIMALS
Like decimals have an equal number of digits to the right of the decimal point.
For example:
\[13.\] and \[4.\] are like decimals.
UNLIKE DECIMALS
The decimals having the different number of decimal places are called unlike decimals.
For example:
\[2.\] \[1.\] \[2.\]
REPRESENTATION OF DECIMALS ON THE NUMBER LINE
REPRESENTATION OF 4.2 ON THE NUMBER LINE
Clearly 4.2 lies between 4 and 5 in Fig. (i).
Take a magnified look of the line segment between 4 and 5 and divide it into 10 equal parts and mark each point of division between 4 and 5 as shown in the Fig. (ii).
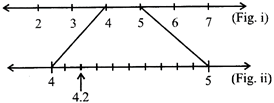
We can see in Fig. (ii), 4.2 is represented by the second mark of division after 4 in between 4 and 5.
FRACTIONS AS DECIMALS
To express given fraction into decimals, we follow the following steps:
Case I: Fractions whose denominators are of 10, 100, 1000 etc.
Step (i) Count the number of zeroes in denominator.
Step (ii) Place the decimal point in numerator so that the number of digits on right of decimal point becomes equal to the number of zeroes in denominator.
Step (iii) In case the number of digits in numerator is less than the number of zeroes in denominator, we place zero just right to decimal.
For example: In \[\frac{14}{1000},\] number of zeros in denominator is 3
So, \[\frac{14}{1000}=0.014\]
Case II: Fractions whose denominators are not 10, 100, 1000 etc.
Divide the numerator by the denominator and write the quotient in decimal form.
DECIMALS AS FRACTION
(i) To express the given decimals into fraction, we follow the following steps;
Step (i) Write the decimal without the decimal point as the numerator of the fraction.
Step (ii) Write the denominator of the fraction by inserting as many zeros on the right of 1 as the number of decimal places in the given decimals.
Step (iii) Simplify the fraction and write the fraction in the lowest form.
For example:
Express 0.038 as fraction \[0.038\,=\frac{38}{1000}\,=\frac{19}{500}\]
COMPARING DECIMALS
TO COMPARE LIKE DECIMALS
To compare like decimals follow these steps.
For example:
To compare 0.275, 2.34, 4.67, 2.24,4.67 > 2.34 > 2.24 > 0.275.
TO COMPARE UNLIKE DECIMALS
Like decimals and follow the steps to compare like decimals.
For example: To compare 4.2 and 4.27 first convert them to like decimals 4.20 and 4.27, 4.27 > 4.20 (0. 7 > 0)
USES OF DECIMALS
(I) IN MONEY VALUE
We know that 100 paise = Rs 1
Therefore, 1 paisa \[=\frac{1}{100}=\text{Rs}\,0.01\]
So 45paisa \[=\frac{45}{100}=\text{Rs}\,0.45\]
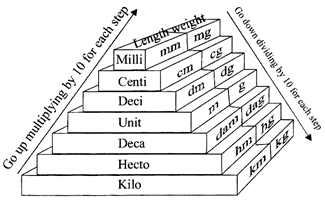
(II) IN MEASURES OF LENGTH
1km = 1000m \[1m\,=\frac{1}{1000}km\]
1m = 100 cm \[1cm=\frac{1}{100}m\]
1dm =10 cm \[1cm=\frac{1}{10}\,dm\]
1 cm = 10 mm \[1mm=\frac{1}{10}\,cm\]
For example:
\[7m=\frac{7}{1000}\,=0.007km\]
(III) IN MEASURES OF WEIGHT
Same as discussed in length (above) just use 'g' instead of 'm'.
1kg = 1000gm
1gm = 1000mg
i.e., \[1\,g\,=\frac{1}{1000}\,kg\]
\[1mg\,=\frac{1}{1000}\,gm\]
For example: \[7gm\,=\frac{7}{1000}\,kg\,=0.007\,gm\]
OPERATIONS OF DECIMALS
Addition of decimals
Step (i) Convert the given decimals to like decimals.
Step (ii) Write the decimals in columns with the decimal points directly below each other so that tenths come under tenths hundredths come under hundredths and so on.
Step (iii) Add as we add whole numbers, starting from right.
Step (iv) Place the decimal point in the answer directly below the other decimal points.
For Example: Add 12.73, 4.7, 1.074
12.730
+ 4.700
+ 1.074
_______
18.504
_______
Add 9.23, 4.75, 8.1
9.23
+ 4.75
+ 8.10
______
22.08
______
SUBTRACTION OF DECIMALS
We may follow the following steps to subtract a decimal number from another decimal number.
Step (i) Convert the given decimals to like decimals.
Step (ii) Write the decimals in columns with decimal points directly below each other.
Step (iii) Subtract as we subtract whole numbers starting from right.
Step (iv) Place the decimal point in the difference directly below the other decimal points.
For example: Subtract 10.205 from 20.05
20.050
- 10.250
_______
9.800
_______
RATIO
Ratio: If a and \[b(b\ne 0)\] are two quantities of the same kind, then the fraction a- is called the ratio of a to b.
COMPARISON OF RATIOS
To compare two given ratios, we follow the following steps:
(i) Express the given ratio in the form of a fraction.
(ii) Convert the fraction in its simplest form.
(iii) Find the L.C.M of the denominators of the simplest form of the fraction obtained in above step.
(iv) Divide the L.C.M obtained in step (iii) by the donominator of first fraction to get a number a (say).
Now, multiply the numerator and denominator of the fraction by x. Apply the same procedure for other fraction.
(v) Compare the numerators of the fractions obtained in step (v).
(vi) Having the same denominators.
The fraction having larger numerator will be larger than the other.
For example:
Compare the ratios 7 : 12 and 5:8
we have, \[7:12\,=\frac{7}{12}\] and \[5:8=\frac{5}{8}\]
(given ratios are in their simplest form)
Now, L.C.M. of 12 and 8 is 24.
Making the denominators equal to 24 of each fraction, we have
\[\frac{7\times 2}{12\times 2}\,=\frac{14}{24}\,\] and \[\frac{5\times 3}{\,8\times 3}\,=\frac{15}{24}\]
Clearly,
15 > 14
\[\therefore \,\,\,\frac{15}{24}>\frac{14}{24}\]
\[\Rightarrow \,\,\,\frac{5}{8}\,>\frac{7}{12}\]
EQUIVALENT RATIOS
A ratio obtained by multiplying or dividing the numerator and denominator by the same number is called an equivalent ratio.
For example:
Consider the ratio 5 : 7
We have,
\[\frac{5}{7}=\frac{5\times 2}{7\times 2}\,=\frac{10}{14}\]
\[\frac{5}{7}\,=\frac{5\times 3}{7\times 3}\,=\frac{15}{21}\] and so on.
also, \[\frac{10}{14}=\frac{10\div 2}{14\div 2}=\frac{5}{7}\]
\[\frac{10}{14},\,\frac{15}{21},\,\frac{20}{28}\] etc. are equivalent to the ratio \[\frac{5}{7}\]
PROPORTION
PROPORTION
Proportion is defined as an equality of two ratios,
Four (non-zero) quantities of the same kind a, b, c and d are said to be in proportion if the ratio of a to b is equal to the ratio of c to d
i.e., if \[\frac{a}{b}=\frac{c}{d}\]
we can write as a : b : : c : d
a, b, c, d are in proportion if ad = be
The (non-zero) quantities of the same kind a, b, c, d, e, f,... are said to be in continued proportion.
\[\frac{a}{b}=\frac{b}{c}\,=\frac{c}{d}=\frac{d}{e}=\]
If a, b, c are in continued proportion, then b is called mean proportional of a and c.
If a, b, c are in continued proportion then c is called the third proportional.
For example: Check if 3,4, 6 and 12 are in proportion we have, a = 3, b = 4, c = 6 and d = 12
\[\frac{a}{b}=\frac{3}{4}\] and \[\frac{c}{d}=\frac{6}{12}\,=\frac{1}{2}\]
Clearly, \[\frac{a}{b}\ne \frac{c}{d}\]
\[\therefore \,\,\,\,\,\,3:4\ne 6:12\]
Hence, 3, 4, 6 and 12 are not in proportion.
UNITARY METHOD
The method in which first we find the value of one unit and then the value of required number of units by multiplying the value of one unit with the number of required units.
For example:
A car travels 240 km in 4 hours.
How for does it travel in 7 hours?
Solution
We have,
Distance travelled in 4 hours = 240 km
\Distance travelled in 1 hour \[=\left( \frac{240}{4} \right)\] km = 60 km
Hence, the distance travelled in 7 hours = (60 x 7) = 420 km
For example:
The cost of four dozens of mangoes is Rs 150. What will be the cost of such 12 dozens of mangoes?
Solution: We know
cost of 1 dozen mangoes \[=\frac{150}{4}\]
cost of 12 dozen of mangoes \[=\frac{150}{\bcancel{4}}\times {{\bcancel{12}}^{3}}\]
= Rs450
You need to login to perform this action.
You will be redirected in
3 sec
