Fractions and Decimals
Category : 6th Class
FRACTIONS AND DECIMALS
FUNDAMENTALS
Natural numbers: All counting numbers are called natural numbers.
Whole number: Natural numbers together with zero are called whole numbers.
or
A number written in the form \[\frac{x}{y},\] where \[x\] and \[y\] are whole numbers and \[y\ne 0\] is called fraction,
Types of Fraction
Example: \[\frac{1}{10},\frac{2}{100},\frac{5}{1000}\]etc...
Example: \[\frac{1}{2},\frac{3}{4},\frac{7}{9}\]etc...
Example: \[\frac{7}{2},\frac{9}{4},\frac{11}{10}\] etc...
Example: \[1\frac{1}{2},7\frac{3}{4},2\frac{1}{2}\]etc...
Example: \[\frac{1}{2}\] and \[\frac{2}{4},\,\,\frac{1}{4}\,\,\text{and}\]\[\frac{4}{16},\,\,\frac{10}{12}\,\,\text{and}\]\[\frac{5}{6}\]
Example: \[\frac{4}{5}\]and \[\frac{2}{5},\,\,\frac{2}{7},\,\,\frac{3}{5},\,\,\frac{5}{7}\]etc...
Companion of fractions
(i) If\[x\times w>y\times z\], then \[\frac{x}{y}>\frac{z}{w}\]
(ii) If\[x\times w<y\times z\], then \[\frac{x}{y}<\frac{z}{w}\]
(iii) If\[x\times w=y\times z\], then\[\frac{x}{y}=\frac{z}{w}\]
Example: Compare the \[\frac{2}{3}\] and \[\frac{5}{6}\]
Solution: On cross multiplication we get, \[2\times 6=12\]and \[3\times 5=15\]
\[12<15\]
\[\therefore \] \[\frac{2}{3}<\frac{5}{6}\]
Example: Arrange \[\frac{2}{5},\,\,\frac{1}{4},\,\,\frac{3}{2},\,\,\frac{9}{10}\]in ascending order.
Solution: The LCM of \[5,\,\,4,\,\,2,\,\,10=20\]
\[\frac{2}{5}=\frac{2\times 4}{5\times 4}=\frac{8}{20},\frac{1}{4}=\frac{1\times 5}{4\times 5}=\frac{5}{20},\frac{3}{2}=\frac{3\times 10}{2\times 10}=\frac{30}{20}.,\]
\[\frac{9}{10}=\frac{9\times 2}{10\times 2}=\frac{18}{20}\]
Now, compare the numerators of like fractions \[\frac{8}{20},\frac{5}{20},\frac{30}{20},\frac{18}{20}\]
Arrange them in ascending order, we get \[\frac{5}{20}<\frac{8}{20}<\frac{18}{20}<\frac{30}{20}\]
Hence, \[\frac{1}{4}<\frac{2}{5}<\frac{9}{10}<\frac{3}{2}\]
Finding fraction between two given fraction
Example: Find a fraction lying between \[\frac{2}{3}\] and\[\frac{5}{7}\]
Solution: We have \[\frac{2}{3}\]and \[\frac{5}{7}\]
Fraction lying between \[\frac{2}{3}\] and \[\frac{5}{7}\] is \[\frac{2+5}{3+7}=\frac{7}{10}\]
So, we have \[\frac{2}{3},\frac{7}{10},\frac{5}{7}.\]
Fundamental operations on fraction
Example: Add\[\frac{3}{7}+\frac{5}{7}=\frac{3+5}{7}=\frac{8}{7}\]
Note: While adding unlike terms, first convert them into like fractions and then add as like fractions.
Properties of Addition of fraction
(i) Closure property: If \[\frac{x}{y}\] and \[\frac{z}{w}\] are two fractions, then \[\frac{xw+zy}{yw}\] is also a fractions
(ii) Commutative property: If \[\frac{x}{y}\] and \[\frac{z}{w}\] are two fractions, them\[\frac{x}{y}+\frac{z}{w}=\frac{z}{w}+\frac{x}{y}\]
(iii) Associative property: If \[\frac{r}{s},\]\[\frac{t}{u}\] and \[\frac{v}{w}\] are three fractions, then \[\frac{r}{s}+\left( \frac{t}{u}+\frac{v}{w} \right)=\left( \frac{r}{s}+\frac{t}{u} \right)+\frac{v}{w}\] Subtraction \[\frac{x}{y}-\frac{z}{y}=\frac{x-z}{y}\]
Example: \[\frac{4}{5}-\frac{3}{5}=\frac{4-3}{5}=\frac{1}{5}\]
Note: While subtracting unlike fraction, first convert them into like fractions and find difference as in like fractions.
Multiplication
Example: Multiply \[\frac{3}{7}\] and \[\frac{2}{5}\]
Solution: \[\frac{3}{7}\times \frac{2}{5}=\frac{3\times 2}{7\times 5}=\frac{6}{35}\]
Properties of multiplication of fractions
\[\frac{r}{s}\times \left( \frac{t}{u}\times \frac{v}{w} \right)=\left( \frac{r}{s}\times \frac{t}{u} \right)\times \frac{v}{w}\]
Example: Find the reciprocal of\[\frac{5}{7}\].
Solution: The reciprocal of \[\frac{5}{7}\] is\[\frac{7}{5}\].
Example: Divide\[\frac{5}{9}\div \frac{25}{3}\]
Solution: \[\frac{5}{9}\div \frac{25}{3}=\frac{5}{9}\times \frac{3}{25}=\frac{1}{15}\]
Example: \[0.6,\,\,1.76,\,\,5.046\]
Look at this Example
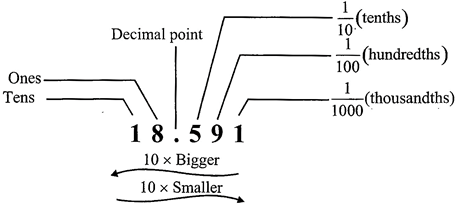
Example: Study the expanded form of the given decimal numbers:
|
Decimal Number |
Tens |
Units |
Tenths |
Hundredths |
Thousandths |
|
\[35.6\] |
3 tens |
5 units |
6 tenths |
|
|
|
\[25.58\] |
2 tens |
5 units |
5 tenths |
8 hundredths |
|
|
\[17.415\] |
1 ten |
7 units |
4 tenths |
1 hundredths |
5 thousandths |
|
\[78.004\] |
7 tens |
8 units |
0 tenths |
0 hundredths |
4 thousandths |
|
\[06.07\] |
0 tens |
6 units |
0 tenths |
7 hundredths |
|
Types of Decimals
Example: (i) \[0.7,\,\,1.1,\,\,25.6,\,\,238.4~\] (ii) \[0.21,\,\,666.26,\,\,6.57\]
Example: (i) \[0.7,\,\,0.21,\,\,6.323\] (ii) \[5.17,\,\,9.2,\,\,16.276\]
Converting unlike decimals into like decimals
Example: To convert \[2.5,\,\,8.03\]and \[7.352\] into like decimals.
Solution: We have to convert \[2.5\]and \[8.03\] into equivalent decimals with three decimal places i.e., \[2.5=2.500,\,\,8.03=8.030,\]
Now\[2.500,\,\,8.030,\,\,7.352\]like decimals.
Operations on Decimals
1. Addition of Decimals:
Example: (i) Add \[6.3\]and \[5.75\]
![]() Convert to like decimals
Convert to like decimals ![]()
(ii) Add \[6.5,\,\,7.05\] and \[5.325\]
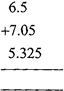 Convert to like decimals
Convert to like decimals 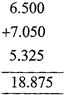
2. Subtraction of Decimals:
Example: Subtract \[56.128\]from \[68.75\]
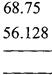 convert to like decimals
convert to like decimals 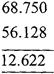
3. Multiplication of decimals:
In multiplying the decimals,
(a) Multiply as with whole numbers ignoring the decimals.
(b) Count the number of decimal places in factors.
(c) Show the number of decimal places in the product as many as there are in the factors.
(d) While counting the digits in the product to place the decimal point, start from the right.
Step-1:
Example:
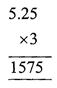
Step-2:
Total number of decimal places in the factors are 2
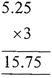
Care - 1: Some time you need to write extra zeros in the product on the left side to be able to show the correct number of decimal places.
Example: Multiply \[0.2\]by \[0.2\]
![]()
Here, number of decimal places are 2

Extra zero is required to show two decimal places.
Case - 2: Multiplication by 10,100 and 1000.
Example: \[10\times 0.389=03.89\]
Example:\[100\times 0.785=78.5\]
Example: \[1000\times 1.3\text{ }95=1395\]
DIVISION OF DECIMAL
Case - 1: Dividing by whole number
Example:
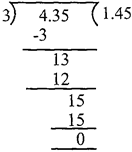
Hence \[4.35-3=1.45\]
Case - 2: Division by 10, 100, 1000
1. On division by 10 the decimal point moves one decimal place to the left.
Example: (i) \[4-10=0.4\]
(ii) \[321.4-10=32.14\]
2. On division by 100 the decimal point moves two decimal places to the left.
Example: (i) \[321.5-100=3.215\]
(ii) \[244-100=2.44\]
3. On division by 1000 the decimal point moves three places to the left.
Example: (i) \[321.6-1000=0.3216\]
(ii) \[3.25-1000=0.0325\]
Note: You need to place extra zeros wherever necessary.
Case - 3: Division by a decimal fraction
When we divide a decimal fraction by another decimal fraction we have to first change the divisor into a whole number.\[0.76\div 0.4=?\]
Step - 1: Make the decimal places of the dividend and divisor equal that is \[\frac{0.76}{0.4}=\frac{0.76}{0.40}\]
Step - 2: Now remove decimal point as the decimal places are equal. \[\frac{0.76}{0.4}=\frac{0.76}{0.40}=\frac{76}{40}\]
Step - 3: Now divide 76 by 40 as usual and write the answer.
\[\therefore \]\[0.76\div 0.4=1.9\]
Note: To convert the decimal divisor into a whole number multiply it with either 10 or 100 or 1000 and so on according to the decimal places.
You need to login to perform this action.
You will be redirected in
3 sec
