Geometry
Category : 5th Class
GEOMETRY
FUNDAMENTALS
Example: If you hold a thread taut between two hands, it represents part of a line.
![]()
Example: A piece of paper represents a plane, Top of a table represents plane, etc.
Passing through a point, an infinite number of lines can be drawn.
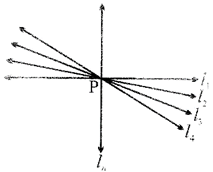
\[{{l}_{1,}}{{l}_{2.................}}{{l}_{n}}\] All pass through ?P?
These lines are also called CONCURRENT lines and the point P is called point of concurrence.
Collinearity of Points
Three points A, B, C in a place are collinear if they lie on the same straight line.
![]()
If this equality holds, then points are collinear.
If this equality doesn?t hold, then points are non-collinear.
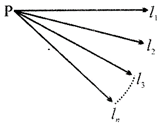
\[{{l}_{1,}}{{l}_{2,}}{{l}_{3..........}}{{l}_{n}}\]are all rays.
Line Segment
![]()
Example: if \[\overline{AB}\]=10cm and \[\overline{CD}=4\]inch then \[\overline{AB}=\overline{CD}\](because 1 inch=2.5 cm)
ANGLE
Example:
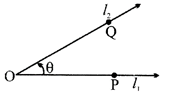
Rays \[{{l}_{1}}\] and \[{{l}_{2}}\] form an angle between them; this angle is represented as \[\theta =\angle POQ.\angle POQ\] can simply be written as\[\angle O\].
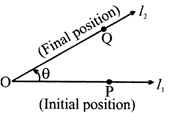
Angles in a triangle
A plane figure bounded by three line segment is called a triangle.
Example:
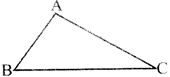
It has three angles \[\angle BAC\] (also called\[\angle A\]), \[\angle ABC\] (also called\[\angle B\]) and\[\angle ~ACB\] (also called\[\angle C\]).
In a\[\Delta \], if one angle \[={{90}^{o}}\]then it is called right angled triangle.
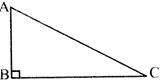
ABC is a right \[\Delta \] in which \[\angle B={{90}^{o}}\]
In a right-angle\[\Delta \], side opposite to right \[\angle \]is called hypotenuse. Other two sides are called base and perpendicular. The relation between these sides is given by Pythagoras as
\[{{\text{(Base)}}^{\text{2}}}\text{+(Perpendicular}{{\text{)}}^{\text{2}}}\text{=(Hypotenuse}{{\text{)}}^{\text{2}}}\]\[A{{B}^{2}}+B{{C}^{2}}=A{{C}^{2}}\] (in right \[\Delta ABC\])
(i) Isosceles \[\Delta \](a \[\Delta \] in which two sides are equal)
(ii) Equilateral \[\Delta \](a \[\Delta \] in which all three sides are equal)
(iii) Scalene triangle \[\Delta \] (a \[\Delta \] in which no side is equal)
If in \[\Delta \] ABC, AB=AC, then it is isosceles, also \[\angle \]B=\[\angle \]C
If 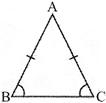 in equilateral\[\Delta ABC\],
in equilateral\[\Delta ABC\],
\[AB=BC=CA\], also\[\angle A=\angle B=\angle C={{60}^{o}}\]
QUADRILATERALS
Type of Quadrilaterals are as follows:
(i) Trapezium: A quadrilateral having only one pair of parallel sides
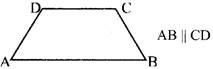
(ii) Isosceles trapezium: It is a special type of trapezium in which non-parallel sides are equal i. e. \[AB||CD\] and\[AD=BC\]
(iii) Parallelogram: A quadrilateral having both pairs of opposite sides are parallel i. e.,\[AB||CD\]and\[AD||BC\]
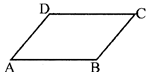
As a natural consequence of this, AB = CD and AD = BC, and \[\angle A=\angle C\]and\[\angle B=\angle D\]
(iv) Rhombus: It is a special type of parallelogram in which all side are equal
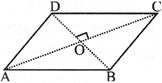
\[AB||CD\]
\[AD||BC\]
\[AB=BC=CD=DA\]
Also, \[\text{ A}C\bot BD\](i.e. diagonals are perpendicular to each other)
And,\[AO=CO\] and \[BO=DO\] (i.e., diagonals bisect each other)
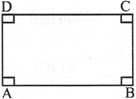
\[AB||CD;\,\,AD||BC\]
\[AB=CD;\,\,AD=BC\]
\[\angle A=\angle B=\angle C=\angle D={{90}^{o}}\]
\[AB||CD;\,\,AD||BC\]
\[AB=BC=CD=DA\]
\[\angle A=\angle B=\angle C=\angle D={{90}^{o}}\]

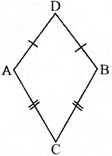
Adjacent Sides: \[AB=BC\] and\[AD=CD\]
Opposite sides: \[AB\ne CD\]; \[BC\ne AD\]
CIRCLE
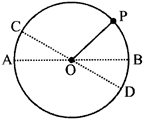
'O' is the fixed point called center.
'P' is movable point.
OP is called radius of a circle
\[\therefore \] AB or CD are diameters.
Since infinite line segments can be drawn through O, therefore, numbers of diameters are infinite.
Now, look at the figure below:
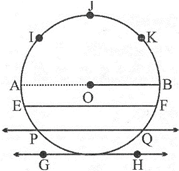
AB = diameter
You need to login to perform this action.
You will be redirected in
3 sec
