Trigonometry
Category : 10th Class
TRIGONOMETRY
Systems of Measurement of an Angle
Circular System
In this system, the angle is measured in radians.
Radian: The angle subtended by an arc length APB equal to the radius of a circle at its centre is defined of one radian (see figure). It is written as \[{{1}^{c}}\]. (‘c’ denotes radian)
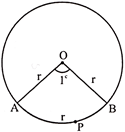
Relation between the Units
Look at the circle in the above figure and note that,
\[360{}^\circ =2{{\pi }^{c}}\Rightarrow 90{}^\circ =\frac{{{\pi }^{c}}}{2}\] and \[45{}^\circ =\frac{{{\pi }^{c}}}{4}\]; Or, simply, \[90{}^\circ =\frac{\pi }{2},45{}^\circ =\frac{\pi }{4}\]
For convenience, the above relation can be written as, \[\frac{D}{90}=\frac{R}{\frac{\pi }{2}}\], where, D denotes degrees, and R radians.
Remember
Note: If no unit of measurement is shown for any angle, it is considered as radian.
Trigonometric Ratios
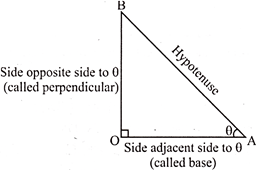
Let AOB be a right triangle with \[\angle AOB\] as \[90{}^\circ \]. Let \[\angle OAB\]be \[\theta \]. Notice that \[0{}^\circ <9<90{}^\circ \]. That is, \[\theta \] is an acute angle (see adjacent figure).
Six possible ratios among the three sides of the triangle AOB, are possible. They are called trigonometric ratios.
We observe that,
PYTHAGOREAN TRIPLETS
Pythagorean Triplets are basically sides of a right A which obey Pythagoras theorem.
Examples are:
Example: If \[\cos \theta =\frac{2}{5}\], then find the values of \[\tan \,\theta ,\cos ec\,\theta .\]
Solution:
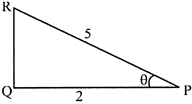
Given, \[\cos .\theta =\frac{2}{5}\]
Let PQR be the right triangle such that \[\angle QPR=\theta \] (see figure)
Assume that \[PQ=2\] and \[PR=5\].
Then, \[QR=\sqrt{P{{R}^{2}}-P{{Q}^{2}}}=\sqrt{25-4}=\sqrt{21}\]
So, \[\tan \,\theta =\frac{Opposite\,\,side\,\,to\,\,\angle \theta }{base}=\frac{QR}{PQ}=\frac{\sqrt{21}}{2}\] and \[\cos ec\,\theta =\frac{Hypotenuse}{perpendicular}=\frac{PR}{QR}=\frac{5}{\sqrt{21}}\]
Trigonometric Identities
Value of Trigonometric Ratios for Specific Angles
|
Trigonometric Ratios |
Angles |
||||
|
\[0{}^\circ \] |
\[30{}^\circ =\frac{\pi }{6}\] |
\[45{}^\circ =\frac{\pi }{6}\] |
\[60{}^\circ =\frac{\pi }{3}\] |
\[90{}^\circ =\frac{\pi }{2}\] |
|
|
\[\sin \theta \] |
\[0\] |
\[\frac{1}{2}\] |
\[\frac{1}{\sqrt{2}}\] |
\[\frac{\sqrt{3}}{2}\] |
\[1\] |
|
\[\cos \theta \] |
\[1\] |
\[\frac{\sqrt{3}}{2}\] |
\[\frac{1}{\sqrt{2}}\] |
\[\frac{1}{2}\] |
\[0\] |
|
\[\tan \theta \] |
\[0\] |
\[\frac{1}{\sqrt{3}}\] |
\[1\] |
\[\sqrt{3}\] |
\[\infty \] |
|
\[\cos ec\theta \] |
\[\infty \] |
\[2\] |
\[\sqrt{2}\] |
\[\frac{2}{\sqrt{3}}\] |
\[1\] |
|
\[\sec \theta \] |
\[1\] |
\[\frac{2}{\sqrt{3}}\] |
\[\sqrt{2}\] |
\[2\] |
\[\infty \] |
|
\[cot\theta \] |
\[\infty \] |
\[\sqrt{3}\] |
\[1\] |
\[\frac{1}{\sqrt{3}}\] |
\[0\] |
Note:
Graphically it is shown as,
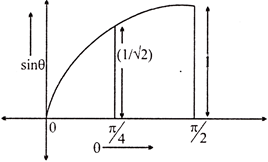
Graph of \[y=\sin \,\theta \] in \[\theta \,\in [0,\pi /2]\]
Graphically, it is shown as,
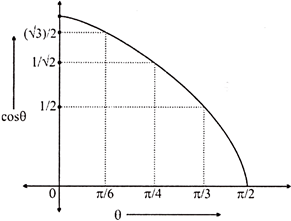
Graph of \[y=\cos \theta \] in \[\theta \in \left[ 0,\frac{\pi }{2} \right]\]
You need to login to perform this action.
You will be redirected in
3 sec
