10th Class Mathematics Introduction to Trigonometry Trigonometrical Ratios

       Introduction

As we know that the trigonometry is the branch of mathematics which study about the relationship between angles and its sides. All the trigonometrical ratios defined in the special type of a triangle i.e. Right angled triangle. In this chapter we will discuss about these ratios.  

       Trigonometrical Ratios

In the given right angle triangle ABC, in which right angle at B. Angle C is\[''\theta ''\] (suppose).

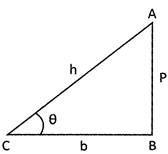
Then the trigonometrical ratios are defined as follows:

\[\sin \theta =\frac{\text{Perpendicual}}{\text{Hypotenuse}}=\frac{AB}{AC}\]

\[\cos \theta =\frac{Base}{\text{Hypotenuse}}=\frac{BC}{AC}\]

\[\tan \theta =\frac{\text{Perpendicular}}{Base}=\frac{AB}{BC}\]

\[\cot \theta =\frac{Base}{\text{Perpendicular}}=\frac{BC}{AB}\]

\[\sec \theta =\frac{\text{Hypotenuse}}{Base}=\frac{AC}{BC}\]  

\[co\sec \theta =\frac{\text{Hypotenuse}}{\text{Perpendicular}}=\frac{AC}{AB}\]  AB

If we represent perpendicular, base and hypotenuse by P, b and h respectively then the ratios can be written as:

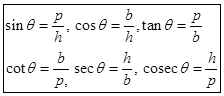
Relationship between Ratios

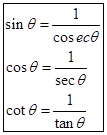
From the above, we conclude that sine of an angle is reciprocal to the cosec of that angle and so - on.