10th Class Mathematics Coordinate Geometry Distance Between two Points

      Distance Between two Points 

 

Let us consider the two points \[A({{x}_{1}}{{y}_{1}}),\And B({{x}_{2}},{{y}_{2}}),\] in a two dimensional plane, then the distance between the two points is given by \[AB=\sqrt{{{({{x}_{2}}-{{x}_{1}})}^{2}}+{{({{y}_{2}}-{{y}_{1}})}^{2}}}\]. If it is a three dimensional plane containing the points \[A({{x}_{1}},{{y}_{1}},{{z}_{1}})\And B({{x}_{2}},{{y}_{2}},{{z}_{2}},{{z}_{2}}),\] then the distance between the points is given by: \[AB=\sqrt{{{({{x}_{2}}-{{x}_{1}})}^{2}}+{{({{y}_{2}}-{{y}_{1}})}^{2}}+{{({{z}_{2}}-{{z}_{1}})}^{2}}}\]  

 

       Proof of Finding Distance Between Two Points

Let \[A({{x}_{1}},{{y}_{1}})\And B({{x}_{2}},{{y}_{2}})\] be the given points.

Draw AL \[\bot \] OX and AN \[\bot \] BM

                         

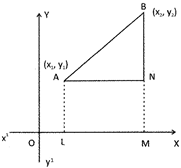
Now, from Figure 

\[OL={{X}_{1}},OM={{X}_{2}},AL={{Y}_{1}}\And BM={{Y}_{2}}\].

\[\therefore \,\,\,AN=LM=(OM-OL)={{X}_{2}}-{{X}_{1}}\]

BN = (BM - NM) = (BM - AL)  \[={{Y}_{2}}-{{Y}_{1}}\,\,[\therefore \,NM=AL]\]

From right \[\Delta ANB\], By Pythagorean" theorem, we have \[A{{B}^{2}}=(A{{N}^{2}}+B{{M}^{2}})\]

\[\Rightarrow \,\,{{(AB)}^{2}}={{({{x}_{2}}-{{x}_{1}})}^{2}}+{{({{Y}_{2}}-{{Y}_{1}})}^{2}}\]

\[\Rightarrow \,\,(AB)=\sqrt{{{({{x}_{2}}-{{x}_{1}})}^{2}}+{{({{Y}_{2}}-{{Y}_{1}})}^{2}}}\]

Hence, the distance between the points \[A({{x}_{1}},{{y}_{1}})\And B({{x}_{2}},{{y}_{2}})\] given by:

\[(AB)=\sqrt{{{({{x}_{2}}-{{x}_{1}})}^{2}}+{{({{Y}_{2}}-{{Y}_{1}})}^{2}}}\]    

 

Section Formula                                             

Let us consider the point P(X, Y) which divides the line segment joining \[A({{x}_{1}},{{x}_{1}})\And B({{x}_{2}}-{{y}_{2}})\] in the ratio m : n, then the coordinate of the point P is given by:                                                                         

\[X=\frac{m{{x}_{1}}n{{x}_{2}}}{m+n}and\,\,y=\frac{m{{y}_{1}}+n{{y}_{2}}}{m+n}\]  

 

Proof

Let \[A({{x}_{1}},{{y}_{1}})\And B({{x}_{2}},{{y}_{2}})\]be the two points and let P(X, Y) divides it in the ratio m:n, then,

\[\frac{AP}{PB}=\frac{m}{n}\]  

 

Draw \[AL\bot OX;BM\bot OX;PN\bot OX,AR\bot PN,\And PS\bot \]BM.                          

Now,        \[AR=LM=ON=OL=({{x}_{1}}-{{x}_{2}})\]

\[PS=NM=OM=ON=({{x}_{2}}-x)\]

\[PR=PN-RN=PN-AL=(y-{{y}_{1}})\]

\[BS=MB-SM=BM-PN=({{y}_{2}}-y)\]

Clearly \[\Delta ARP\,and\,\Delta PSB\] are similar & their sides are in proportional.             

\[\therefore \,\,\,\,\,\frac{AP}{PB}=\frac{AR}{PS}=\frac{PR}{BS}\]

\[\Rightarrow \,\,\,\,\,\frac{m}{n}=\frac{x-{{x}_{1}}}{{{x}_{2}}-x}=\frac{y-{{y}_{2}}}{{{y}_{2}}-y}\]

\[\Rightarrow \,\,\,\,x=\frac{m{{x}_{2}}+n{{x}_{1}}}{m+n}and\,y=\frac{m{{y}_{2}}+n{{y}_{1}}}{m+n}\]

Hence the coordinate of the point \[P\left( x=\frac{m{{x}_{2}}+n{{x}_{1}}}{m+n}and\,y=\frac{m{{y}_{2}}+n{{y}_{1}}}{m+n} \right)\]  

 

      Coordinate of Midpoint

The coordinate of the mid-point M of a line segment AB with coordinate \[A({{x}_{1}}-{{y}_{1}})\And B({{x}_{2}},{{y}_{2}})\] is given by \[\left( \frac{{{x}_{1}}+{{x}_{2}}}{2}\,and\,\,\frac{{{y}_{1}}+{{y}_{2}}}{2} \right)\]

 

Proof 

The mid-point of a line segment joining two points divide it into two equal parts, so the ratio is 1 : 1.

So, by the section formula, the coordinates of the \[\left( \frac{1.{{x}_{2}}+1.{{x}_{1}}}{1+1},\frac{1.{{y}_{2}}+{{y}_{2}}}{1+1} \right)\] i.e \[\left( \frac{{{x}_{1}}+{{x}_{2}}}{2},\frac{{{y}_{1}}+{{y}_{2}}}{2} \right)\]

Hence, the coordinates of the midpoint of AB are. \[\left( \frac{{{x}_{1}}+{{x}_{2}}}{2},\frac{{{y}_{1}}+{{y}_{2}}}{2} \right)\].

 

      Centroid of a Triangle

If is defined as the point of intersection of the medians of the triangle. To find the coordinates of the centroid of the triangles, we have, Let \[A({{x}_{1}},{{y}_{2}})B({{x}_{2}},{{y}_{2}})\And C({{x}_{3}},{{y}_{3}})\] be the vertices of a AABC. Let D be the midpoint of BC. Then, the coordinates of the point D is given by:

\[=\left( \frac{{{x}_{2}}+{{x}_{3}}}{2},\frac{{{y}_{2}}+{{y}_{3}}}{2} \right)\]

 

Let G (x, y) be the centroid of A ABC.

Then, G divides AD in the ratio 2 : 1.

\[\therefore \,\,\,\,x=\left\{ \frac{2\frac{({{x}_{2}}+{{x}_{3}})}{2}+1.{{x}_{1}}}{2+1} \right\}=\frac{({{x}_{1}}+{{x}_{2}}+{{x}_{3}})}{3}\]

\[y=\left\{ \frac{2\frac{({{y}_{2}}+{{y}_{3}})}{2}+1.{{y}_{3}}}{2+1} \right\}=\frac{({{y}_{1}}+{{y}_{2}}+{{y}_{3}})}{3}\]

Hence the coordinates of G are \[\left( \frac{({{x}_{1}}+{{x}_{2}}{{+}_{3}}}{3},\frac{({{y}_{1}}+{{y}_{2}}+{{y}_{3}})}{3} \right)\].  

 

      Area of the Triangle

Let \[A({{x}_{1}},{{y}_{1}})B({{x}_{3}},{{y}_{2}})\And C({{x}_{3}},{{y}_{3}})\]be the vertices of the triangle, then the area of the triangle is given by: Area \[=\frac{1}{2}\left| {{x}_{1}}({{y}_{2}}-{{y}_{3}})+{{x}_{2}}({{y}_{3}}-{{y}_{1}})+{{x}_{3}}({{y}_{1}}-{{y}_{2}}\left. ) \right| \right.\]

 

Proof

Let \[A({{x}_{1}},{{y}_{2}}),B({{x}_{2}},{{y}_{2}})\And C({{x}_{3}},{{y}_{3}})\] be the vertices of the given A ABC. Draw AL, BM & CN perpendicular to the x - axis.

Then, \[ML=({{x}_{1}}-{{x}_{2}}),Ln=({{x}_{3}}-{{x}_{1}})\And MN=({{x}_{3}}-{{x}_{2}})\]

 

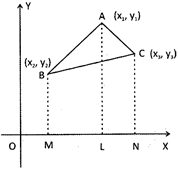
\[\begin{align}   & \therefore \,\,Area\,(\Delta ABC)=ar(trapBMLA)+ar(trapALNO) \\ & -ar(trapBMNC) \\ \end{align}\]

\[=\left\{ \frac{1}{2}(AL+BM)\times ML \right\}+\left\{ \frac{1}{2}(AL+CN)\times LN \right\}-\left\{ \frac{1}{2}(BM+CN)\times MN \right\}.\]

\[=\frac{1}{2}({{y}_{1}}+{{y}_{2}})\,({{x}_{1}}-{{x}_{2}})+\frac{1}{2}({{y}_{1}}+{{y}_{3}})\,({{x}_{3}}-{{x}_{1}})-\frac{1}{2}({{y}_{2}}+{{y}_{3}})\,({{x}_{3}}-{{x}_{2}}).\]

Ar. \[(\Delta ABC)=\frac{1}{2}\left| \left. {{x}_{1}}({{y}_{2}}-{{y}_{3}}+{{x}_{2}}({{y}_{3}}-{{y}_{1}})+{{x}_{3}}({{y}_{1}}-{{y}_{2)}}) \right|. \right.\]  

 

        Condition for Collinearity of Three Points

Let the given points be A \[A({{x}_{1}},{{y}_{2}})B,({{x}_{2}},{{y}_{2}})\And C({{x}_{3}},{{y}_{3}})\] .If A, B & C are collinear then. Area (\[\Delta \] ABC) = 0.

\[\Rightarrow \,\,\,\,\frac{1}{2}\left| \left. {{x}_{1}}({{y}_{2}}-{{y}_{3}})+{{x}_{2}}({{y}_{3}}-{{y}_{2}}) \right|=0 \right.\] 

 

       Coordinate of In center

Lf \[A({{x}_{1}},{{y}_{2}}),B({{x}_{2}},{{y}_{2}})\And C({{x}_{3}},{{y}_{3}})\] be the coordinate of the vertices of the A (ABC), having side length' a, b, c,' then the incentre of the triangle is given by:

\[I=\left( \frac{a{{x}_{1}}+b{{x}_{2}}+c{{x}_{3}}}{a+b+c},\frac{a{{y}_{1}}+b{{y}_{2}}+c{{y}_{3}}}{a+b+c} \right)\]  

 

 

 

Find the coordinates of the point which divides the line segment Joining the points A(4 - 3) & B(9,7) in the ratio 3 : 2.

(a) (7, 3)                                                 

(b) (5, 3)

(c) (4, 3)                                                  

(d) (2, 3)

(e) None of these  

 

Answer: (a)

Explanation

The coordinate of the points are A(4-3) &B(9,7)

Also m : n = 3 : 2

Let the coordinate of the point be P(x, y)

Then by section formula, we have             

\[x=\frac{m{{X}_{1}}+n{{X}_{2}}}{m+n}and\,y=\frac{m{{Y}_{1}}+n{{Y}_{2}}}{m+n}\]             

\[x=\frac{(3\times 9+2\times 4)}{(3+2)},y=\frac{\{3\times 7+2(-3)\}}{(3+2)}\]                    

\[x=7,y=3\].

Hence the required point is P (7, 3)  

 

 

  Find the distance between the points A (7, 13) & B (10, 9).

(a) 2 units                                              

(b) 3 units

(c) 5 units                                               

(d) 7 units                              

(e) None of these  

 

Answer: (c)

Explanation

The given points are A (7,13) & B(10, 9).

Here we have,             

\[({{x}_{1}}=7,{{y}_{1}}=13)\And ({{x}_{2}}=10,{{y}_{2}}=9)\]

Now the distance is given by:

\[AB=\sqrt{{{({{x}_{2}}-{{x}_{1}})}^{2}}+{{({{y}_{2}}-{{y}_{1}})}^{2}}}\]

\[=\sqrt{{{(10-7)}^{2}}+{{(9-13)}^{2}}}\]

\[=\sqrt{9+16}=\sqrt{25}=5\,unit\]  

 

 

  Find the coordinates of the mid-point of the line segment joining the points A(-5,4) & B(7,-8).

(a) (3, -2)                                                

(b) (1, -2)

(c) (1, 4)                                                  

(d) (3, 4)

(e) None of these  

 

Answer: (b)

Explanation

Let M (x, y) be the midpoint of AB.

Then  \[x=\frac{-5+7}{2}=1\,\,and\,\,y=\frac{4-8}{2}=-2\]     

Hence the mid- point is M (1, -2)  

 

 

  Find the area of the triangle whose vertices are A (2, 7), B (3,-l) & C(-5, 6).

(a) 18 sq. units                                     

(b) 24 sq. units

(c) 22 sq. units                                     

(d) 28 sq. units

(e) None of these  

 

Answer: (d)

Explanation

We have the coordinate of the vertices of the triangle\[\Delta ABC\,as\,A(2,7),B(3,-1)\And C(-5,\,6)\].

Then \[{{x}_{1}}=2,{{y}_{1}}=7,({{x}_{2}}=3,{{y}_{2}}=-1)\And ({{x}_{3}}=-5,{{x}_{3}}=6)\]

Area \[\Delta \]ABC \[=\frac{1}{2}\left| \left. {{x}_{1}}({{y}_{2}}-{{y}_{3}})+{{x}_{2}}({{y}_{3}}-{{y}_{1}})+{{x}_{3}}({{y}_{1}}-{{y}_{2}}) \right| \right.\]

\[=\frac{1}{2}\left| \left. 2(-1-6)+3(6-7)-5(7+1) \right| \right.\]

\[=\frac{1}{2}\left|\left. -14-12-30 \right|=\frac{56}{2}= \right.28\] Sq units  

 

 

  Find the value of 'K' for which the points A (2, 3) B (5, K) & C (6, 7) are collinear.

(a) 4                                                         

(b) 6                   

(c) \[\frac{-3}{2}\]                                              

(d) \[\frac{11}{4}\]

(e) None of these

 

Answer: (b)

Explanation

Since the points are collinear so area (\[\Delta \]ABC) = 0

\[\Rightarrow \,\,\,\frac{1}{2}\left| \left. 2(K-7)+5(7-3)+6(3-K) \right|=0 \right.\]

\[\Rightarrow \,\,\,4K-24=0\]

\[\Rightarrow \,\,\,4K=24\]

\[\Rightarrow \,\,\,K=6\]  

 

 

  Find the centroid of \[\Delta \] ABC whose vertices are A (-3, 0) B (5, -2) and C (- 8, 5).

(a) (-2, 1)                                                

(b) (2, 1)

(c) (3, 5)                                                  

(d) (3, 5)

(e) None of these                          

 

Answer: (a)

Explanation

We have, \[{{x}_{1}}=-3,{{y}_{1}}=0,{{x}_{2}}=5,{{y}_{2}}=-2\,and\,{{x}_{3}}=-8,{{y}_{3}}=5\]

Let G(x, y) be centroid of \[\Delta \] ABC, then \[x=\frac{1}{3}({{x}_{1}}+{{x}_{2}}+{{x}_{3}})\,and\,y=\frac{1}{3}({{y}_{1}}+{{y}_{2}}+{{y}_{3}})\]

\[\Rightarrow \,\,x=\frac{-3+5-8}{3}=-2\,and\ =\frac{0-2+5}{3}=1\]  

 

 

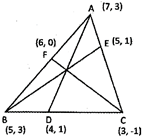
  Find the lengths of the medians AD of the A \[\Delta ABC\] whose vertices are A(7, 3), B(5, 3) and C(3, -1), where D is the midpoint of the side BC.  

 

(a) 5                                                         

(b) 6

(c) 10                                                       

(d) 9

(e) None of these  

 

Answer: (a)  

Explanation

Since D is the mid-point of the side BC of the triangle \[\Delta ABC\], then we have the coordinate of the point D is given by:  

\[D\left( \frac{5+3}{2}=4\frac{3+(-1)}{2}=1 \right)\]

i.e., D (4, 1)

Therefore the length of the medians AD is given by:

\[AD=\sqrt{{{(4-7)}^{2}}{{(1+3)}^{2}}}=\sqrt{{{(3)}^{2}}+{{4}^{2}}}=\sqrt{25}=5\]units  

 

 

  Let D (3, 2), E (-3, 1) & F (4 - 3) be the midpoints of the sides BC, CA & AB respectively of \[\Delta ABC\]. Find the coordinates of the vertex A of the \[\Delta ABC\].

(a) (10,-6)                                              

(b) (-2, 0)

(c) (-4,2)                                                 

(d) (5, -3)

None of these  

 

Answer: (b)

Explanation

Let the coordinate of the vertices of the triangle be given by \[A\left( {{x}_{1}},{{y}_{1}} \right)\], \[B({{x}_{2,}}{{y}_{2}})\And C({{x}_{3}},{{y}_{3}})\]

The coordinate of the midpoints of the sides BC, CA & AB of the triangle are given as D (3, 2), E (-3, 1) & F (4, 3) respectively

 

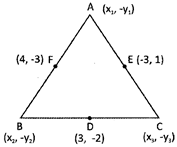
Since D is the mid-points of BC

Therefore, coordinate of D is given by:

\[\Rightarrow \,\,\frac{{{x}_{2}}+{{x}_{3}}}{2}=3,\frac{{{y}_{3}}+{{y}_{3}}}{2}=2\]

\[\Rightarrow \,\,{{x}_{2}}+{{x}_{3}}=6\,.................(i)\,and\,{{y}_{3}}=-4\,............(ii)\]

Again E in the mid-point of CA, therefore, coordinate of E is given by:

\[\Rightarrow \,\,\,\frac{{{x}_{1}}+{{x}_{3}}}{2}=-3\frac{{{y}_{1}}+{{y}_{3}}}{2}=-1\]

\[{{x}_{1}}+{{x}_{3}}=-6.......(iii),\,and\,{{y}_{1}}+{{y}_{3}}=2\,.......\,(iv)\]

And F is the mid-point of AB, therefore, coordinate of F is given by:

\[\Rightarrow \,\,\frac{{{x}_{1}}+{{x}_{2}}}{2}=4\frac{{{y}_{2}}+{{y}_{2}}}{2}=-3\]

\[\Rightarrow \,\,\,{{x}_{1}}+{{x}_{2}}=8\,........\,(v)\,\,and\,({{y}_{1}}+{{y}_{2}}=6........(iv)\]

Solving the above equations we get,

\[({{x}_{1}}=-2,{{x}_{2}}=10,{{x}_{3}}=4)\,and\,({{y}_{1}}=0,{{y}_{2}}=-6,{{y}_{3}}=2)\]

Therefore, coordinate of the vertex A (-2, 0)  

 

 

  If A (-2, -1), B (a, 0), C (4, b) & D (1, 2) are the vertices of a parallelogram, then find the values of a & b.

(a) a = 3, b = - 3                                    

(b) a = 3, b = 5

(c) a = 1, b = - 3                                    

(d) a = 1, b = 3

(e) None of these  

 

Answer: (d)

Explanation

We have the vertices of the parallelogram as A (-2, -1), B (a, 0), C (4, b) & D (1, 2).

Then AC & BD are the diagonals, intersecting each other at the point O Since we know that '0' is the midpoint of the diagonal AC and BD, as the diagonal of the parallelogram bisect each other,

Therefore, mid-point of \[AC=\left( \frac{-2+4}{2},\frac{-1+b}{2} \right)=\left( 1,\frac{b-1}{2} \right)\]

And the mid-point of \[BD=\left( \frac{a+1}{2},\frac{0+2}{2} \right)=\left( \frac{a+1}{2},1 \right)\]  

   

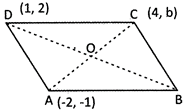
\[\therefore \,\,\,\,\,\,\frac{a+1}{2}=1\,\,\,\And \,\,\frac{b-1}{2}=1\]

\[\Rightarrow \,\,\,\,a=1\,\,\And \,\,b=3\]